Two wires of the same material and same length have radii 1 mm and 2 mm respectively. Compare :
their resistances,
To compare the resistances of two wires made of the same material and having the same length but different radii, we can follow these steps:
1. Understand the Formula for Resistance:
The resistance R of a wire is given by the formula:
R=ρLA
where:
- ρ is the resistivity of the material,
- L is the length of the wire,
- A is the cross-sectional area of the wire.
2. Identify Given Information:
- Both wires are made of the same material, so they have the same resistivity ρ.
- Both wires have the same length L.
- The radii of the wires are r1=1mm and r2=2mm.
3. Calculate the Cross-Sectional Area:
The cross-sectional area A of a wire with radius r is given by:
A=πr2
- For the first wire (radius r1=1mm):
A1=π(1mm)2=πmm2
- For the second wire (radius r2=2mm):
A2=π(2mm)2=4πmm2
4. Write the Resistance Equations:
- The resistance of the first wire R1:
R1=ρLA1=ρLπ
- The resistance of the second wire R2:
R2=ρLA2=ρL4π
5. Compare the Resistances:
To compare R1 and R2, we can find the ratio:
R1R2=ρLπρL4π=4ππ=4
Thus, the ratio of their resistances is:
R1:R2=4:1
Final Result:
The resistance of the first wire is four times that of the second wire. Therefore, the ratio of their resistances is 4:1.
The resistance R of a wire is given by the formula:
R=ρLA
where:
- ρ is the resistivity of the material,
- L is the length of the wire,
- A is the cross-sectional area of the wire.
- Both wires are made of the same material, so they have the same resistivity ρ.
- Both wires have the same length L.
- The radii of the wires are r1=1mm and r2=2mm.
The cross-sectional area A of a wire with radius r is given by:
A=πr2
- For the first wire (radius r1=1mm):
A1=π(1mm)2=πmm2
- For the second wire (radius r2=2mm):
A2=π(2mm)2=4πmm2
- The resistance of the first wire R1:
R1=ρLA1=ρLπ
- The resistance of the second wire R2:
R2=ρLA2=ρL4π
To compare R1 and R2, we can find the ratio:
R1R2=ρLπρL4π=4ππ=4
Thus, the ratio of their resistances is:
R1:R2=4:1
The resistance of the first wire is four times that of the second wire. Therefore, the ratio of their resistances is 4:1.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(B)
15 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(B) (MULTIPLE CHOICE TYPE)
3 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(A) ( MULTIPLE CHOICE TYPE)
2 Videos
- CALORIMETRYBOOK - ICSECHAPTER - CALORIMETRYEXERCISE - EXERCISE-11(B) (NUMERICALS)
15 Videos
- ELECTRO-MAGNETISM BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS
1 Videos
Similar Questions
Two wires of the same material and same length have radii 1 mm and 2 mm respectively. Compare :
their specific resistance.
View SolutionTwo wires of the same material have length 6cm and 10cm and radii 0.5 mm and 1.5 mm respectively. They are connected in series across a battery of 16 V. The p.d. across the shorter wire is
View Solution
Knowledge Check
Two copper wire of length l and 2l have radii, r and 2r respectively. What si the ratio of their specific resistance.?
A1:2B2:1C1:1D1:3
Two vibrating strings of the same material but lengths L and 2L have radii 2r and r respectively. They are stretched under the same tension. Both the string vibrate in their fundamental nodes, the one of length L with freuqency v1 and the other with frequency v2. the ratio v1/v2 is given by
View SolutionTwo wires of the same materical having equal area of cross-section have L and 2L. Their respective resistances are in the ratio
View SolutionTwo wires of the same material have lengths in the ratio 1:2 and their radii are in the ratio 1:√2 If they are stretched by applying equal forces, the increase in their lengths will be in the ratio
View SolutionTwo wires of the same material have lengths in the ratio 1:2 and their radii are in the ratio 1:√2 If they are stretched by applying equal forces, the increase in their lengths will be in the ratio
View SolutionThe wires A and B shown in Fig. are made of the same material and have radii rA and rB, respectively. The block between them has a mass m. When the force F is mg/3, one of the wires breaks. Then
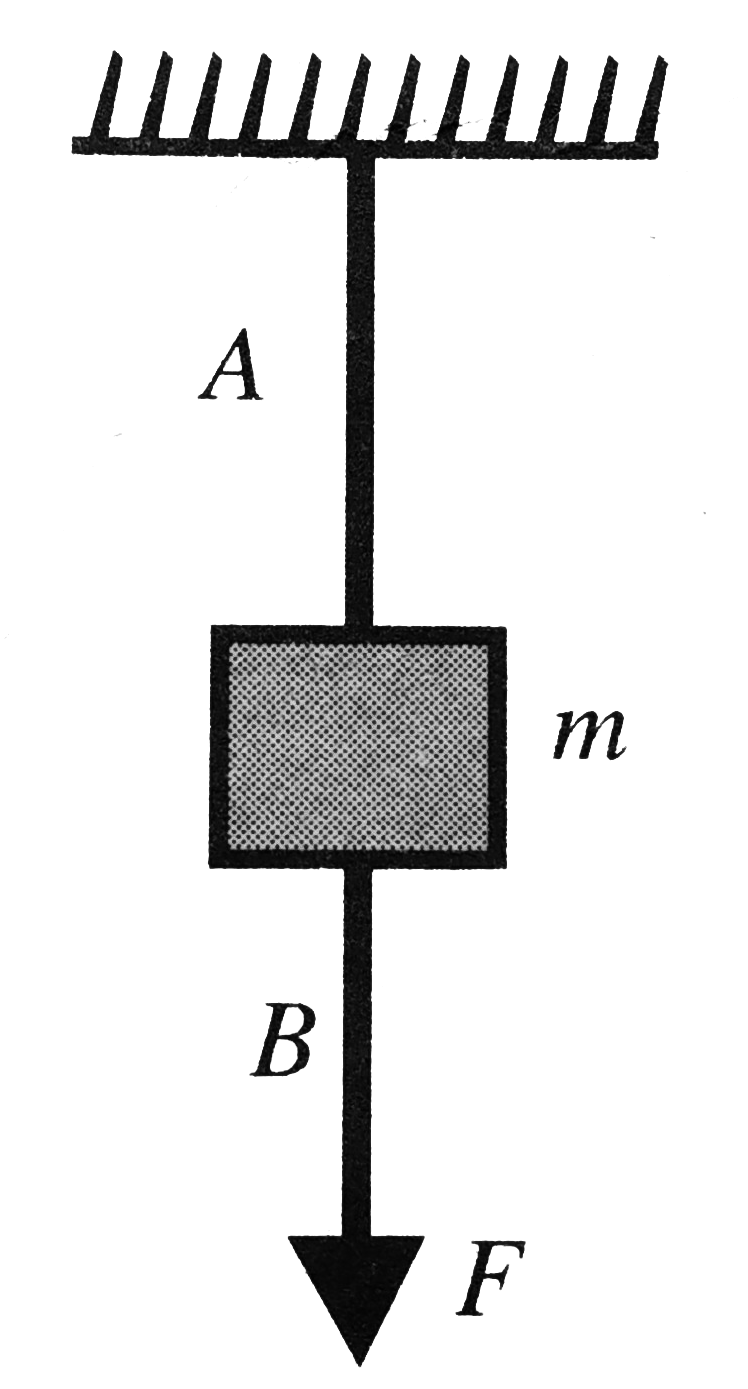
View SolutionThe wires A and B shown in Fig. are made of the same material and have radii rA and rB, respectively. The block between them has a mass m. When the force F is mg/3, one of the wires breaks. Then

View SolutionTwo wires of the same material and length are stretched by the same force. Their masses are in the ratio 3:2. Their elongations are in the ratio
View Solution
