Question

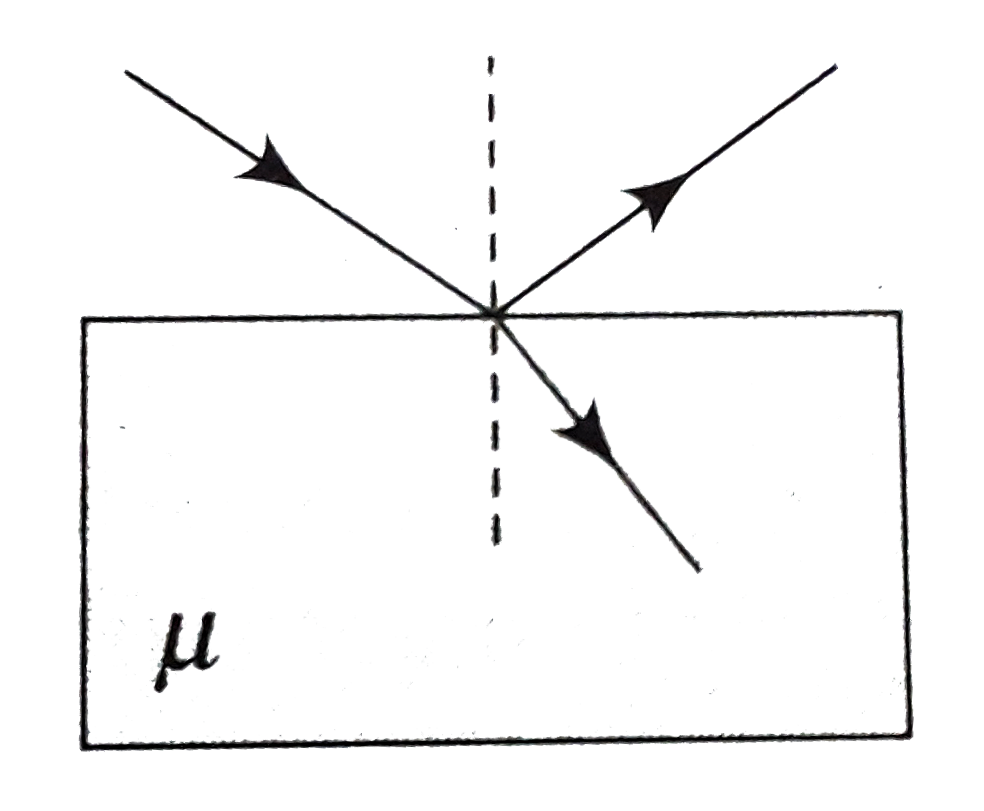
Unpolarized light is incident on a plane glass surface. What should be the angle of incidence so that the reflected and refracted rays are perpendicular to eachother ?

Explanation

To solve the problem of finding the angle of incidence so that the reflected and refracted rays are perpendicular to each other when unpolarized light is incident on a plane glass surface, follow these steps:

1. Understanding the Problem: We need to find the angle of incidence (denoted as \( I \)) at which the reflected ray and the refracted ray are perpendicular to each other. This means that the angle between these two rays is \( 90^\circ \).

2. Using the Condition for Perpendicular Rays: The condition for the reflected and refracted rays to be perpendicular is that the angle of incidence should be equal to the polarizing angle (\( I_p \)).

3. Refractive Index: We are given that the refractive index (\( \mu \)) of glass is \( 1.5 \).

4. Polarizing Angle Formula: The polarizing angle can be calculated using the formula:
\( \tan(I_p) = \mu \)
where \( \mu \) is the refractive index of the medium (glass in this case).

5. Substituting the Refractive Index: Substituting the value of the refractive index into the formula:
\( \tan(I_p) = 1.5 \)

6. Calculating the Polarizing Angle: To find \( I_p \), we take the arctangent (inverse tangent) of \( 1.5 \):
\( I_p = \tan^{-1}(1.5) \)

7. Finding the Value: Using a calculator or trigonometric tables, we find:
\( I_p \approx 56.3^\circ \)

8. Conclusion: Therefore, the angle of incidence \( I \) that makes the reflected and refracted rays perpendicular is:
\( I = 56.3^\circ \)

Final Answer:
The angle of incidence should be \( 56.3^\circ \).

---