Water enters through end A with a speed v1 and leaves through end B with a speed v2 of cylindrical tube AB. The tube is always completely filled with water. In case I the tube is horizontal, in case II it vertical with the end A upward and in case III it is vertical with the end B upward. We have v1=v2for
caseI
caseII
caseIII
each case
The correct Answer is:D
To solve the problem, we need to analyze the three cases of the cylindrical tube filled with water and determine under what conditions the speeds v1 and v2 are equal. We will use the principle of continuity in fluid mechanics, which states that for an incompressible fluid flowing through a pipe, the product of the cross-sectional area and the fluid velocity is constant along the pipe.
1. Understanding the Setup:
- We have a cylindrical tube with two ends: A (where water enters) and B (where water exits).
- The water enters with speed v1 at end A and exits with speed v2 at end B.
- The tube is completely filled with water.
2. Case I: Horizontal Tube:
- In this case, the tube is horizontal, and the height difference between A and B is zero.
- According to the continuity equation:
A1v1=A2v2
- Since the cross-sectional area A1 at A is equal to the cross-sectional area A2 at B (the tube is cylindrical and uniform), we can conclude:
v1=v2
3. Case II: Vertical Tube with A Upward:
- Here, the tube is vertical with end A at the top and end B at the bottom.
- The continuity equation still applies:
A1v1=A2v2
- Again, since A1=A2, we have:
v1=v2
- The gravitational potential energy does not affect the flow speed directly since the tube is completely filled with water.
4. Case III: Vertical Tube with B Upward:
- In this scenario, the tube is vertical with end B at the top and end A at the bottom.
- The continuity equation applies as well:
A1v1=A2v2
- Since A1=A2, we again find:
v1=v2
- The orientation does not change the fact that the tube is filled with water.
5. Conclusion:
- In all three cases (horizontal, vertical A up, vertical B up), the speeds v1 and v2 are equal due to the continuity equation and the uniform cross-sectional area of the tube.
Final Answer:
In all cases (I, II, and III), we have v1=v2.
- We have a cylindrical tube with two ends: A (where water enters) and B (where water exits).
- The water enters with speed v1 at end A and exits with speed v2 at end B.
- The tube is completely filled with water.
- In this case, the tube is horizontal, and the height difference between A and B is zero.
- According to the continuity equation:
A1v1=A2v2
- Since the cross-sectional area A1 at A is equal to the cross-sectional area A2 at B (the tube is cylindrical and uniform), we can conclude:
v1=v2
- Here, the tube is vertical with end A at the top and end B at the bottom.
- The continuity equation still applies:
A1v1=A2v2
- Again, since A1=A2, we have:
v1=v2
- The gravitational potential energy does not affect the flow speed directly since the tube is completely filled with water.
- In this scenario, the tube is vertical with end B at the top and end A at the bottom.
- The continuity equation applies as well:
A1v1=A2v2
- Since A1=A2, we again find:
v1=v2
- The orientation does not change the fact that the tube is filled with water.
- In all three cases (horizontal, vertical A up, vertical B up), the speeds v1 and v2 are equal due to the continuity equation and the uniform cross-sectional area of the tube.
In all cases (I, II, and III), we have v1=v2.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - Objective 2
8 Videos
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - Exercises
35 Videos
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - Short Answer
16 Videos
- CIRCULAR MOTIONBOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Exercises
30 Videos
- FRICTIONBOOK - HC VERMACHAPTER - FRICTIONEXERCISE - Exercises
31 Videos
Similar Questions
Water enters into a smooth horizontal tapering tube with a speed 2 m/sec and emerges out of the tube with a speed 8 m/sec . Find the ratio of the radii of cross sections of the tube at these two ends.
View SolutionA block of mass m is placed in contact with one end of a smooth tube of mass M. A horizontal force F acts on the tube in each case (i) and (ii). Then,
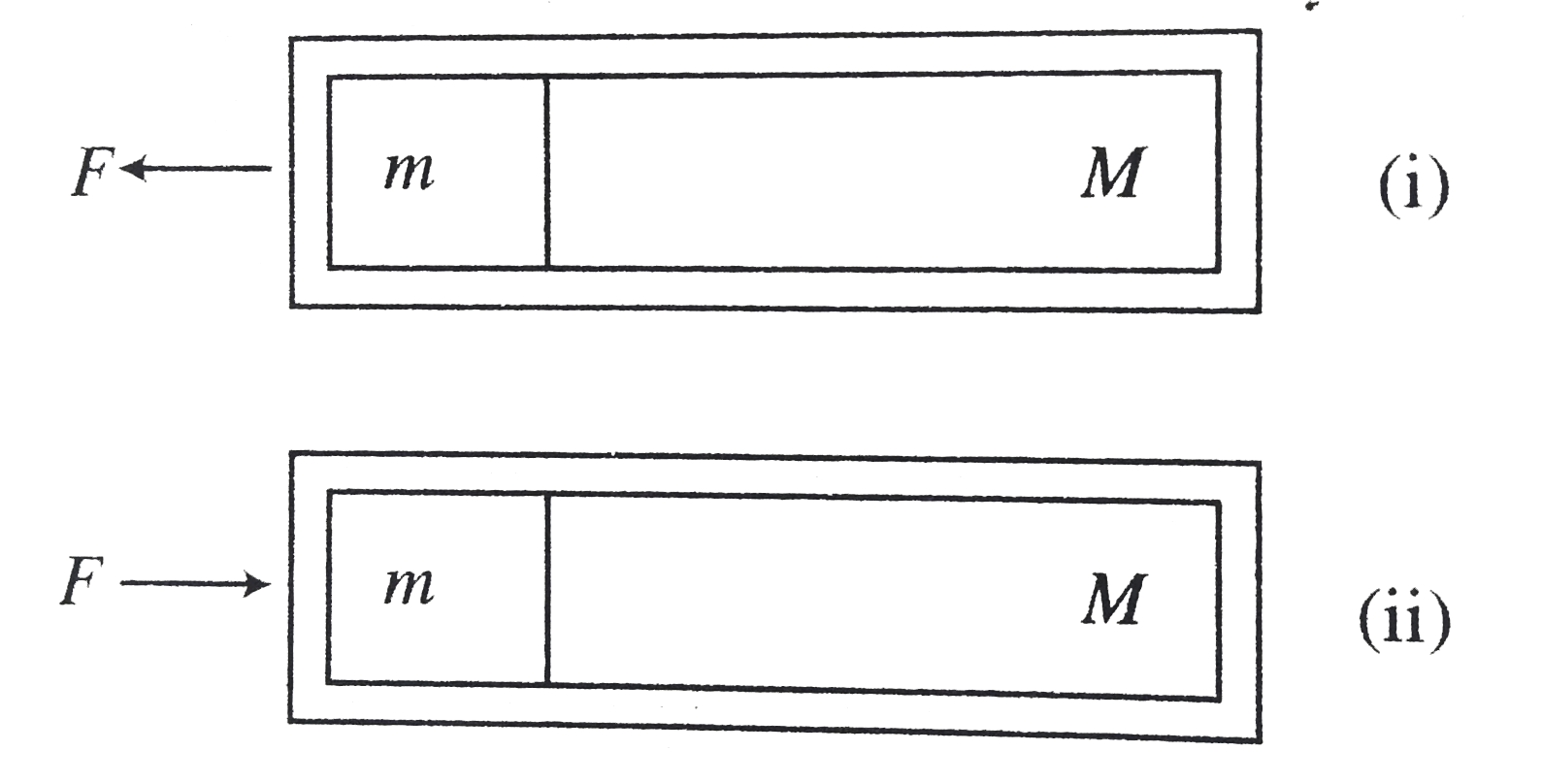
View Solution
Knowledge Check
Water enters through end A with a speed v1 and leaves through end B with a speed v2 of cylindrical tube AB. The tube is always completely filled with water. In case I the tube is horizontal, in case II it vertical with the end A upward and in case III it is vertical with the end B upward. We have v1=v2for
Acase IBcase IICcase IlIDeach caseWater is flowing through a tube of radius r with a speed v. If this tube is joined to another tube of radius r/2, what is the speed of water in the second tube?
Av/4Bv/2C2vD4vA cylindrical tube open at both the ends has a fundamental frequency of 390 Hz in air. If 14 th of the tube is immesed vertically in water the fundamental frequency of air column is
A260 HzB130 HzC390 HzD520 Hz
A cylindrical tube open at both ends, has a fundamental frequency F in air. The tube is dipped vertically in water so that half of it is in water. The fundamental frequency of air column is now
View SolutionA cylindrical tube open at both ends, has a fundamental frequency F in air. The tube is dipped vertically in water so that half of it is in water. The fundamental frequency of air column is now
View SolutionA cylindrical tube, open at the both ends, has a fundamental frequency f in air . The tube is dipped vertically in water so that half of it is in water . The fundamental frequency of the air column is now-
View SolutionA horizontal jet of water coming out of a pipe of area of cross-section 20cm2 hits a vertical wall with a velocity of 10ms−1 and rebounds with the same speed. The force exerted by water on the wall is .
View SolutionOne of the modes of resonance in a tube containing water at one end has been shown. The tube in the present case is in
View Solution
