We know that electric field (E ) at any point in space can be calculated using the relation

if we know the variation of potential (V) at that point. Now let the electric potential in volt along the x-axis vary as , where x is in meter. Its variation is as shown in figure
Draw the variation of electric field (E ) along the x-axis.
A
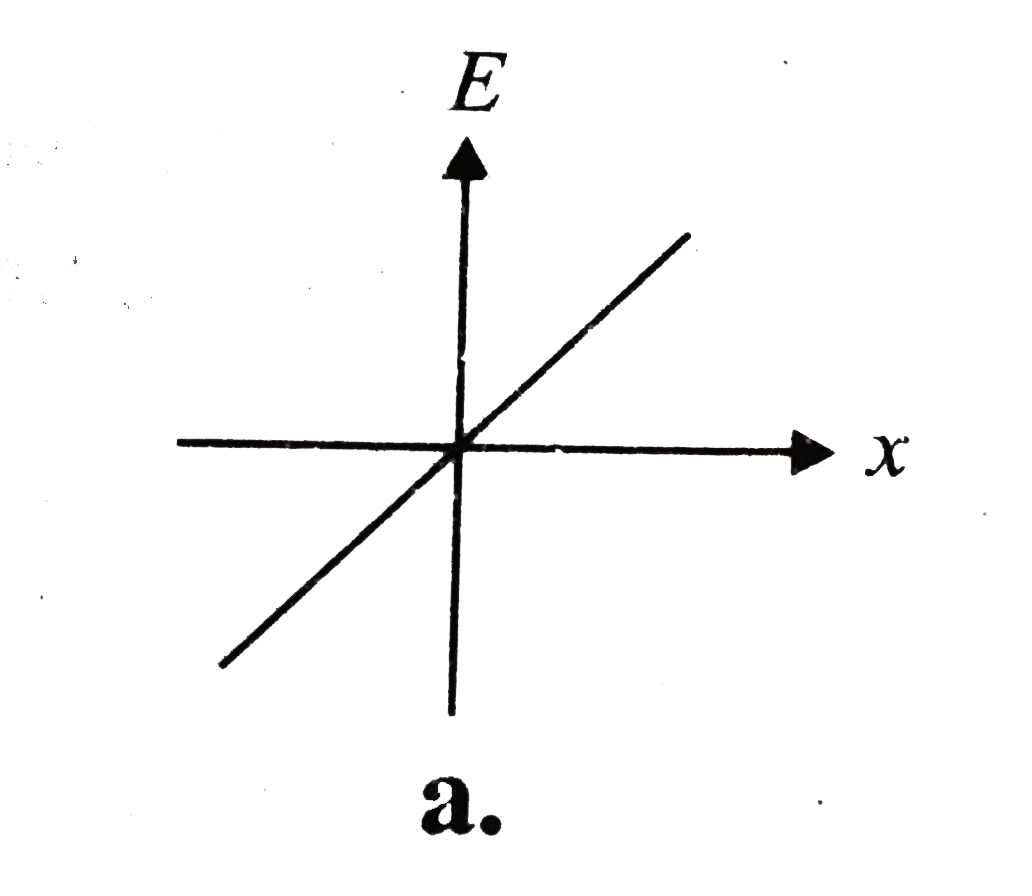
B
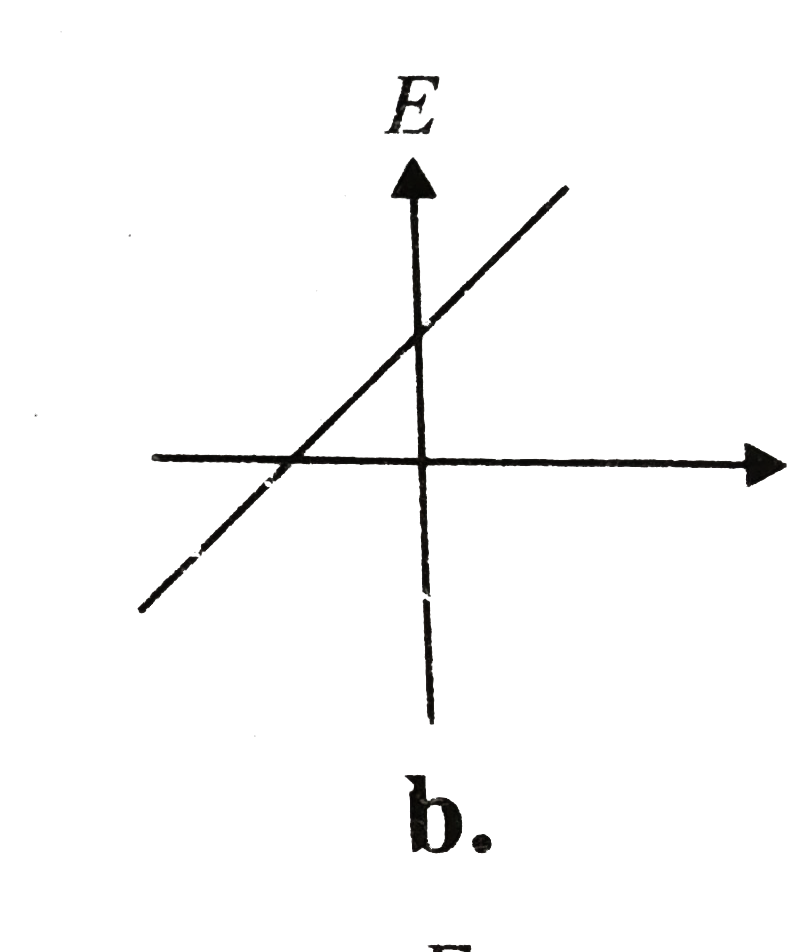
C
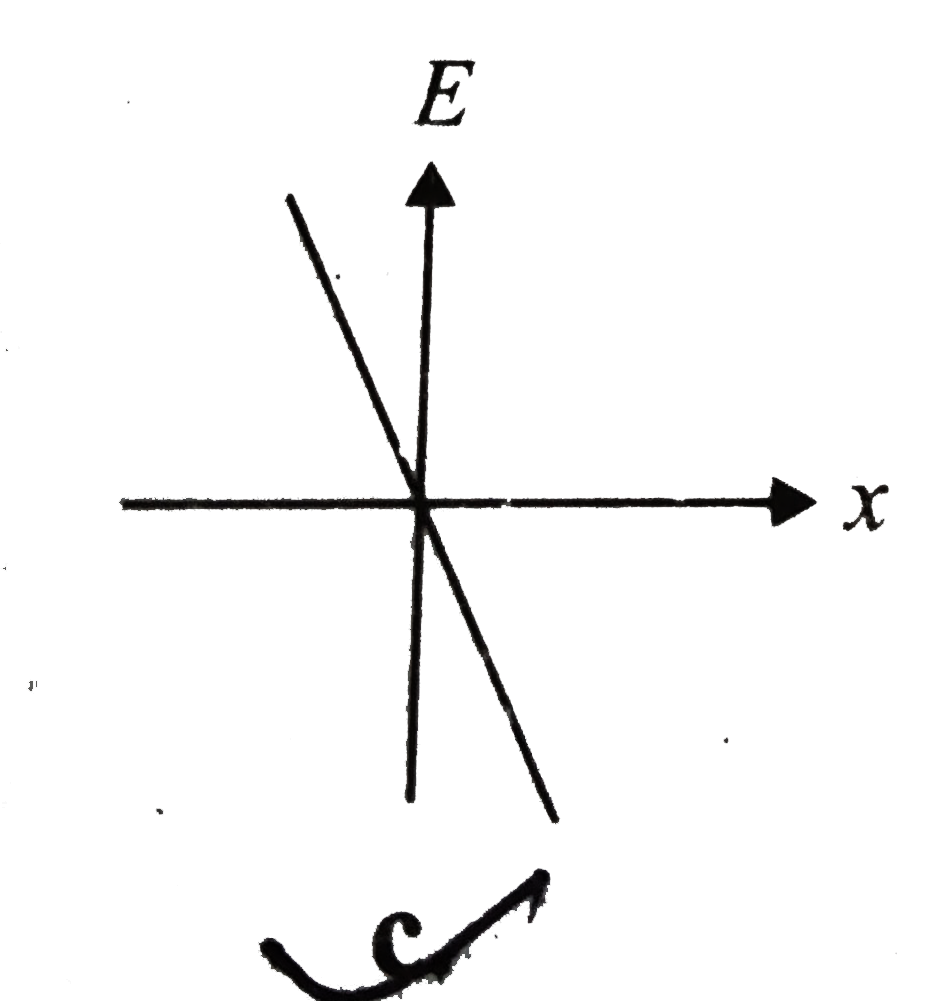
D
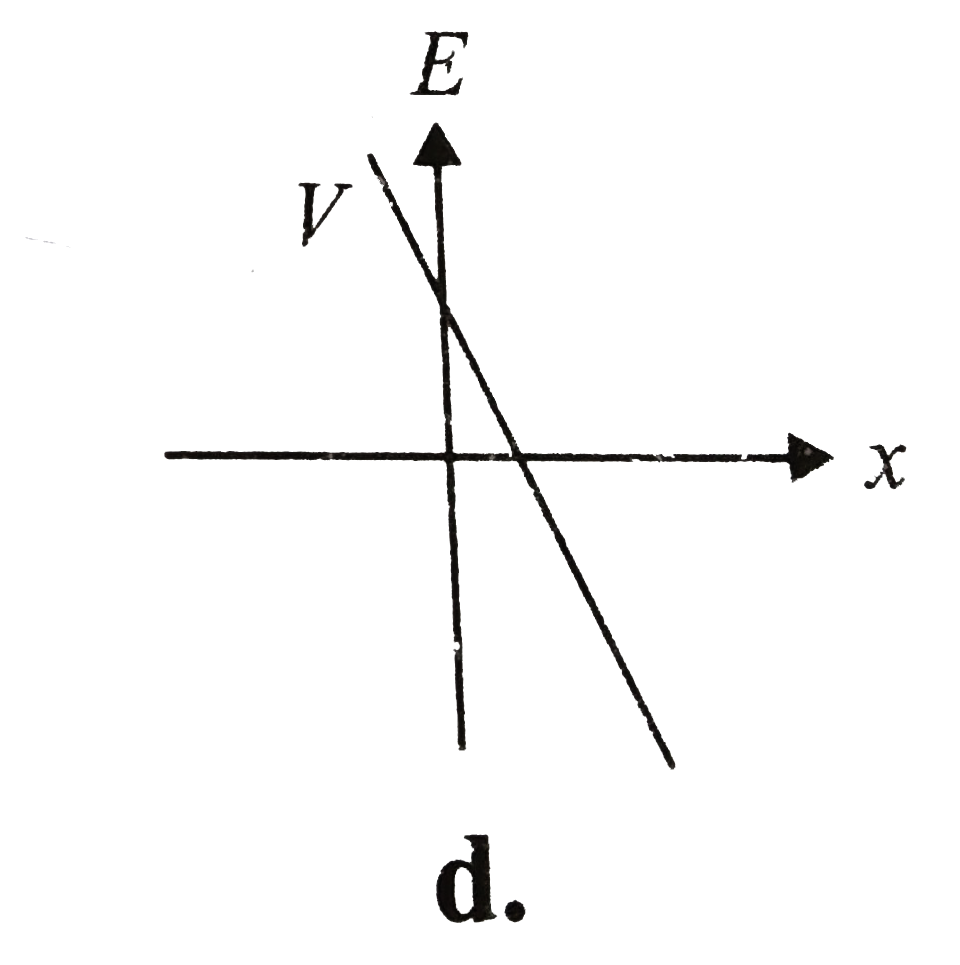
Video Solution
Text Solution
The correct Answer is:C
or
|
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 3Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 3Exercise:Interger Answer type
Explore 20 Videos
- MISCELLANEOUS VOLUME 3Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 3Exercise:Fill in the blanks
Explore 10 Videos
- MISCELLANEOUS VOLUME 3Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 3Exercise:Assertion and Reason Type
Explore 8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:CENGAGE PHYSICSChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:Question Bank
Explore 4 Videos
- MISCELLANEOUS VOLUME 5Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 5Exercise:Integer
Explore 12 Videos
Similar Questions
Knowledge Check
We know that electric field (E ) at any point in space can be calculated using the relation
→E=−δVδxˆi−δVδyˆj−δVδzˆk

if we know the variation of potential (V) at that point. Now let the electric potential in volt along the x-axis vary as V=2x2, where x is in meter. Its variation is as shown in figure
Will the particle perform a simple harmonic motion? Also, find the time period of its oscillations.AYes, time period =2πs.BNo, time period =2πsCYes, time period =4πs.DThe particle will perform SHM, but time period cannot be found from the given data.We know that electric field (E ) at any point in space can be calculated using the relation
→E=−δVδxˆi−δVδyˆj−δVδzˆk

if we know the variation of potential (V) at that point. Now let the electric potential in volt along the x-axis vary as V=2x2, where x is in meter. Its variation is as shown in figure
A charge particle of mass 10 mg and charge 2.5μC is released from rest at x=2m. Find its velocity when it crosses origin.A0.5ms−1B1ms−1C2ms−1D4ms−1In a certain region of space, variation of potential with distance from origin as we move along x-axis is given by V=8x2+2, where x is the x-coordinate of a point in space. The magnitude of electric field at a point ( -4,0) is
A−16V/mB16V/mC−64V/mD64V/m
The electric potential V at any point in space is given V=20x3 volt, where x is in meter. Calculate the electric intensity at point P (1, 0, 2).
View Solutionthe electric potential in the region of space is given by :V(x)=A+Bx+Cx2 where V is in volts,x is in meters and A,B,C are constants.Then,
View SolutionThe variation of potential with distance R from a fixed point is shown in figure. The electric field at R = 5 m is -
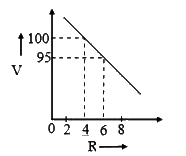
View SolutionThe electric potential at a point (x,y,z) is given by V=−s2
View SolutionLet V be the electric potential at a given point. Then the electric field Ex along x-direction at that point is given by :
View Solution

 .
.