What is the age of an ancient wooden piece if it is known that the specific activity of C14 nuclide in it amouts to 3/5 of that in fresh trees? Given: the half of C nuclide is 5570years and loge(5/3)=0.5 .
1000years
2000years
3000years
4000years
The correct Answer is:d
To solve the problem of determining the age of an ancient wooden piece based on the specific activity of the C14 nuclide, we can follow these steps:
Step 1: Understand the relationship between the activities
Let \( n_0 \) be the number of C14 atoms in fresh trees. The number of C14 atoms in the ancient wooden piece is given as \( \frac{3}{5} n_0 \).
Step 2: Relate the number of atoms to time
The number of radioactive atoms decreases over time according to the equation:
\(
N(t) = N_0 e^{-\alpha t}
\)
where:
- \( N(t) \) is the number of atoms at time \( t \),
- \( N_0 \) is the initial number of atoms,
- \( \alpha \) is the decay constant,
- \( t \) is the time elapsed.
Substituting the values, we have:
\(
\frac{3}{5} n_0 = n_0 e^{-\alpha t}
\)
Step 3: Simplify the equation
Dividing both sides by \( n_0 \) (assuming \( n_0 \neq 0 \)):
\(
\frac{3}{5} = e^{-\alpha t}
\)
Step 4: Take the natural logarithm
Taking the natural logarithm of both sides gives:
\(
\ln\left(\frac{3}{5}\right) = -\alpha t
\)
Step 5: Express \( \alpha \) in terms of half-life
The decay constant \( \alpha \) is related to the half-life \( T_{1/2} \) by the formula:
\(
\alpha = \frac{\ln(2)}{T_{1/2}}
\)
Given that the half-life of C14 is \( 5570 \) years, we have:
\(
\alpha = \frac{\ln(2)}{5570}
\)
Step 6: Substitute \( \alpha \) into the equation
Substituting \( \alpha \) into the equation:
\(
\ln\left(\frac{3}{5}\right) = -\frac{\ln(2)}{5570} t
\)
Step 7: Solve for \( t \)
Rearranging gives:
\(
t = -\frac{5570 \ln\left(\frac{3}{5}\right)}{\ln(2)}
\)
Step 8: Calculate \( \ln\left(\frac{3}{5}\right) \)
Using the property of logarithms:
\(
\ln\left(\frac{3}{5}\right) = \ln(3) - \ln(5)
\)
We can use the given value \( \ln\left(\frac{5}{3}\right) = 0.5 \), thus:
\(
\ln\left(\frac{3}{5}\right) = -0.5
\)
Step 9: Substitute and calculate \( t \)
Now substituting this back into the equation for \( t \):
\(
t = -\frac{5570 \cdot (-0.5)}{\ln(2)}
\)
Step 10: Calculate \( \ln(2) \)
Using the approximate value \( \ln(2) \approx 0.693 \):
\(
t = \frac{5570 \cdot 0.5}{0.693}
\)
Step 11: Final calculation
Calculating this gives:
\(
t \approx \frac{2785}{0.693} \approx 4018.7 \text{ years}
\)
Conclusion
Thus, the age of the ancient wooden piece is approximately \( 4000 \) years.
Let \( n_0 \) be the number of C14 atoms in fresh trees. The number of C14 atoms in the ancient wooden piece is given as \( \frac{3}{5} n_0 \).
The number of radioactive atoms decreases over time according to the equation:
\(
N(t) = N_0 e^{-\alpha t}
\)
where:
- \( N(t) \) is the number of atoms at time \( t \),
- \( N_0 \) is the initial number of atoms,
- \( \alpha \) is the decay constant,
- \( t \) is the time elapsed.
\(
\frac{3}{5} n_0 = n_0 e^{-\alpha t}
\)
Dividing both sides by \( n_0 \) (assuming \( n_0 \neq 0 \)):
\(
\frac{3}{5} = e^{-\alpha t}
\)
Taking the natural logarithm of both sides gives:
\(
\ln\left(\frac{3}{5}\right) = -\alpha t
\)
The decay constant \( \alpha \) is related to the half-life \( T_{1/2} \) by the formula:
\(
\alpha = \frac{\ln(2)}{T_{1/2}}
\)
Given that the half-life of C14 is \( 5570 \) years, we have:
\(
\alpha = \frac{\ln(2)}{5570}
\)
Substituting \( \alpha \) into the equation:
\(
\ln\left(\frac{3}{5}\right) = -\frac{\ln(2)}{5570} t
\)
Rearranging gives:
\(
t = -\frac{5570 \ln\left(\frac{3}{5}\right)}{\ln(2)}
\)
Using the property of logarithms:
\(
\ln\left(\frac{3}{5}\right) = \ln(3) - \ln(5)
\)
We can use the given value \( \ln\left(\frac{5}{3}\right) = 0.5 \), thus:
\(
\ln\left(\frac{3}{5}\right) = -0.5
\)
Now substituting this back into the equation for \( t \):
\(
t = -\frac{5570 \cdot (-0.5)}{\ln(2)}
\)
Using the approximate value \( \ln(2) \approx 0.693 \):
\(
t = \frac{5570 \cdot 0.5}{0.693}
\)
Calculating this gives:
\(
t \approx \frac{2785}{0.693} \approx 4018.7 \text{ years}
\)
Thus, the age of the ancient wooden piece is approximately \( 4000 \) years.
Topper's Solved these Questions
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Linked Comprehension
29 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Integer
6 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Subjective
35 Videos
- MISCELLANEOUS VOLUME 5BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Integer
12 Videos
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Integer Type
4 Videos
Similar Questions
A 25 g piece of characoal is found in some ruins of an ancient city. The sample shows a C14 activity of 250 decay/minutes. How long has the three this charcoal came from been dead ? Given, the half-life of C14 is 5730 years. The ratio of C14andC12 in the living sample is 1.3 ×10−12.
View SolutionThe half-life of a radioactive substance is 5000 years. In how many years, its activity will decay to 0.2 times of its initial value ? Given log105 = 0.6990.
View SolutionIn a smaple of rock, the ration .206Pb to .238U nulei is found to be 0.5. The age of the rock is (given half-life of U238 is 4.5×109 years).
View SolutionHalf - life period of 14C is 5770 years . If and old wooden toy has 0.25% of activity of 14C Calculate the age of toy. Fresh wood has 2% activity of 14C.
View SolutionThe amount of .6C14 isotope in a piece of wood is found to be one-fifth of that present in a fresh piece of wood. Calculate the age of wood (Half life of C14=5577 years)
View SolutionThe age of the wood if only 1/16 part of original C14 is present in its piece is (in years) ( T of C14 is 5,580 years)
View SolutionThe β− activity of a sample of CO2 prepared form a contemporary wood gave a count rate of 25.5 counts per minute (cpm). The same of CO2 form an ancient wooden statue gave a count rate of 20.5cpm, in the same counter condition. Calculate its age to the nearest 50 year taking t1/2 for .14C as 5770 year. What would be the expected count rate of an identical mass of CO2 form a sample which is 4000 year old?
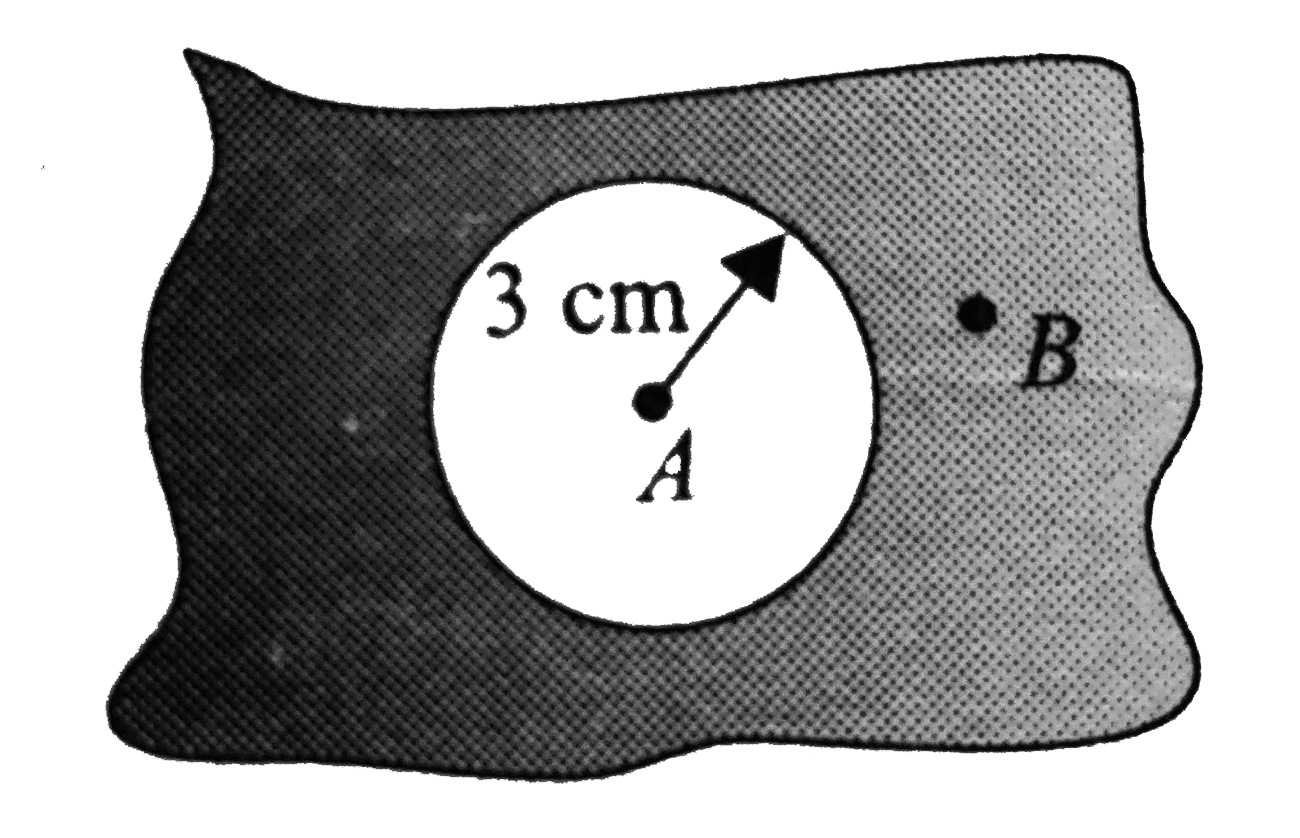
View SolutionFigure shows a point charge of 0.5×10−6 C at the center of the spherical cavity of radius 3 cm of a piece of metal. The electric field at
View SolutionIf 3(c+d)=5, what is the value of c+d?
View SolutionA piece of wood form the ruins of an ancient building was found to have a C14 activity of 12 disintegrations per minute per gram of its carbon content. The C14 activity of the living wood is 16 disintegrations/minute/gram. How long ago did the trees, from which the wooden sample came, die? Given half-life of C14 is 5760 years.
View Solution
