What is the percentage change in the tension necessary in a somometer of fixed length to produce a note one octave lower (half of original frequency) than before?
25%
50%
67%
75%
The correct Answer is:D
To find the percentage change in the tension necessary in a somometer of fixed length to produce a note one octave lower (which corresponds to half of the original frequency), we can follow these steps:
1. Understand the relationship between frequency and tension:
The frequency f of a vibrating string is given by the formula:
f=12L√Tμ
where:
- L is the length of the string (fixed in this case),
- T is the tension in the string,
- μ is the mass per unit length of the string.
2. Establish the relationship between frequency and tension:
From the formula, we can see that frequency is directly proportional to the square root of the tension:
f∝√T
Therefore, if the frequency changes, the tension must change accordingly.
3. Define the frequencies:
Let f1 be the original frequency and f2 be the new frequency (one octave lower). Since one octave lower means half the frequency:
f2=12f1
4. Relate the tensions:
Using the relationship between frequency and tension:
f1f2=√T1T2
Substituting f2:
f112f1=√T1T2⟹2=√T1T2
5. Square both sides:
Squaring both sides gives:
4=T1T2⟹T1=4T2
6. Calculate the percentage change in tension:
The percentage change in tension is given by:
Percentage Change=T1−T2T1×100
Substituting T1=4T2:
Percentage Change=4T2−T24T2×100=3T24T2×100=34×100=75%
Final Answer:
The percentage change in tension necessary to produce a note one octave lower is 75%.
The frequency f of a vibrating string is given by the formula:
f=12L√Tμ
where:
- L is the length of the string (fixed in this case),
- T is the tension in the string,
- μ is the mass per unit length of the string.
From the formula, we can see that frequency is directly proportional to the square root of the tension:
f∝√T
Therefore, if the frequency changes, the tension must change accordingly.
Let f1 be the original frequency and f2 be the new frequency (one octave lower). Since one octave lower means half the frequency:
f2=12f1
Using the relationship between frequency and tension:
f1f2=√T1T2
Substituting f2:
f112f1=√T1T2⟹2=√T1T2
Squaring both sides gives:
4=T1T2⟹T1=4T2
The percentage change in tension is given by:
Percentage Change=T1−T2T1×100
Substituting T1=4T2:
Percentage Change=4T2−T24T2×100=3T24T2×100=34×100=75%
The percentage change in tension necessary to produce a note one octave lower is 75%.
Topper's Solved these Questions
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Multiple
26 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Assertion - Reasoning
6 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Subjective
24 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 24
1 Videos
Similar Questions
What is the percentage change in the tension necessary in a sonometer of fixed length to produce a note one octave lower (half of original frequency) than before?
View SolutionWhat is the fractional change in tension in a sonometer wire of constant length to produce a note one octave lower than the previous note?
View SolutionWhat will be the change in resistance of a constantan wire when its radius is made half and length reduced to one-fourth of its original length ?
View SolutionA heavy string is tied at one end to a movable support and to a light thread at the other end as shown in figure. The thread goes over a fixed pulley and supports a weight to produce a tension. The lowest frequency with which the heavy string resonates is 120 Hz. If the movable support is pushed to the right by 10 cm so that the joint is placed on the pulley, what will be the minimum frequency at which the heavy string can resonate ?
 .
.
View SolutionA heavy string is tied at one end to a movable support and to a light thread at the other end as shown in figure. The thread goes over a fixed pulley and supports a wieght to produce a tension. The lowest frequency with which the heavy sting resonates is 120Hz. If the movable support is pushed to the right by 20cm so that the joint is placed on the pulley, what will be the minimum frequency at which the heavy string can resonate?
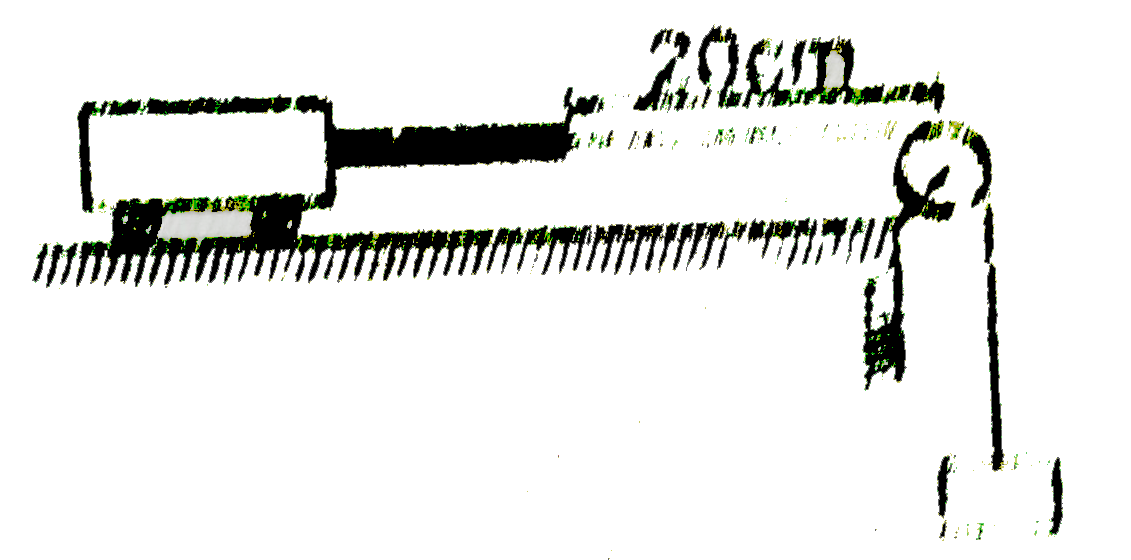
View SolutionThe fundatmental frequency of a sonometer wire increases by 6Hz if its tension is increased by 44% keeping the length constant. Find the change in the fundamental frequency of the sonometer when the length of the wire is increased by 20% keeping the original tension in the wire.
View SolutionTwo sitar strings A and B playing the note 'Ga' are slightly out of tune and produce beats of frequency 6 Hz. The tension in the string A is slightly reduced and the beat frequency is found to reduce to 3 Hz. If the original frequency of A is 324 Hz, what is the frequency of B?
View SolutionTivo sitar strings A and B playing the note 'Ga'are slightly out of tunc and produce bedis of frequency 6 Hz. The tension in the string A is slightly reduced and the bear frequency is found to reduce to 3 Hz If the original frequency of A is 324 Hz, what is the frequency of B?
View SolutionTwo wires are in unison. If the tension in one of the wires is increased by 2%, 5 beats are produced per second. The initial frequency of each wire is
View SolutionA sonometer wire of 70cm length fixed at one end has a solid mass M, hanging from its other end to produce tension in it. The wire produces a certian frequency. When the same mass 'M' hanging in water, it is found that the length of the wire has to be changed by 5 cm. in order to produce the same frequency. Then the density of the material of mass 'M' is
View Solution
