What length of copper wire of specific resistance 1.7×10−8Ω m and radius 1 mm is required so that its resistance is 1Ω ?
To find the length of copper wire required for a resistance of 1 ohm, we can use the formula for resistance:
R=ρ⋅LA
where:
- R is the resistance,
- ρ is the specific resistance,
- L is the length of the wire,
- A is the area of cross-section of the wire.
Step 1: Identify the given values
- Specific resistance ρ=1.7×10−8Ωm
- Resistance R=1Ω
- Radius r=1mm=1×10−3m
Step 2: Calculate the area of cross-section A
The area A of a circular cross-section can be calculated using the formula:
A=πr2
Substituting the value of r:
A=π(1×10−3)2
A=π×10−6
Step 3: Substitute the known values into the resistance formula
Now we can substitute the values into the resistance formula:
1=1.7×10−8⋅Lπ×10−6
Step 4: Rearranging the equation to solve for L
To isolate L, we can rearrange the equation:
L=1⋅π×10−61.7×10−8
Step 5: Calculate L
Now we can calculate L:
L=π×10−61.7×10−8
Calculating this gives:
L≈3.14×10−61.7×10−8
L≈184.7m
Final Answer
The length of copper wire required so that its resistance is equal to 1 ohm is approximately 184.7 meters.
---
- R is the resistance,
- ρ is the specific resistance,
- L is the length of the wire,
- A is the area of cross-section of the wire.
- Specific resistance ρ=1.7×10−8Ωm
- Resistance R=1Ω
- Radius r=1mm=1×10−3m
The area A of a circular cross-section can be calculated using the formula:
A=π×10−6
Now we can substitute the values into the resistance formula:
To isolate L, we can rearrange the equation:
Now we can calculate L:
L≈184.7m
The length of copper wire required so that its resistance is equal to 1 ohm is approximately 184.7 meters.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(B)
15 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(B) (MULTIPLE CHOICE TYPE)
3 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(A) ( MULTIPLE CHOICE TYPE)
2 Videos
- CALORIMETRYBOOK - ICSECHAPTER - CALORIMETRYEXERCISE - EXERCISE-11(B) (NUMERICALS)
15 Videos
- ELECTRO-MAGNETISM BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS
1 Videos
Similar Questions
Knowledge Check
The potentiometer wire of length 200cm has a resistance of 20Ω. It is connected in series with a resistance 10Ω and an accumulator of emf 6V having negligible internal resistance. A source of 2.4V is balanced against length 1 of the potentiometer wire. Find the length l of the potentiometer wire. Find the length l
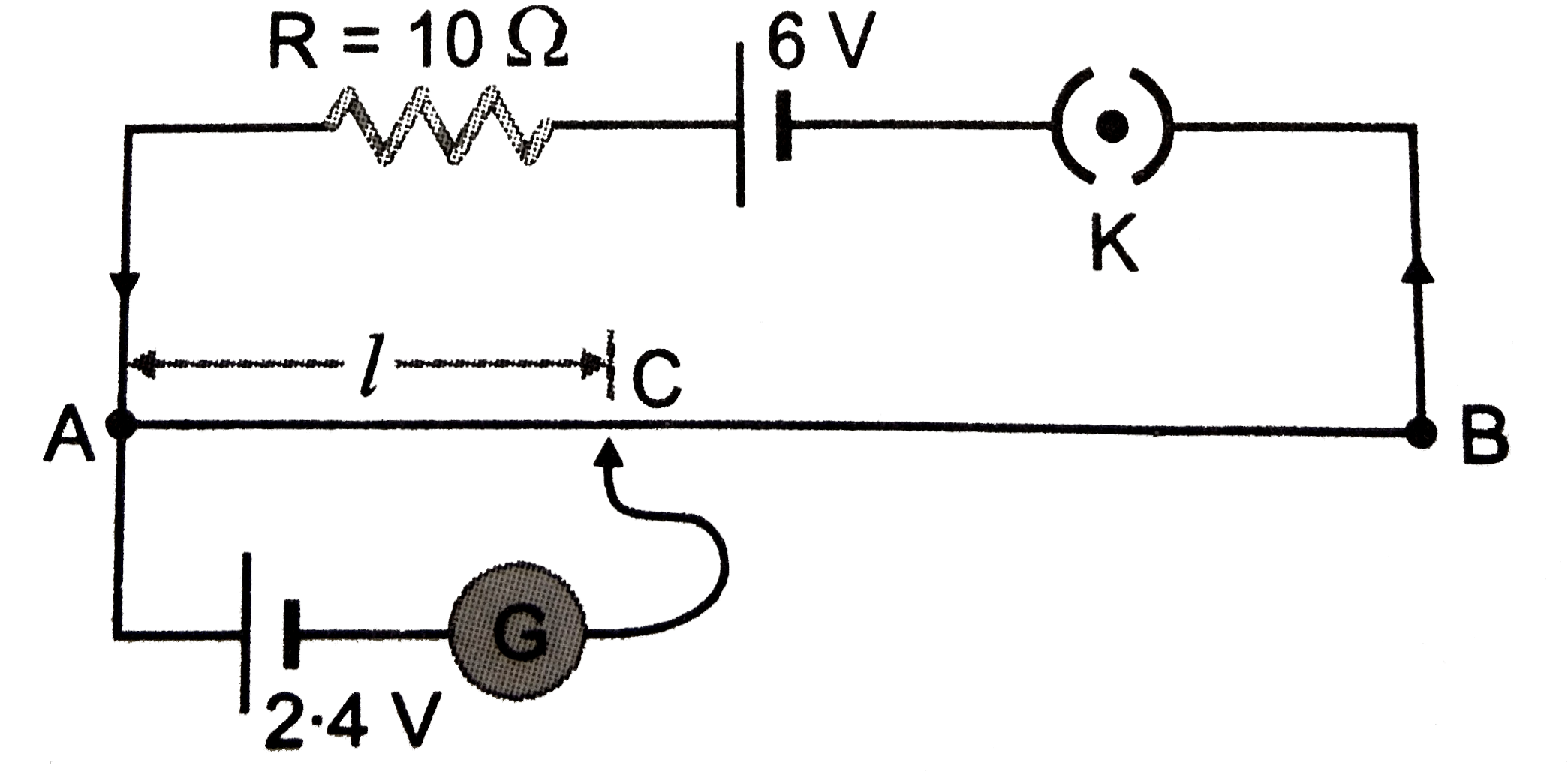 A100cmB120cmC110cmD140cm
A100cmB120cmC110cmD140cm
What length of a copper wire of cross-sectional area 0.01mm2 will be needed to prepare a resistance of 1kΩ? Resistivity of copper =1.7×10−8Ωm.
View SolutionWhen the resistance of copper wire is 0.1Ω and the radius is 1mm, then the length of the wire is (specific resistance of copper is 3.14×10−8ohm×m)
View SolutionIn a meter bridge circuit, the two resistances in the gap are 5Ω and 10Ω. The wire resistance is 4Ω. The emf of the cell connected at the ends of the wire is 5V and its internal resistance is 1Ω. What current will flow through the galvenometer of resistance 30Ω if the contact is made at the midpoint of wire ?
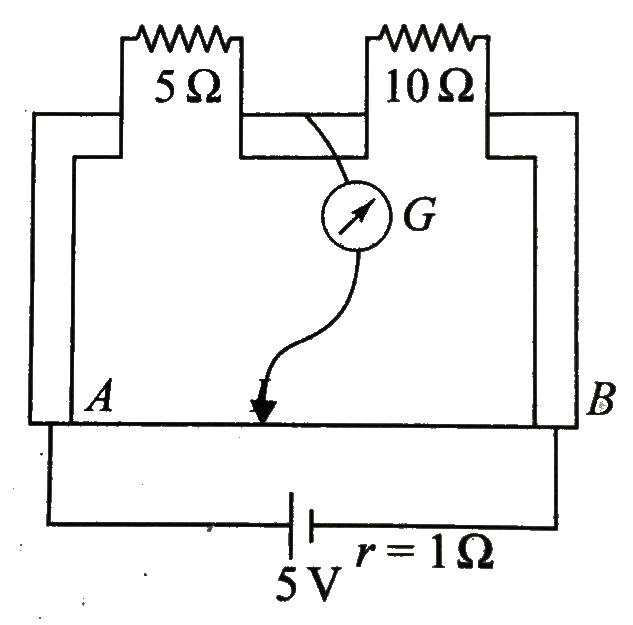
View SolutionA given wire of resistance 1 Ω is stretched to double its length. What will be its new resistance ?
View SolutionA potentiometer wire of length 10m and resistance 30Ω is connected in series with a battery of emf 2.5V, internal resistance 5Ω and an external resistance R. If the fall of potential along the potentiometer wire is 50μVmm−1, then the value of R is found to be 23nΩ. What is n?
View SolutionThe temperature coefficient of resistance of the material of a wire is 0.00125∘C−1. Its resistance at 27∘C is 1Ω. At what temperature will its resistance be 2Ω ?
View SolutionA current of 5 A passss through a copper conductor (resistivity = 1.7×10−8Ωm) of radius of cross -section 5mm. Find the mobility of the charges if their drift velocity is 1.1×10−3m/s.
View Solution
