When an uncharged conducting ball of radius R is placed in an external uniform electric field, a surface charge densityh σ=σ0cosθ is induced on the ball's surface charge (here σ0 is a constant, θ is a polar angle). Find the magnitude of the resultant electric force acting on an induced charge of the same sign.
We know that the force acting on the area element dS of a conductor is,
d→F=12σ→EdS …(1)
It follows from symmetry considerations that the resultant force →F is directed along the z-axis, and hence it can represented as the sum (intergal) of the projection of elementary forces (i) onto the z-axis :
dFx=dFcosθ ...(2)
For simplicity let us consider an element area dS=2πRsinθRdθ (Fig). Now considering that E=σ/ε0. Equaction (2) takes teh form
dFx=πσ2R2ε0sinθcosθdθ
=−(πσ20R2ε0)cos2θdcosθ
Intergating this expression over the half sphere (i.e with respect to cosθ between 1 and 0), we obtain F=Fx=πσ20R24ε0

Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Capacitance - Energy Of An Electric Field46videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Current72videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Motion Of Charged Particle In Magnetic Field36videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions6videos
ELECTRICITY AND MAGNETISM
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions24videos
ELECTROMAGNETISM
Similar Questions
A spherical shell is uniformly charged with the surface density σ. Using the energy conservation law, find the magnitude of the electric force acting on a unit area of the shell.
View SolutionA solid conducting sphere of radius R is placed in a uniform electric field E as showo in figure. Due to electric field non uniform surface charges are induced on the surface of the sphere. Consider a point A on the surface of sphere at a polar angle θ from the direction of electric field as shown in figure. Find the surface density of induced charges at point A in terms of electric field and polar angle θ : lt brgt
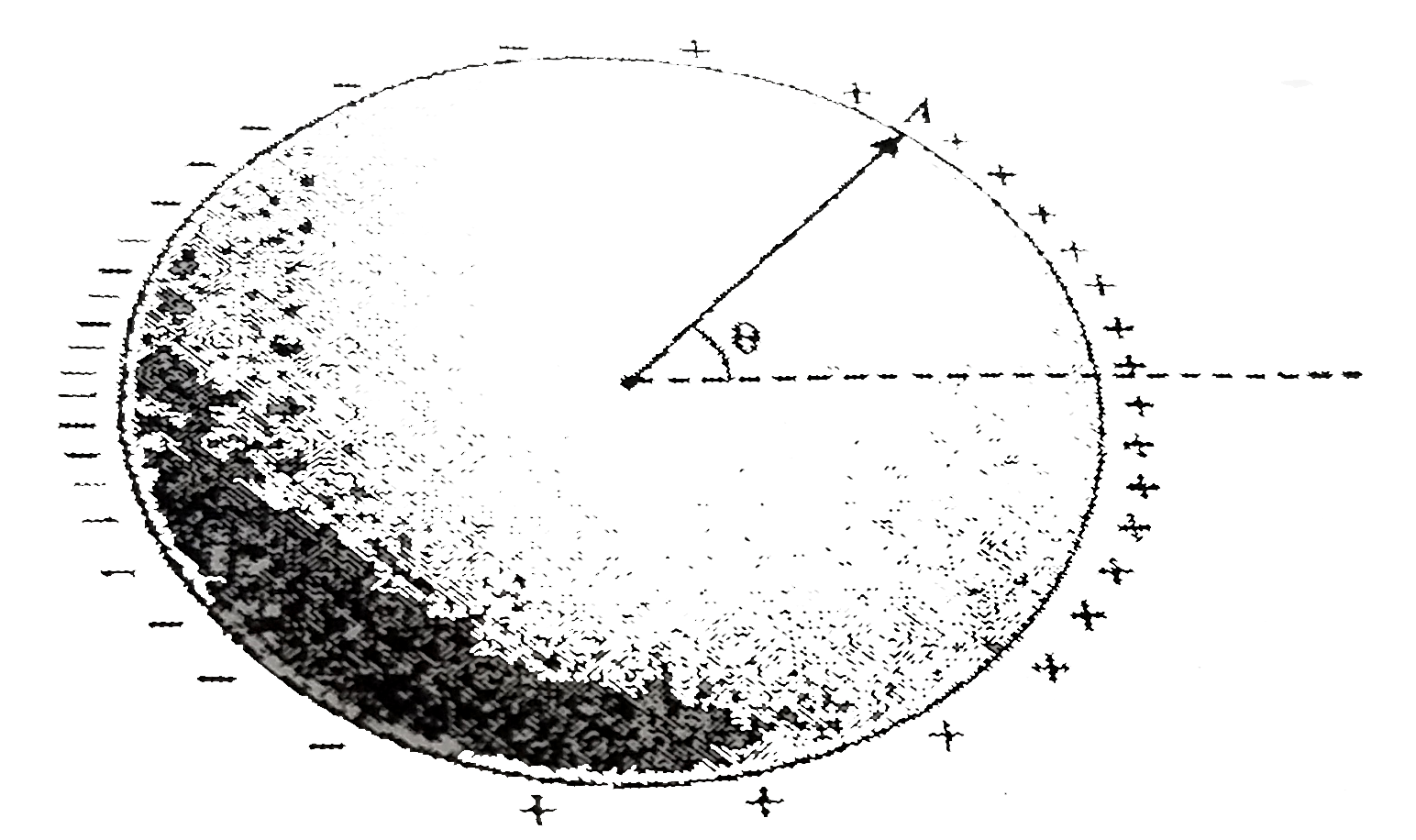 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
An uncharged conducting large plate is placed as shown. Now an electric field E towards right is applied. Find the induced charge density on the right surface of the plate.
 A−ε0EBε0EC−2ε0ED2ε0E
A−ε0EBε0EC−2ε0ED2ε0E - Question 2 - Select One
For the hemisphere of radius R shown in the figure the surface charge density σ varies with θ (shown in figure) as σ=σ0cosθ the total charge on the sphere is
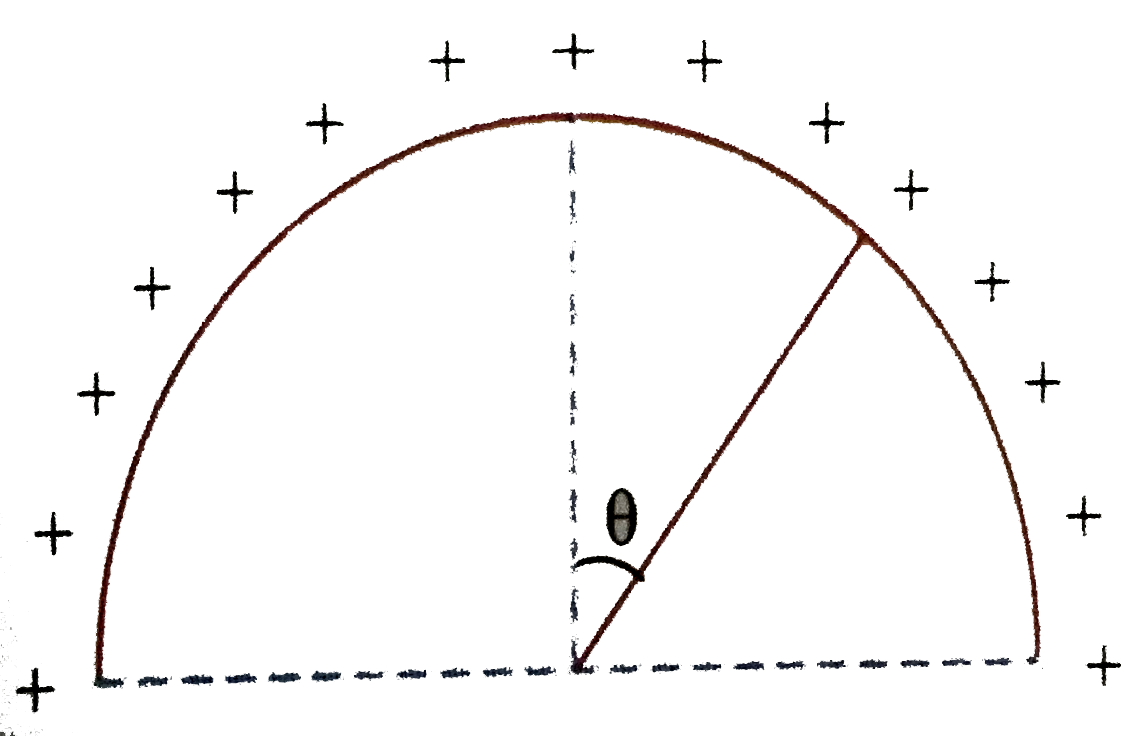 A2σ0πR2Bσ0πR22Cσ0πR23Dσ0πR2
A2σ0πR2Bσ0πR22Cσ0πR23Dσ0πR2 - Question 3 - Select One
The electric field near a conducting surface having a uniform surface charge denstiy σ is given by
Aσε0 and is parallel to the surfaceB2σε0Cσε0 and is normal to the surfaceD2σε0 and is normal to the surface
Electric field at the centre of uniformly charge hemispherical shell of surface charge density σ is σnε0 then find the value of n.
 View Solution
View SolutionWhat is the direction of the electric field at the surface of a charged conductor having charge density σ <0?
View SolutionRepeat the question if sphere is a hollow non-conducting sphere of radius R and has uniform surface charge density σ.
View SolutionA uniform disc of radius R is charged with a uniform surface charge density σ. Find the electric potential due to the charges on the disc at a point on its edge. [σRπ∈0]
View SolutionShow that the electric field at the surface of a charged conductor is given by →E=σε0ˆn , where σ is the surface charge density and ˆn is a unit vector normal to the surface in the outward direction .
View Solution

