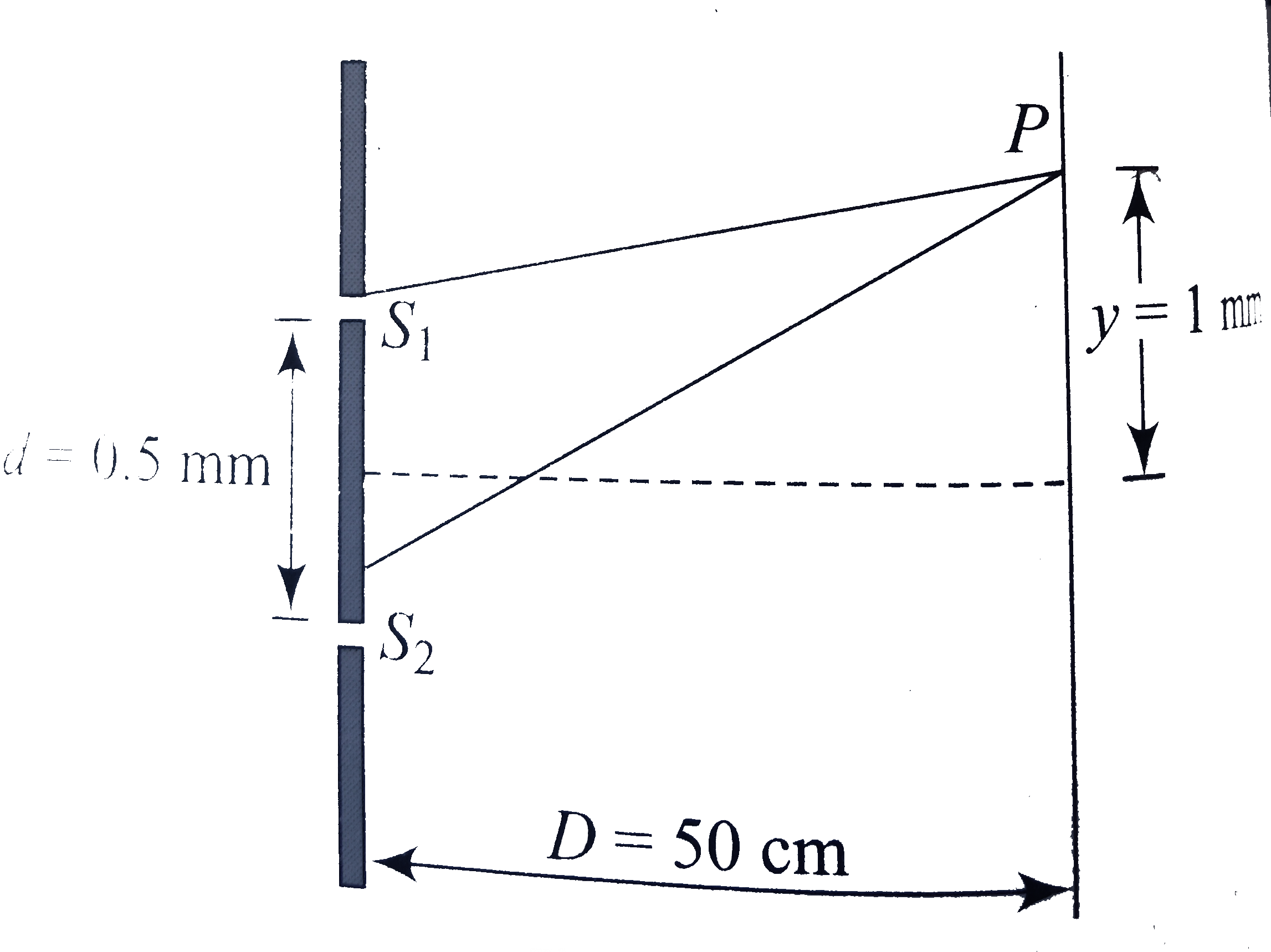
White coherent light (400 nm-700 nm) is sent through the slits of a Young's double slit experiment. The separation between the slits is 05 mm and the screen is 50 cm away from the slits. There is a hole in the screen at a point 1.0 mm away (along the width of the fringes) from the central line. (a) Which wavelength(s) will be absent in the light coming from the hole ? (b) which wavelength(s) will have a strong intensity ?

a. The absent wavelength will correspond to minima at position order of minima corresponding to 4000Å,
yn=(2n−1)Dλ2d⇒(2n−1)
yn2dDλ⇒n=12[2dynDλ+1]
n1=12[0.5×10−3×1×10−3×250×10−2×4000×10−10+1]
=12[2×1044000+1]=3
Order or minima corresponding to 7000Å,
n2=12[2×1047000+1]=1.9
Number of integers between 1.9 and 3.0 are 2 and 3.
Wavelength corresponding to n=2 is
λ(2)=yn2d(2n−1)D=1×10−3×2×0.5×10−3(2×2−1)×50×10−2
yn=nDλd⇒n=yndDλ=1×10−3×0.5×10−350×10−2×λ
n4000=10−64000×10−10=100004000=2.5
and n7000=100007000=1.4
Integer between 1.4 and 2.5 is 2.
∴λ(2)=yndnD=10−62=500nm
 .
.
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Solved Examples
10 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Exercise 2.1
12 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - QUESTION BANK
34 Videos
- WAVESBOOK - CENGAGE PHYSICSCHAPTER - WAVESEXERCISE - QUESTION BANK
38 Videos
Similar Questions
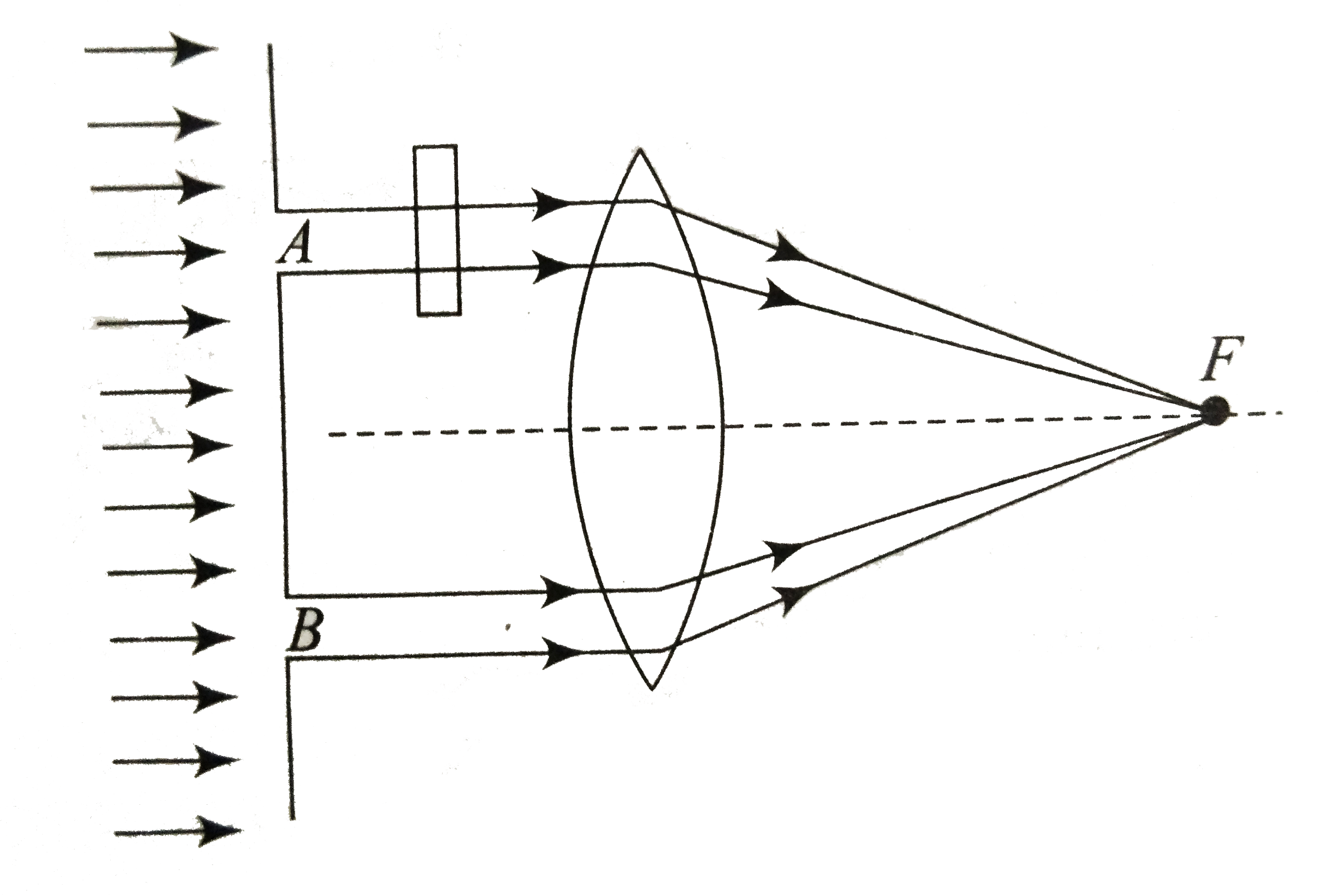
In a modified Young's double-slit experiment, a monochromatic uniform and parallel beam of light of wavelength 6000Å and intensity (10/π) W m−2 is incident normally on two circular apertures A and B of radii 0.001 m and 0.002 m, respectively. A perfectly transparent film of thickness 2000Å and refractive index 1.5 for the wavelength of 6000Å is placed in front of aperture A (see the figure). Calculate the power (in mW) received at the focal spot F of the lens. Then lens is symmetrically placed with respect to the aperture. Assume that 10% of the power received by each aperture goes in the original direction and is brought to the focal spot.
View SolutionTwo coherent sources of light of intensity ratio β produce interference pattern. Prove that in the interferencepattern
Imax−IminImax+(Imin)=2√β1+β
where Imax and Imin are maximum and mininum intensities in the resultant wave.
View SolutionThe figure shows two paths through which a gas can be taken from state A to state B. If the ratio of work done by the gas in the two paths is xy, then find (x+y).
'(##CEN_KSR_PHY_JEE_C15_E01_001_Q01##)'
View SolutionA string of length 1m fixed at both ends is vibrating in 3rd overtone. Tension in string is 200N and linear mass density is 5 gmin. Frequency of these vibrations is (in Hz ).
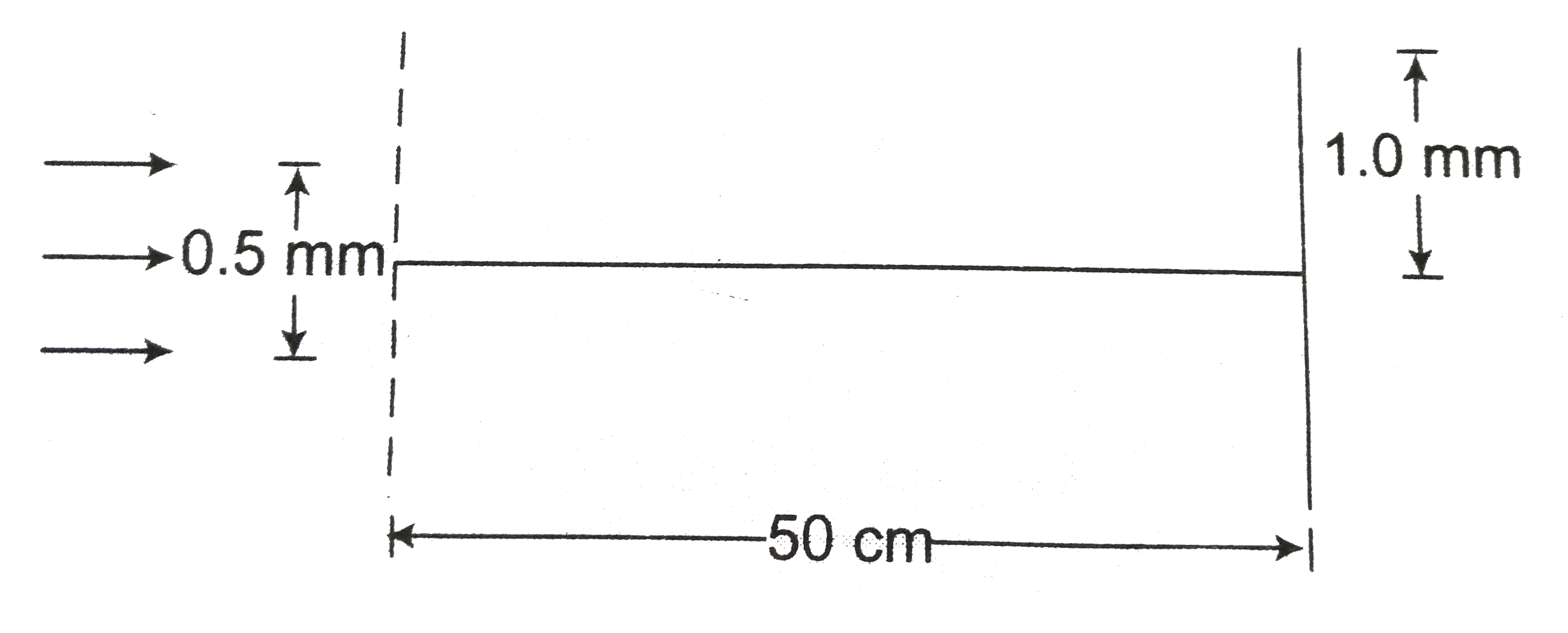
View SolutionWhite coherent light (400nm−700nm) is sent through the slits of a YDSE. D=0.5mm, D=50 cm. There is a hole in the screen at a point 1.0mm away (along the width of the fringes) from the central line.
(a) Which wavelength will be absent in the light coming from the hole?
(b) Which wavelength(s) will have a strong intensity?
View SolutionIn a Young's double slit experiment, the separation between the slits = 2.0 mm, the wavelength of the light = 600 nm and the distance of the screen from the slits = 2.0 m. If the intensity at the centre of the central maximum is 0.20Wm−2, what will be the intensity at a point 0.5 cm away from this centre along the width of the fringes ?
View SolutionIn Young's double slit experiment, the two slits 0.15mm apart are illuminated by light of wavelength 450nm. The screen is 1.0m away from the slits. Find the distance of second bright fringe and second dark fringe from the central maximum. How will the fringe pattern change if the screen is moved away from the slits ?
View SolutionIn Young's double slit experiment, the distance between the slits is 1 mm and that between slit and screen is 1 meter and 10th fringe is 5 mm away from the central bright fringe, then wavelength of light used will be
View SolutionIn Young's double slit experiment the slits are separated by 0.24 mm. The screen is 2 m away from the slits . The fringe width is 0.3 cm. Calculate the wavelength of the light used in the experiment.
View SolutionWhite coherent light (400 nm-700 nm) is sent through the slits of a Young's double slit experiment (as shown in the figure). The separation between the slits is 1 mm and the screen is 100 cm away from the slits. There is a hole in the screen at a point 1.5 mm away (along the width of the fringes) from the central line. (a) For which wavelength (s) there will be minima at that point ? (b) which wavelength (s) will have a maximum intensity?
View SolutionIn a double experiment, the distance between the slit is 1 mm and screen is 25 cm away from the slits. The wavelength of light is 6000Å. The width of the fringe on the screen is
View SolutionIn Young's double slit experiment shown in figure S1 and S2 are coherent sources and S is the screen having a hole at a point 1.0 mm away from the central line. White light (400 to 700nm) is sent through the slits. Which wavelength passing through the hole has strong intensity?
View SolutionIn Young's double-slit experiment the distance between the slits is 1 mm and that between slit and screen is 1m. If 10th bright fringe is 5 mm away from the central bright fringe, then wavelength of light used will be
View SolutionWhite coherent light (400 nm - 700 nm) is sent through the slits of a Young's double slit experiment. The separation between the slits is 0.5 mm and the screen is 50 cm away from the slits. There is a hole in the screen at a point away (along the width of the fringes) from the central line. Which wavelengths (s) will be absent in the light coming from the hole ?

View SolutionIn Young's double slit experiment, the two slits 0.15mm apart are illuminated by light of wavelength 450nm. The screen is 1.0m away from the slits. Find the distance of second bright fringe and second dark fringe from the central maximum. How will the fringe pattern change if the screen is moved away from the slits ?
View SolutionIn a Young's double slit experiment, the separation between the slits = 2.0 mm, the wavelength of the light = 600 nm and the distance of the screen from the slits = 2.0 m. If the intensity at the centre of the central maximum is 0.20Wm−2, what will be the intensity at a point 0.5 cm away from this centre along the width of the fringes ?
View Solution
