White light is incident on a soap film of μ=4/3 at an angle of 30∘. On examining the transmitted light with a spectrometer a dark band of wavelength 5.5×10−7 m is found. Find the minimum thickness of the film.
To find the minimum thickness of the soap film where a dark band is observed for a wavelength of light, we can use the concept of thin film interference. The condition for destructive interference (dark band) in a thin film is given by the formula:
2t=(m+12)λ/n
Where:
- t is the thickness of the film,
- m is the order of interference (0, 1, 2, ...),
- λ is the wavelength of light in vacuum,
- n is the refractive index of the film.
Step 1: Identify the given values
- Wavelength of light, λ=5.5×10−7 m
- Refractive index of the soap film, n=43
- For the first minimum (dark band), we take m=0.
Step 2: Apply the formula for minimum thickness
Using the formula for destructive interference:
2t=(m+12)λn
Substituting m=0:
2t=12λn
Step 3: Rearranging for thickness t
Now, we can rearrange the formula to find t:
t=λ4n
Step 4: Substitute the values
Now we can substitute the values of λ and n:
t=5.5×10−74×43
Step 5: Calculate the thickness
Calculating the denominator:
4×43=163
Now substituting this back into the equation for t:
t=5.5×10−7163
This simplifies to:
t=5.5×10−7×316
Calculating this gives:
t=16.5×10−716
t=1.03125×10−7 m
Step 6: Final answer
Thus, the minimum thickness of the soap film is approximately:
t≈1.03×10−7 m
- t is the thickness of the film,
- m is the order of interference (0, 1, 2, ...),
- λ is the wavelength of light in vacuum,
- n is the refractive index of the film.
- Wavelength of light, λ=5.5×10−7 m
- Refractive index of the soap film, n=43
- For the first minimum (dark band), we take m=0.
Using the formula for destructive interference:
Now, we can rearrange the formula to find t:
Now we can substitute the values of λ and n:
Calculating the denominator:
Thus, the minimum thickness of the soap film is approximately:
Topper's Solved these Questions
Similar Questions
White light is incident on a soap film of μ=4/3 at an angle of 45∘. On examining the transmitted light with a spectrometer, a bright band of wavelength 5400Å is found. What will be the minimum thickness of the soap film?
View SolutionWhite light is incident on a soap film at an angle of 60∘. On examining the reflecting light by a spectroscope, a dark band corresponding to wavelength 6200Å is found. Find the minimum thickness of the film. Take refractive index of film = 1.33.
View Solution
Knowledge Check
A ray of light is incident at an angle of 45∘ (ralative to the normal) on a thin film of a liquid with an index of refraction of √2. Assuming that the medium of both sides of the film is air, find the minimum thickness of the film required for constructive interference in the reflected light for sodium light of wavelenght 600 nm.
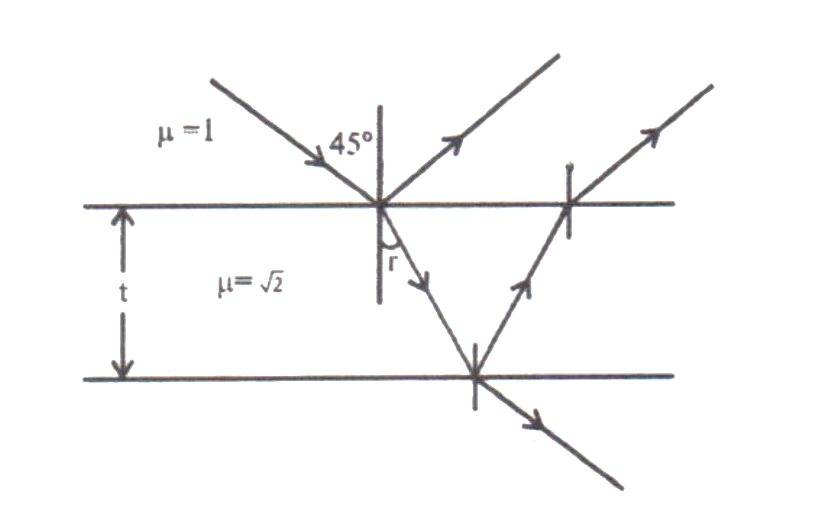 A122 nmB212 nmC173 nmD320 nm
A122 nmB212 nmC173 nmD320 nmWhen light is incident on a soap film of thickness 5×10−5cm, wavelength which is maximum reflected in the visible region is 5320Å. The refractive index of the film will be :
A1.22B1.33C1.51D1.83
A soap film of μ=43 is illuminated by white light incident at an angle of 45∘. The transmitted light is examined by spectrospe and bright fringe is found to be for wavelength of 6000Å. Find the minimum thickness of the film.
View SolutionA soap film is illuminated by while light incient at an angle of 30∘. The reflected light is examined by a spectroscope in which a dark band corresponding to wavelength 6000Å is found . Calculate the minimum thickness of the film. Given refractive index of film=43
View SolutionA soap bubble of µ=4/3 is illuminated by white light incident at an angle of 30∘. The transmittedlight is examined by a spectroscope and bright band is found for a wavelength of 6000 Å. Find the thickness of the film.
View SolutionA soap film of refractive index 4/3 and thickness 1.5×10−4cm is illuminated by white light incident at angle 45∘. The reflected light is examined by a spectroscope in which a dark band corresponding to the wavelength 5×10−5 cm is found. Find the order of the interference band
View SolutionWhite light is incident on a soap film at an angle of sin−145 and the reflected light on examination by the spectroscope shows dark bands. The consecutive dark bands corresponds to wavelength 6100 Å and 6000 Å. If the refractive index of the film is 4/3, calculate its thickness.
View SolutionWhite light incident perpendicular on a soap film gets reflected . It has an interference maximum at 5800Å and a minimum at 4200Å in a visible spectrum. If there is no minimum in between the two bands, find the thickness of the soap film. Take refractive index of film = 4/3.
View Solution
