White light is used to illuminate the two slits in a Young's double slit experiment. The separation between the slits is b and the screen is at a distance d(>>b) from the slits At a point on the screen directly in front of one of the slits, certain wavelengths are missing some of these missing wavelengths are
λ=b2=b2/d
λ=b2=2b2/d
λ=b2=b2/3d
λ=2b2=b2/3d
The correct Answer is:A, C
To solve the problem of determining the missing wavelengths in a Young's double slit experiment with white light, we can follow these steps:
1. Understanding the Setup:
- We have two slits separated by a distance b.
- A screen is placed at a distance d from the slits.
- We are looking for the wavelengths that are missing at a point on the screen directly in front of one of the slits.
2. Condition for Dark Fringes:
- The condition for dark fringes in a double-slit experiment is given by the formula:
y=(2n−1)λdb
- Here, y is the distance from the central maximum to the dark fringe, n is the order of the dark fringe, λ is the wavelength of light, d is the distance from the slits to the screen, and b is the separation between the slits.
3. Finding the Position of Dark Fringes:
- At the point directly in front of one of the slits, the distance y can be set to b2 (half the distance between the slits).
- Substituting y=b2 into the dark fringe formula gives:
b2=(2n−1)λdb
4. Rearranging the Equation:
- Cross-multiplying gives:
b2=(2n−1)λd
- Rearranging for λ gives:
λ=b2(2n−1)d
5. Calculating Missing Wavelengths:
- For different values of n:
- For n=1:
λ1=b21⋅d=b2d
- For n=2:
λ2=b23d
- For n=3:
λ3=b25d
6. Identifying Missing Wavelengths:
- The wavelengths that are missing at the point directly in front of one of the slits are:
- b2d
- b23d
- b25d
7. Conclusion:
- The missing wavelengths correspond to the values derived above. Thus, the correct answers for the missing wavelengths are b2d and b23d.
- We have two slits separated by a distance b.
- A screen is placed at a distance d from the slits.
- We are looking for the wavelengths that are missing at a point on the screen directly in front of one of the slits.
- The condition for dark fringes in a double-slit experiment is given by the formula:
y=(2n−1)λdb
- Here, y is the distance from the central maximum to the dark fringe, n is the order of the dark fringe, λ is the wavelength of light, d is the distance from the slits to the screen, and b is the separation between the slits.
- At the point directly in front of one of the slits, the distance y can be set to b2 (half the distance between the slits).
- Substituting y=b2 into the dark fringe formula gives:
b2=(2n−1)λdb
- Cross-multiplying gives:
b2=(2n−1)λd
- Rearranging for λ gives:
λ=b2(2n−1)d
- For different values of n:
- For n=1:
λ1=b21⋅d=b2d
- For n=2:
λ2=b23d
- For n=3:
λ3=b25d
- The wavelengths that are missing at the point directly in front of one of the slits are:
- b2d
- b23d
- b25d
- The missing wavelengths correspond to the values derived above. Thus, the correct answers for the missing wavelengths are b2d and b23d.
Topper's Solved these Questions
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced C. Comprehension Type Questions. Passage (I)
2 Videos
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced C. Comprehension Type Questions. Passage (II)
2 Videos
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced Only one option is correct
22 Videos
- SOLVED PAPERS 2018BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER
22 Videos
Similar Questions
White light is used to illuminate the two slits in a Young's
double slit experiment. The separation between the slits is b and the screen is at
a distance d(>>b) from the slits. At a point on the screen directly in front of
one of the slits, certain wavelengths are missing. Some of these missing
wavelength are
View SolutionAt a point on the screen directly in front of one of the slits, find the missing wavelengths.
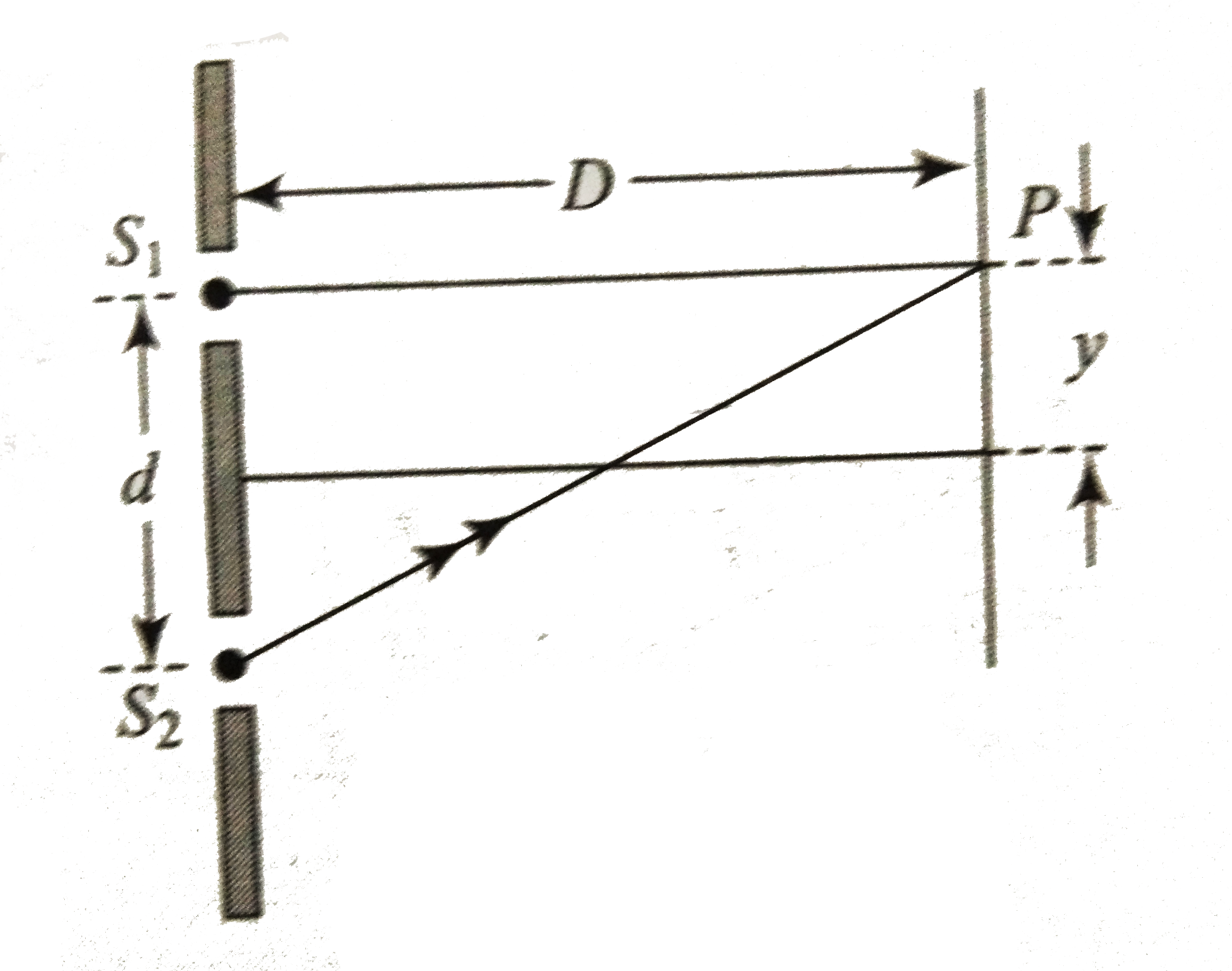
View Solution
Knowledge Check
In a double slit experiment, the distance between the slits is d. The screen is at a distance D from the slits. If a bright fringe is formed opposite to one of the slits, its order is
AdλBλ2dDCD22λDDd22Dλ
In Young's double slit experiment, the separation between the slits is halved and the distance between the slits and the screen is doubled. The fringe width is
View SolutionIn Young's double slit experiment, the sepcaration between the slits is halved and the distance between the slits and the screen is doubled. The fringe width is
View SolutionIn Young's double slit experiment, the sepcaration between the slits is halved and the distance between the slits and the screen is doubled. The fringe width is
View SolutionIn Young's double-slit experiment, the separation between the slits is halved and the distance between the slits and the screen in doubled. The fringe width is
View SolutionThe distance between the tew slits in a Young's double slit experiment is d and the distance of the screen from the plane of the slits is b, P is a point on the screen directly infront of one of the slits. The path difference between the waves arriving at P from the two slits is
View SolutionIn the Young's double slit experiment, the spacing between two slits is 0.1mm. If the screen is kept at a distance of 1.0m from the slits and the wavelength of ligth is 5000Å, then the fringe width is
View SolutionHow does the fringe width, in Young's double-slit experiment, change when the distance of separation between the slits and screen is doubled ?
View Solution
