x-t equation of a particle in SHM is given as x=1.0sin(12πt) in SI units. Potential energy at mean position is zero. Mass of particle is 14 kg. Match the following table (SI units).
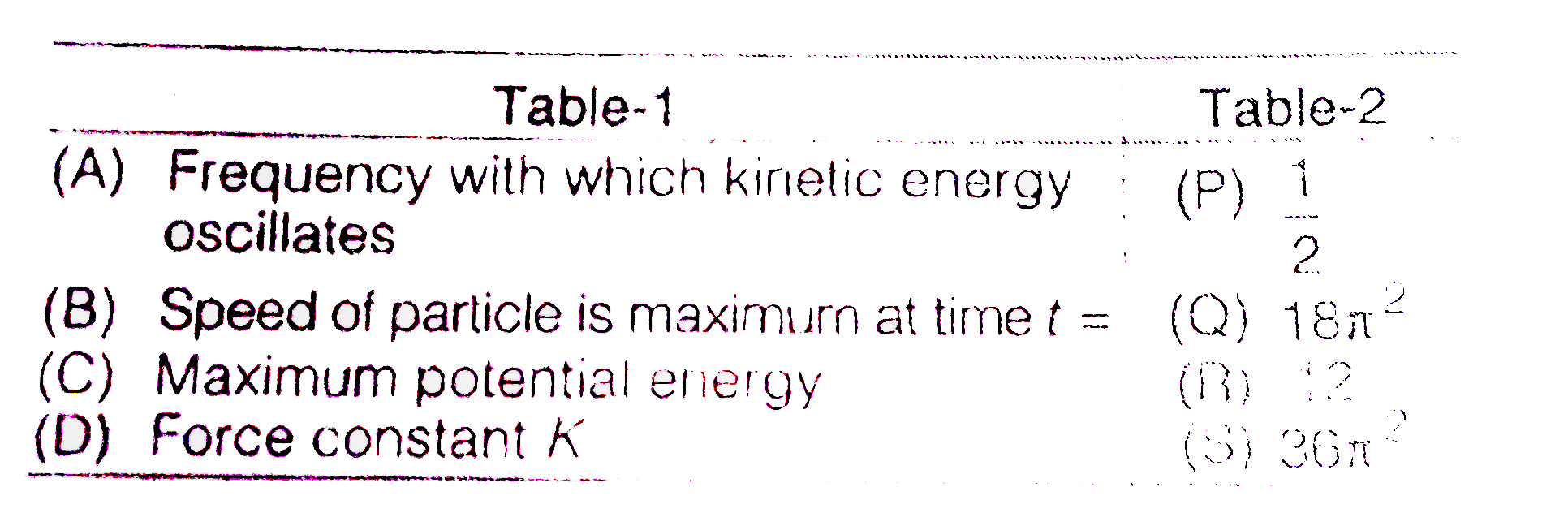
The correct Answer is:(A→r,B→p,C→q,D→s)
Step by step video solution for x-t equation of a particle in SHM is given as x=1.0sin(12pit) in SI units. Potential energy at mean position is zero. Mass of particle is 1/4 kg. Match the following table (SI units). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Assertion : x-t equation of a particle in SHM is given as :x=Acosωt
Reason : In the given equation the minimum potential energy is zero.
View SolutionIn SHM , potential energy of a particle at mean position is E1 and kinetic enregy is E2 , then
View SolutionThe time period of a particle in simple harmonic motion is T. Assume potential energy at mean position to be zero. After a time of T6 it passes its mean position ,then at t=0 its,
View SolutionDisplacement-time equation of a particle moving along x-axis is x=20+t3−12t (SI units)
(a) Find, position and velocity of particle at time t=0.
(b) State whether the motion is uniformly accelerated or not.
(c) Find position of particle when velocity of particle is zero.
View SolutionDisplacement-time equation of a particle moving along x-axis is x=20+t3−12t (SI units)
(a) Find, position and velocity of particle at time t=0.
(b) State whether the motion is uniformly accelerated or not.
(c) Find position of particle when velocity of particle is zero.
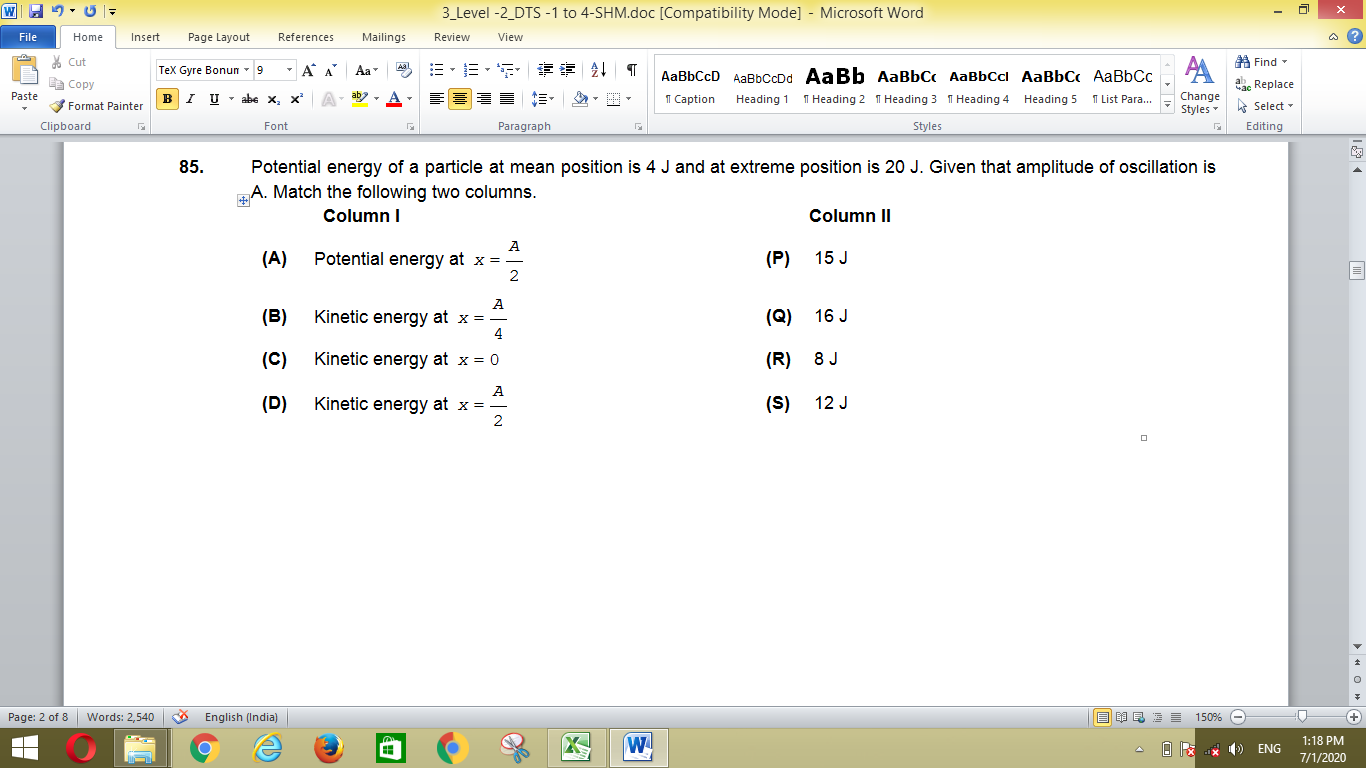
View SolutionPotential energy of a particle at mean position is 4 J and at extreme position is 20 J. Given that amplitude of oscillation is A. Match the following two columns
View SolutionThe kinetic energy of a particle executing shm is 32 J when it passes through the mean position. If the mass of the particle is.4 kg and the amplitude is one metre, find its time period.
View SolutionThe displacement of a particle of mass 3g executing simple harmonic motion is given by x=3sin(0.2t) in SI units. The kinetic energy of the particle at a point which is at a displacement equal to 13 of its amplitude from its mean position is
View SolutionVelocity-time equation of a particle moving in a straight line is, v=(10+2t+3t2) (SI units) Find
(a) displacement of particle from the mean position at time t=1s, if it is given that displacement is 20m at time t=0.
(b) acceleration-time equation.
View SolutionThe simple harmonic motion of a particle is given by x = a sin 2πt . Then, the location of the particle from its mean position at a time 1/8th of second is
View Solution
