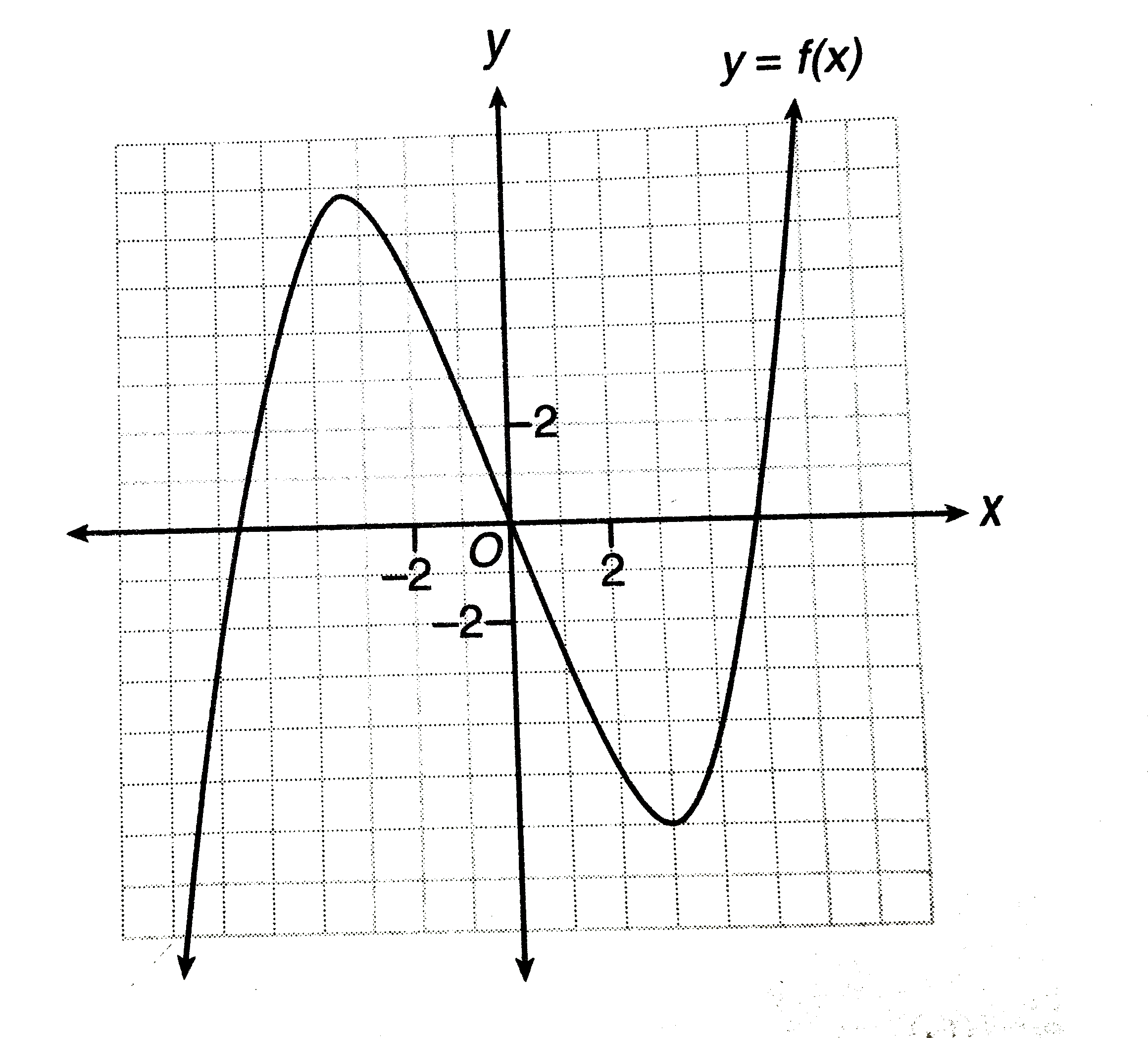
16-18 The graph of polynomial function f is shown above.
Q. What is the maximum number of points a circle whose center is at the origin can intersect the graph of y=f(x)?
A
2
B
3
C
4
D
6
Video Solution
Text Solution
The correct Answer is:D
|
Answer
Step by step video, text & image solution for 16-18 The graph of polynomial function f is shown above. Q. What is the maximum number of points a circle whose center is at the origin can intersect the graph of y=f(x)? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PASSPORT TO ADVANCED MATHBOOK - ENGLISH SATCHAPTER - PASSPORT TO ADVANCED MATHEXERCISE - Grib-In
29 Videos
- PASSPORT TO ADVANCED MATHBOOK - ENGLISH SATCHAPTER - PASSPORT TO ADVANCED MATHEXERCISE - EXERCISE
80 Videos
- PASSPORT TO ADVANCED MATHBOOK - ENGLISH SATCHAPTER - PASSPORT TO ADVANCED MATHEXERCISE - EXERCISE
80 Videos
- PARAMETRIC EQUATIONS BOOK - ENGLISH SATCHAPTER - PARAMETRIC EQUATIONS EXERCISE - EXERCISES
3 Videos
- PIECEWISE FUNCTIONSBOOK - ENGLISH SATCHAPTER - PIECEWISE FUNCTIONSEXERCISE - EXERCISES
8 Videos
Similar Questions
Knowledge Check
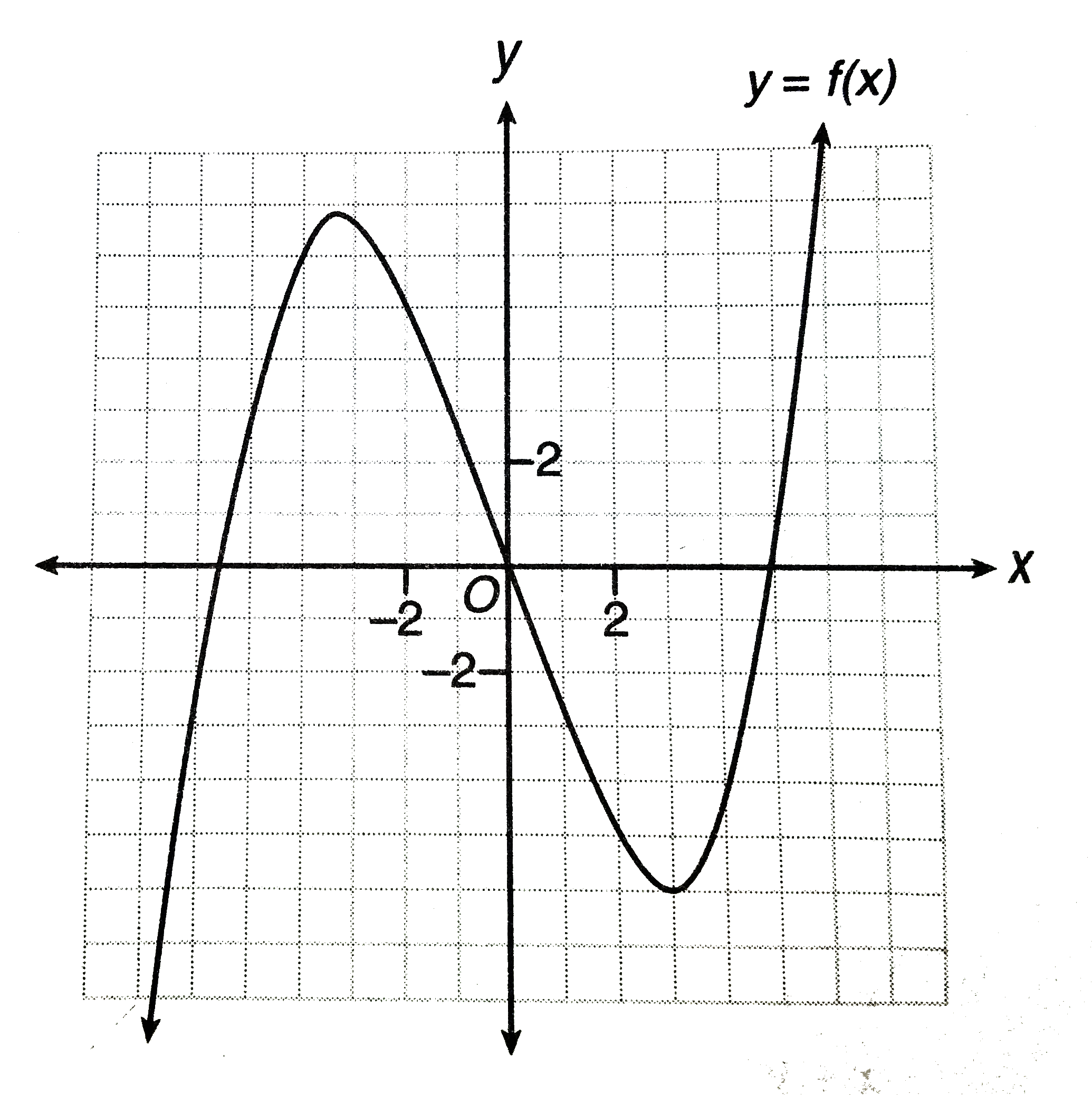
16-18 The graph of polynomial function f is shown above.
Q. What is the best estimate of the remainder when f(x) is divided by x+3?A−6.0B0C6.5DIt cannot be determined.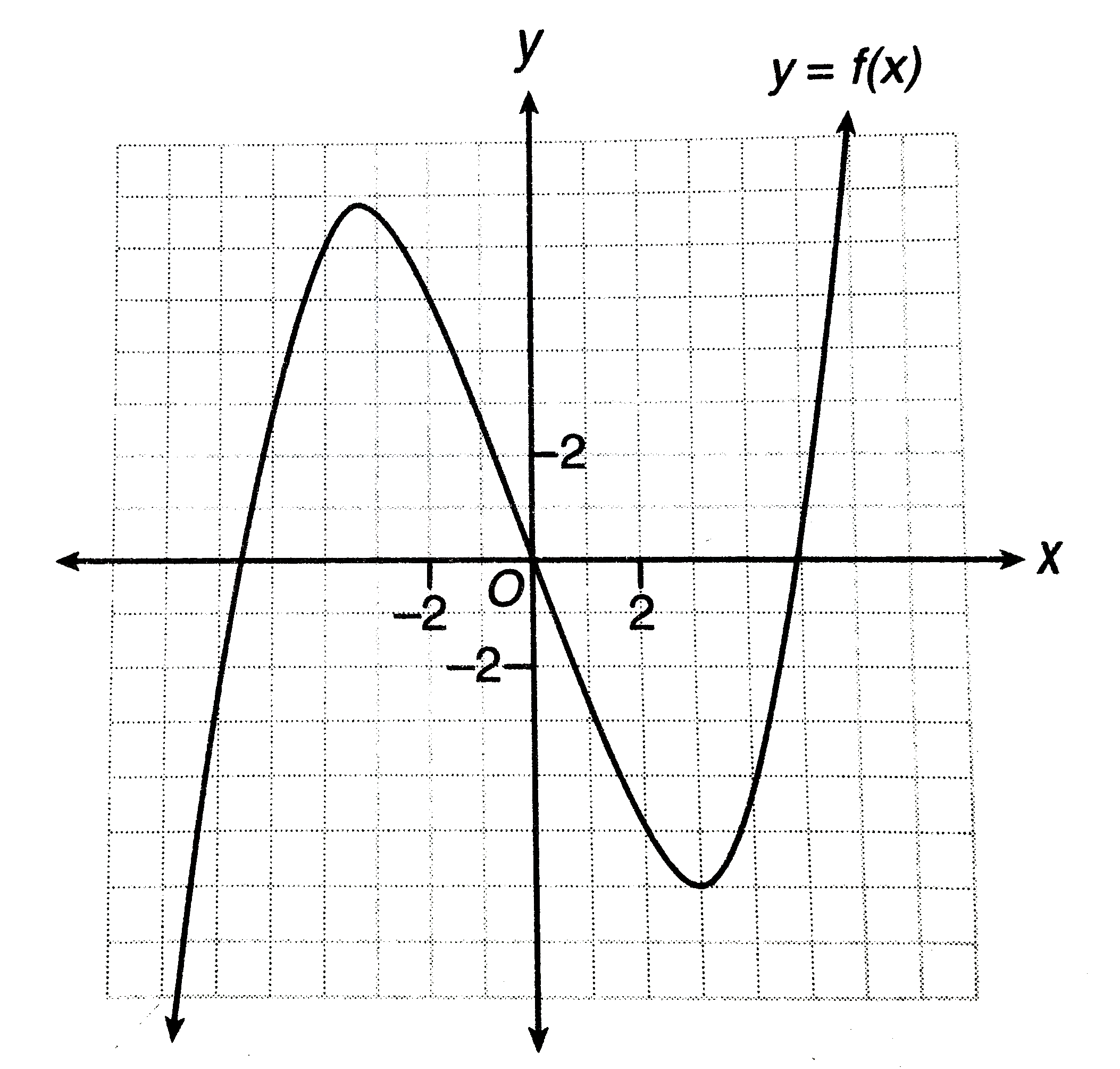
16-18 The graph of polynomial function f is shown above.
Q.What is the greatest integer value of k for which f(x)=k has exactly 3 real solutions?A−5B0C6D7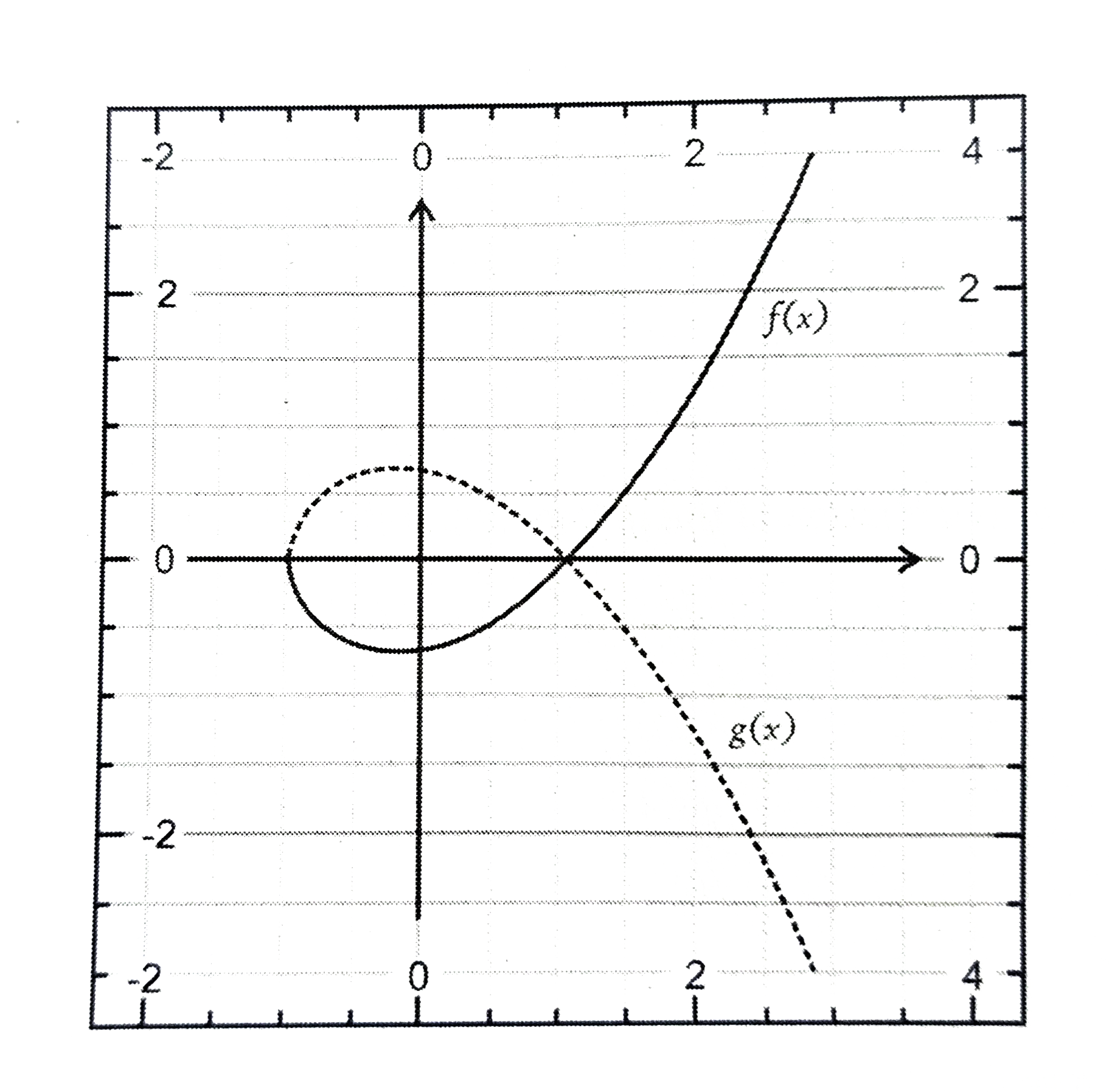
The graph of f(x) and g(x) are shown above . Which options is true ?Af(x)=g(−x)Bf(x)=g(x)−xCf(x)=−g(x)Df(x)=−g(−x)
The graph of y=f''(x) for a function f is shown.
Number of points of inflection for y=f(x) is….. .

View SolutionDraw the graph of the function f(x)=xx
View SolutionThe graph of a polynomial f(x) is as shown in Fig. 2.21. Write the number of real zeros of f(x) . (FIGURE)
View SolutionDraw the graph of the function f(x)=−x|x|.
View Solution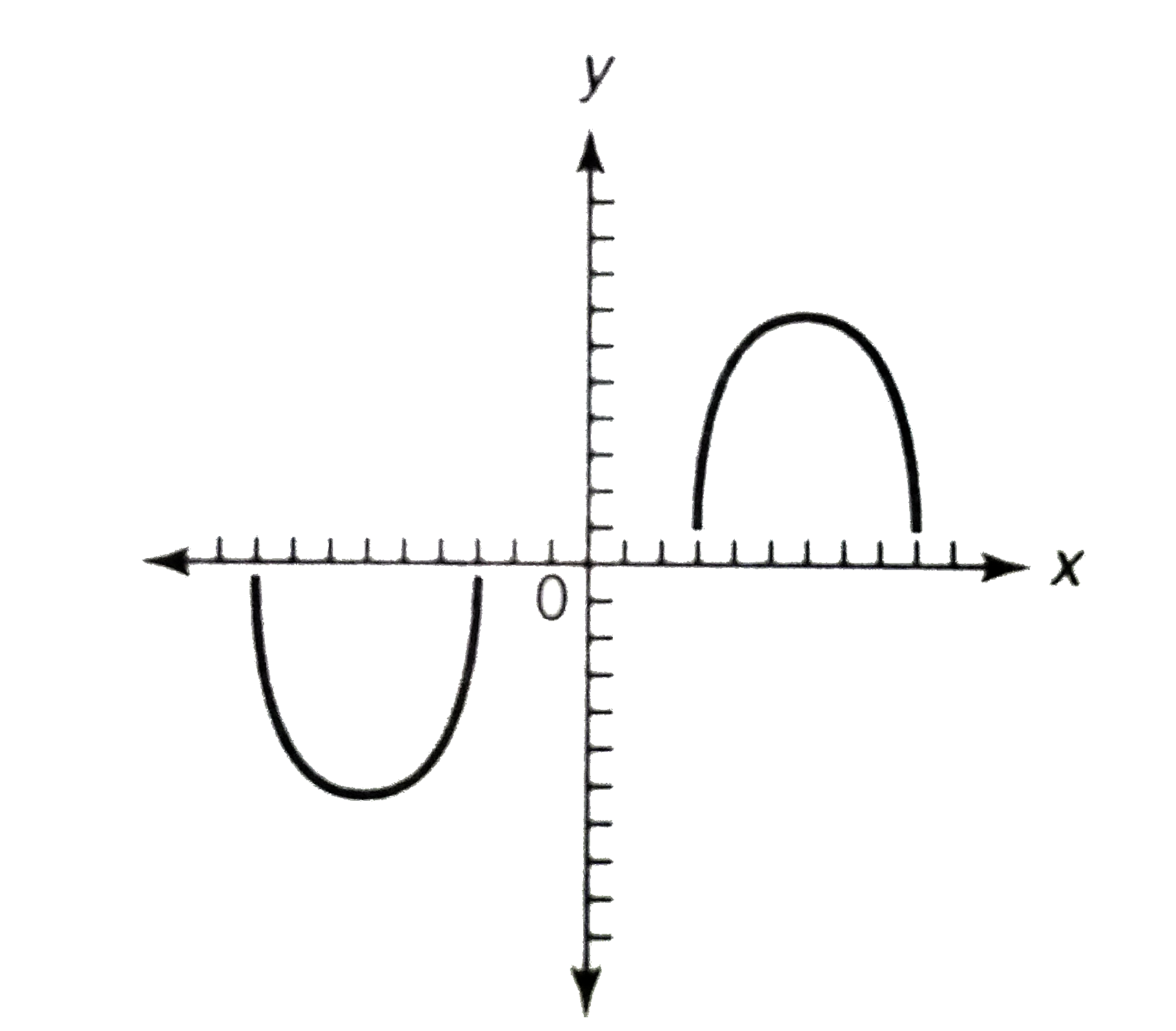
The graph of the function f is shown above. Which of the following could represent the graph of y=|f(x)|?
View Solution