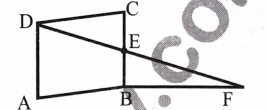
ABCD
is a parallelogram and E
is the mid-point of BC,DEandAB when produced meet at F
Then AF=
(a) 32AB
(b) 2AB
(c) 3AB
(d)54AB
△DCEandBFE,DEC=BEF(vertically opp any)
EC=BE(E is the mid point
∠DCB=∠EBF (alternate angle DC parallel to AF)
So△DCE=△BFE
DC=BF(1)
CD=AB (ABCD is a parallelogram
AF=AB+BF
(AB+DC) from (1)
=AB+AB=2AB
So option(b) is Correct.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If in a triangle AB=a,AC=b and D,E are the mid-points of AB and AC respectively, then DE is equal to
Aa4−b4Ba2−b2Cb4−a4Db2−a2 - Question 2 - Select One
If a parallelogram ABCD, |AB|=a,|AD|=band|AC|=C, then DA. AB is equal to
A12(a2+b2+c2)B12(a2−b2+c2)C14(a2+b2−c2)D13(b2+c2−a2)
In the given figure, ABCD is a parallelogram and E is the midpoint of side BC. If DE and AB when produced meet at F, prove that AF = 2AB.
 View Solution
View SolutionIn the given figure, ABCD is a parallelogram, in which is the mid-point of ADandO is a point on AC such that AO=14AC. When EO produced, meets AB at F. Prove that F is the mid-point of AB.
View SolutionIf A and B are two matrices such that AB=BandBA=A then A2+B2= (A) 2AB (B) 2BA (C) A+B (D) AB
View SolutionIf a and b are position vectors of A and B respectively the position vector of a point C on AB produced such that −−→AC=3−−→AB is
View SolutionIn figure, ABC is a triangle with ∠B=90∘, BC = 3cm and AB = 4cm. D is a point on AC such that AD = 1cm and E is the mid- point of AB. Join D and E and extend DE to meet CB extended at F. Then BF is equal to
View SolutionABC is an isosceles triangle in which AB=AC. If DandE are the mid-points of ABandAC respectively, prove that the points B,C,DandE are concylic.
View Solution