is a rhombus. If find
To solve the problem, we need to find the angle ∠ADB in the rhombus ABCD given that ∠ACB=40∘.
1. Understand the Properties of a Rhombus:
- In a rhombus, the diagonals bisect each other at right angles. This means that ∠AOB=90∘ where O is the intersection point of the diagonals AC and BD.
2. Identify the Angles in Triangle ACB:
- We know that ∠ACB=40∘.
- Since O is the intersection of the diagonals, ∠AOB=90∘.
3. Calculate ∠CBO:
- In triangle COB, we can find ∠CBO using the fact that the sum of angles in a triangle is 180∘.
- Therefore, we have:
∠CBO=180∘−∠ACB−∠AOB
∠CBO=180∘−40∘−90∘=50∘
4. Relate ∠ADB to ∠CBO:
- Since AD is parallel to CB (as opposite sides of a rhombus), ∠ADB is equal to ∠CBO by the Alternate Interior Angles Theorem.
- Thus, we have:
∠ADB=∠CBO=50∘
5. Final Answer:
- Therefore, the value of ∠ADB is 50∘.
Summary:
The angle ∠ADB in rhombus ABCD is 50∘.
- In a rhombus, the diagonals bisect each other at right angles. This means that ∠AOB=90∘ where O is the intersection point of the diagonals AC and BD.
- We know that ∠ACB=40∘.
- Since O is the intersection of the diagonals, ∠AOB=90∘.
- In triangle COB, we can find ∠CBO using the fact that the sum of angles in a triangle is 180∘.
- Therefore, we have:
∠CBO=180∘−∠ACB−∠AOB
∠CBO=180∘−40∘−90∘=50∘
- Since AD is parallel to CB (as opposite sides of a rhombus), ∠ADB is equal to ∠CBO by the Alternate Interior Angles Theorem.
- Thus, we have:
∠ADB=∠CBO=50∘
- Therefore, the value of ∠ADB is 50∘.
The angle ∠ADB in rhombus ABCD is 50∘.
Topper's Solved these Questions
Similar Questions
If ABCD is a rhombus with ∠ABC=56∘, find the measure of ∠ACD
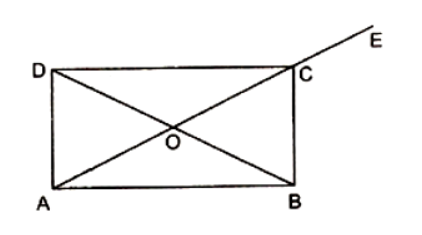
View SolutionIn Figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD=1460, find ∠AOB
View SolutionIn a rhombus ABCD, if ∠ACB=40∘, then ∠ADB=(a) 70∘ (b) 45∘ (c) 50∘ (d) 60∘
View SolutionIn Fig.87, the corresponding arms of ∠ABC and ∠DEF are parallel. If ∠ABC=750, find the ∠DEF.
View SolutionIn Fig. 6.13, lines AB and CD intersect at O. If ∠AOC +∠BOE=70∘and ∠BOD=40∘, find ∠BOE and reflex ∠COE.
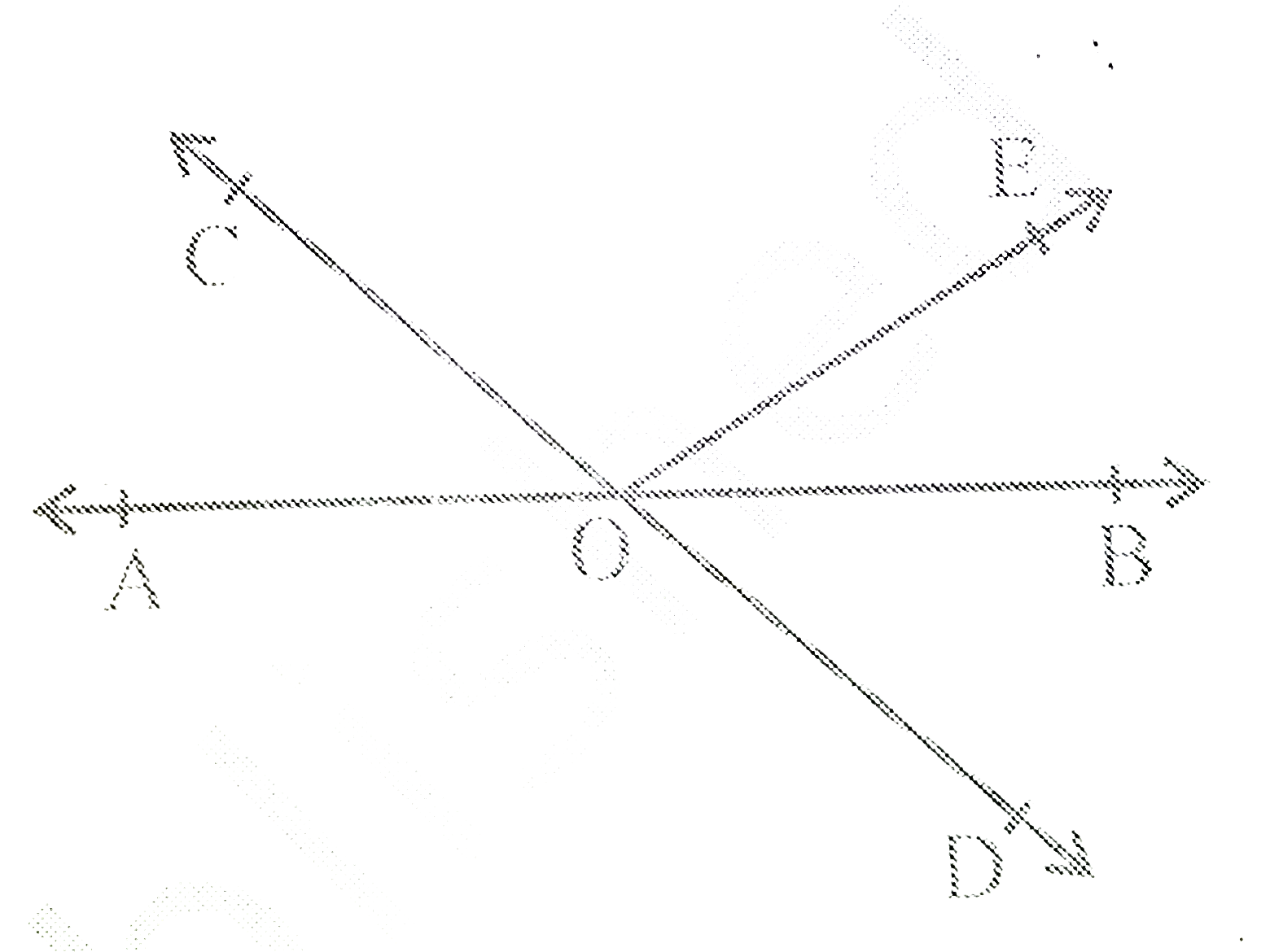
View SolutionABCD is a cyclic quadrilateral in which: BCAD, ∠ADC=1100 and ∠BAC=500. Find ∠DAC ∠DBC=800 and ∠BAC=400. Find ∠BCD ∠BCD=1000and ∠ABD=700 find ∠ADB
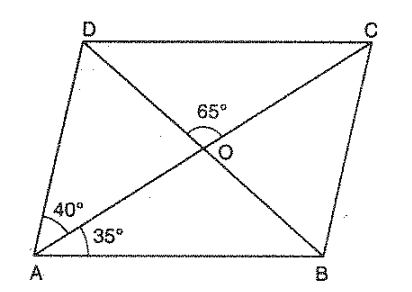
View SolutionIn Figure, ABCD is a parallelogram in which ∠DAO=400, ∠BAO=350 and ∠COD=650. Find: ∠ABO (ii) ∠ODC (iii) ∠ACB (iv) ∠CBD
View SolutionIn Fig.81, side BC of ABC has been produced to D and CEBA. If ∠ABC=650, ∠BAC=550, find ∠ACE, ∠ECD and ∠ACD.
View SolutionIn a ABC, if ∠A=550, ∠B=400, find ∠C
View SolutionIn fig. 15 ABCD is a parallelogram CM⊥AB and BL⊥AD. If AB=16 cm, AD=12 cm, CM=10 cm, find BL If AD=10cm, CM=8cm, BL=12 cm, find AB .
View Solution
