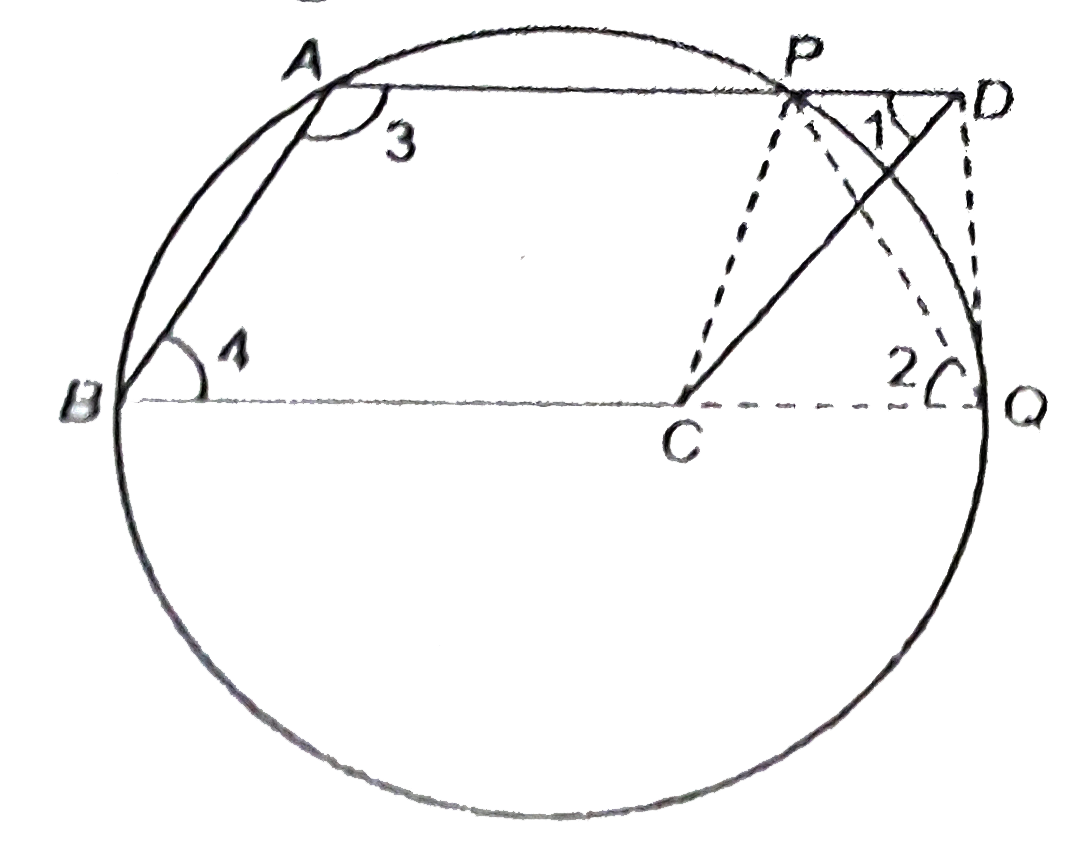
A circle intersects the side AD of a parallelogram ABCD at P and BC produced at Q. Prove that square PDQC is cyclic .
∵AB| |DC
∴∠1+∠3=180∘ (sum of co-interior angles is 180∘) ....(1)
and ∠3+∠2=180∘
(sum of opposite angles of a cyclic quadrilateral )....(2)
∴ From eqs. (1) and (2) , we get
∠1+∠3=∠3+∠2
⇒∠1=∠2
But these are the angles subtended by line segment joining two points P and C lying on the same side of PC.
So, square PDQC is cyclic.
Topper's Solved these Questions
- CIRCLE BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise 10a
22 Videos
- CIRCLE BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise 10b
19 Videos
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Revision Exercise (long Answer Question)
5 Videos
- CO-ORDINATE GEOMETRY BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRY EXERCISE - Exercise
8 Videos
Similar Questions
A point E is taken on the side BC of a parallelogram ABCD. AE and DC are produced to meet at F. Prove that ar(ΔADF)=ar(ABFC).
View SolutionP is a point on side BC of a parallelogram ABCD. If DP produced meets AB produced at point L, prove that :
DP:PL=DC:BL.
View SolutionP is a point on side BC of a parallelogram ABCD. If DP produced meets AB produced at point L, prove that :
DL:DP=AL:DC
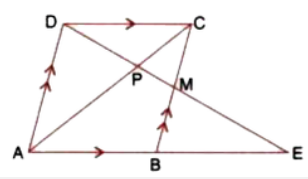
View SolutionIn the following figure, M is mid-point of BC of a parallelogram ABCD. DM intersects the diagonal AC at P and AB produced at E. Prove that : PE = 2 PD .
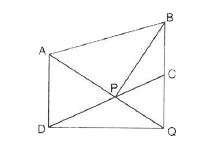
View SolutionABCD is a parallelogram, a line through A cuts DC at point P and BC produced at Q.
Prove that triangle BCP is equal in area to triangle DPQ.
View SolutionABCD is a parallelogram, a line through A cuts DC at point P and BC produced at Q.
Prove that triangle BCP is equal in area to triangle DPQ.

View SolutionE si a point on side AD produced of a parallelogram ABCD and BE intersects DC at F. prove that △ABE~△CFB.
View SolutionE is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that ΔABE~ΔCFB.
View SolutionE is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that ΔABE~ΔCFB .
View SolutionIf E is a point on side AD produced of a parallelogram ABCD and BE intersects CD at F , Prove that △ABE~△CFB .
View Solution
