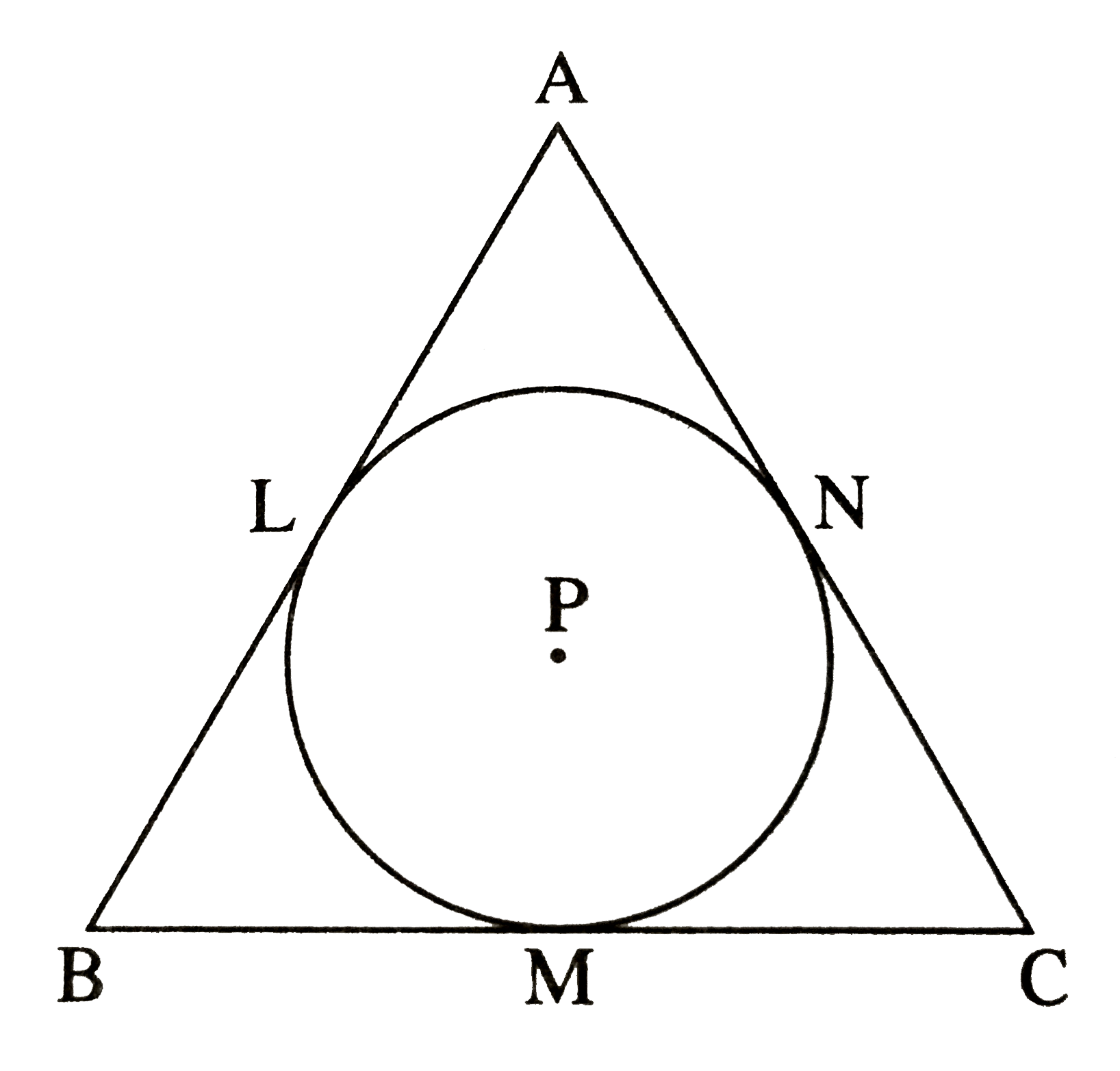
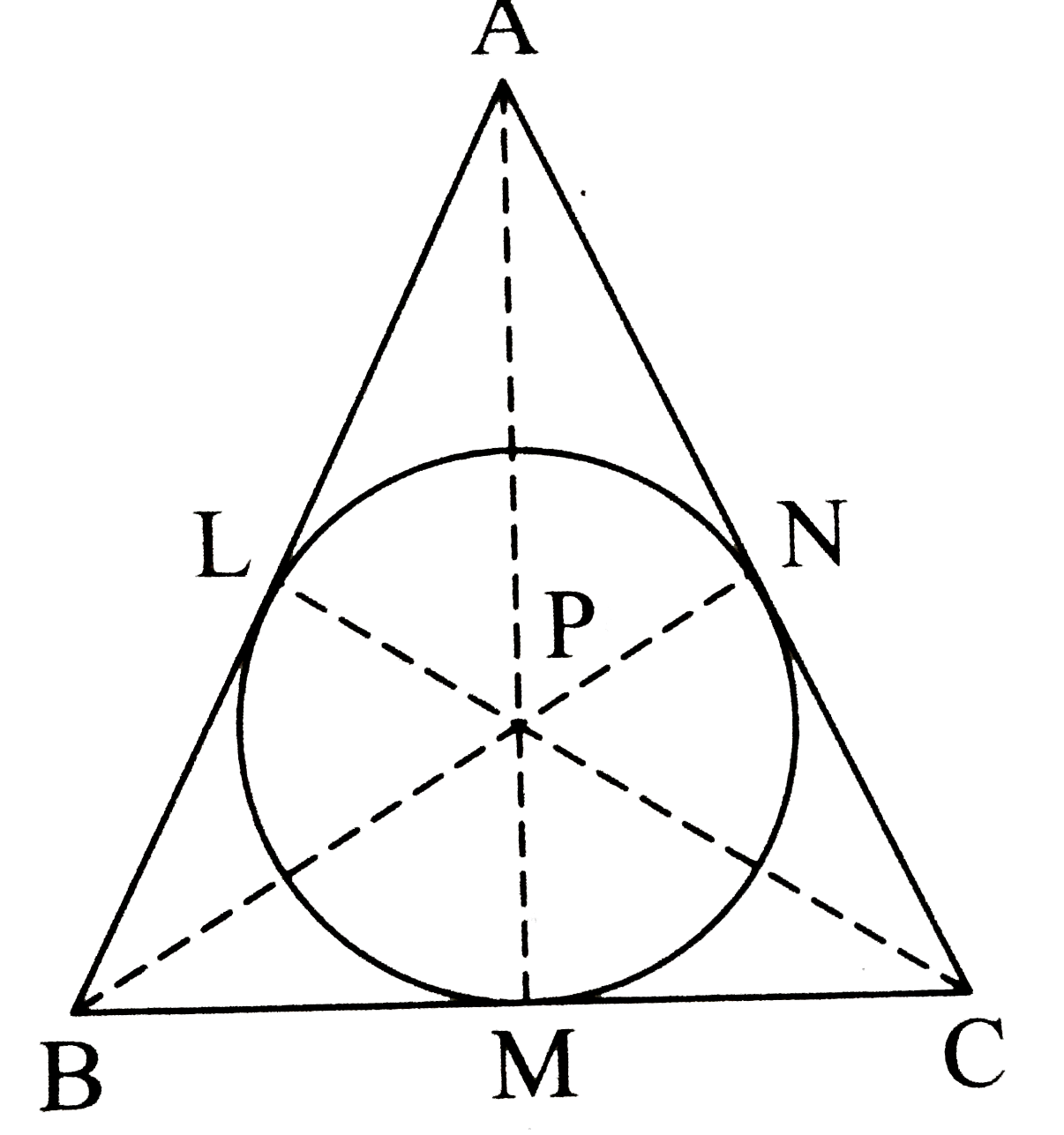
A circle with centre P is inscribed in the triangle ABC. Side AB, side

A circle is inscribed in a triangle ABC. It touches the sides AB, BC and AC at the points R, P and Q respectively. If AQ = 4.5 cm, PC = 5.5 cm and BR = 6 cm, then the perimeter of the triangle ABC is:
एक वृत्त किसी त्रिभुज ABC में अन्तर्निहित है | यह भुजाओं AB, BC और AC को क्रमशः बिंदु R, P और Q पर स्पर्श करता है | यदि AQ = 4.5 सेमी, PC = 5.5 सेमी और BR = 6 सेमी है, तो त्रिभुज ABC का परिमाप ज्ञात करें |
A $30.5 c m$ .
B $28 c m$ .
C $32 c m$ .
D $26.5 c m$ .
$Δ A B C$ is an isosceles triangle and AB = AC. If all the sides AB. BC and CA of a $Δ A B C$ touch a circle at D, E and F respectively. Then BE is equal to :
A $\frac{1}{2}$ BC
B $\frac{1}{3}$ BC
C $\frac{1}{4}$ BC
D $\frac{2}{3}$ BC

A circle with centre P is inscribed in the $△$ ABC. Side AB, side BC and side AC touch the circle at points L,M and N respectively. Radius of the circle is r. Prove that: $A (△ A B C) = \frac{1}{2} (A B + B C + A C) \times r$ .