A curve is given by byy={(√4−x2),0≤x<1√(3x),1≤x≤3. Find the area lying between the curve and x-axis.
Video Solution
Text Solution
The correct Answer is:16(2π−√3+36) sq. units
The given curve is
y={√(4−x2),0≤x<1√(3x),1≤x≤3
Obviously, the curve is the are of the circle x2+y2=4 (1)
between 0≤x<1 and the are of parabola y2=3x (2)
between 1≤x≤3
Required area = shaded area
=Area OABCO+Area CBDEC
=∣∣∣∫10√(4−x2)dx∣∣∣+∣∣∣∫31√3xdx∣∣∣
∣∣∣[12x√(4−x2)+42sin−1(x2)]10∣∣∣+∣∣∣√3[23x3/2]31∣∣∣
=(√32+π3)+23(9−√3)
=16(2π−√3+36) sq. units.
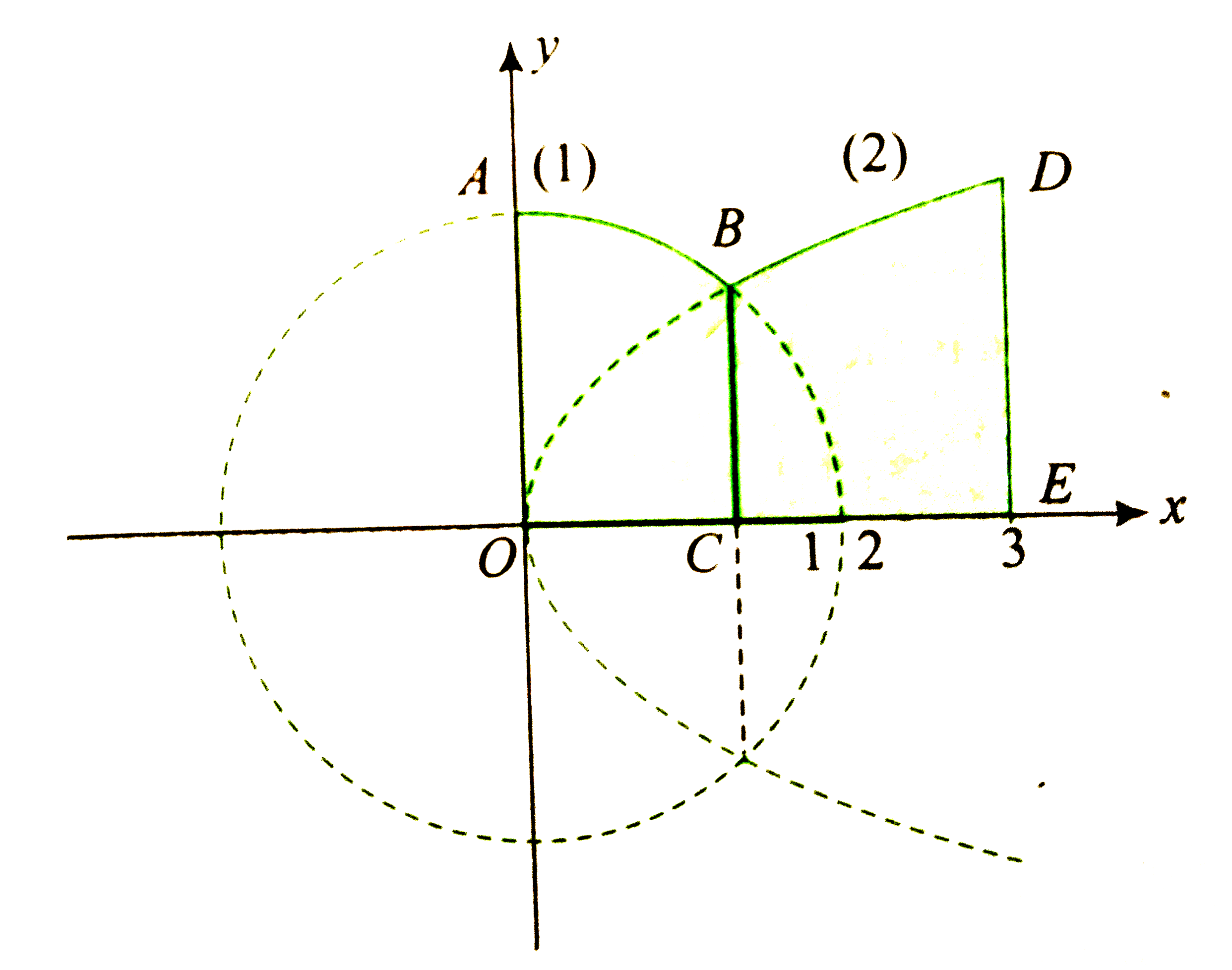
|
Topper's Solved these Questions
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.2
14 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.3
7 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Comprehension Type
2 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Question Bank
29 Videos
- AREA UNDER CURVESBOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
20 Videos
Similar Questions
Knowledge Check
Area under the curve y=√16−x2 between x = 0 and x = 4 in the first quadrant is
A8πB16πC4πD8π3The angle between the curves y=sinxandy=cosx,0<x<x2, is
Atan−1(2√2)Btan−1(3√2)Ctan−1(3√3)Dtan−1(5√2)If f(x) is continuous on [-2, 2], where f(x)=⎧⎪ ⎪⎨⎪ ⎪⎩sinaxx+2, for −2≤x<03x+5, for 0≤x≤1√x2+8−b, for 1<x<2, then a+b=
A-15B0C2D-2
D={x:0≤4x2≤1}
View SolutionThe points of discontinuity of the function f(x)={2√x , 0≤x≤1, 4−2x , 1<x
View SolutionVerify Rolle's theorem for the following functions
(i) f(x)=√4−x2 in [−2,2]
(ii) f(x)=log(x2+ab(a+b)x] in [a,b], where 0<a<b
View SolutionProve that sin−1.((x+√1−x2√2)=sin−1x+π4, where −1√2<x<1√2
View SolutionArea of the region bounded by the curve
y={x2,x<0x,x≥0 and the line y=4 is
View Solution