A lamp post standing at a point A on a circular path of radius r subtends an angle α at some point B on the path, and AB subtends an angle of 45∘ at any other point on the path, then height of the lampost is
√2rcotα
(r/√2)tanα
√2rtanα
(r/√2)cotα
The correct Answer is:C
Step by step video, text & image solution for A lamp post standing at a point A on a circular path of radius r subtends an angle alpha at some point B on the path, and AB subtends an angle of 45^(@) at any other point on the path, then height of the lampost is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- HEIGHTS AND DISTANCES BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEIGHTS AND DISTANCES EXERCISE - SOLVED EXAMPLES (LEVEL 2 ( SINGLE CORRECT ANSWER TYPE QUESTIONS ))
15 Videos
- HEIGHTS AND DISTANCES BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEIGHTS AND DISTANCES EXERCISE - SOLVED EXAMPLES (NUMERICAL ANSWER TYPE QUESTIONS )
5 Videos
- HEIGHTS AND DISTANCES BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEIGHTS AND DISTANCES EXERCISE - QUESTIONS FROM PREVIOUS YEARS. B-ARCHITECTURE ENTRANCE EXAMINATION PAPERS
3 Videos
- ELLIPSEBOOK - MCGROW HILL PUBLICATIONCHAPTER - ELLIPSEEXERCISE - Previous Years B-Architecture Entrance Examination Papers
6 Videos
- HYPERBOLABOOK - MCGROW HILL PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - QUESTION FROM PREVIOUS YEARS. B-ARCHITECTURE ENTRANCE EXAMINATION PAPERS
8 Videos
Similar Questions
Find the equation of the circle in which the chord joining the points (a,b)and(b,−a) subtends an angle of 45∘ at any point on the circumference of the circle.
View SolutionA lamp post is situated at the middle point M of the side AC of a triangular plot of ABC with BC=7m, CA=8 and AB=9m. Lamp post subtends an angle of 150 at the point B. determine the height of the lamp post.
View Solution
Knowledge Check
A pole stands at a point A on the boundary of a circular park of radius a and subtends an angle α at another point B on the boundary. If the chord AB subtends an angle α at the centre of the path, the height of the pole is
A2acos(α/2)tanαB2asin(α/2)cotαC2asin(α/2)tanαD2acos(α/2)cotαA vertical pole stands at a point A on the boundary of a circular park of radius a and subtends an angle α at another point β on the boundary. If the chord AB subtends an angle α at the centre of the park, the height of the pole is
A2a sinα2tanαB2a cosα2tanαC2a sinα2cotαD2a cosα2cotαA vertical pole stands at a point A on the boundary of a circular park of radius 2 km and subtends an angle 60∘ at another point B on the boundary. If the chord AB subtends the same angle 60∘ at the centre of the park, the height of the pole is
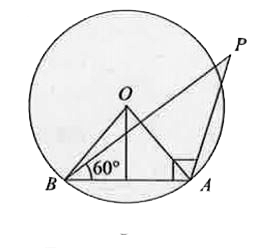 A2√3 kmB√3 kmC2/√3 kmmD1 km
A2√3 kmB√3 kmC2/√3 kmmD1 km
A round balloon of radius R subtend an angle 2α at the eye of the observer, while the angle of elevation of it's centre is 2β, then the height of the centre of balloon is
View SolutionA lamppost stands in the centre of a circular garden and makes angle α at point A and B on the boundary where AB subtends an angle 2β at the foot of the lamppost. If γ is the angle which the lamppost subtends at C, the middle point of the line joining A and B, then tanγ =
View SolutionA vertical tower subtends an angle of 60∘ at a point on the same level as the foot of the tower. On moving 100 m further in line with the tower, it subtends an angle of 30∘ at the point. Then, the height of the tower, it subtends an angle of 30∘ at the point. Then, the height of the tower is
View SolutionAn equation chord joining the points (1,2)and(2,−1) subtends an angle of π/4 at any point on the circumference is
View SolutionIn a triangular plot ABC with BC = 7 m, CA = 8 m and AB = 9 m. A lamp post is situated at the midle point E of the side AC and subtends an angle tan−13 at the point B, the height of the lamp post is
View Solution
