A positive integer N is selected so as to be 100<N<200. Then, the probability that it is divisible by 4 or 7, is Solution in Punjabi
733
1733
3299
3499
The correct Answer is:(d)
Step by step video solution for A positive integer N is selected so as to be 100ltNlt200. Then, the probability that it is divisible by 4 or 7, is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Probability Exercise 1: Single Option Single Correct Type Question
1 Videos
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise (More Than One Correct Option Type Questions)
15 Videos
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise For Session 4
15 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
28 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
52 Videos
Similar Questions
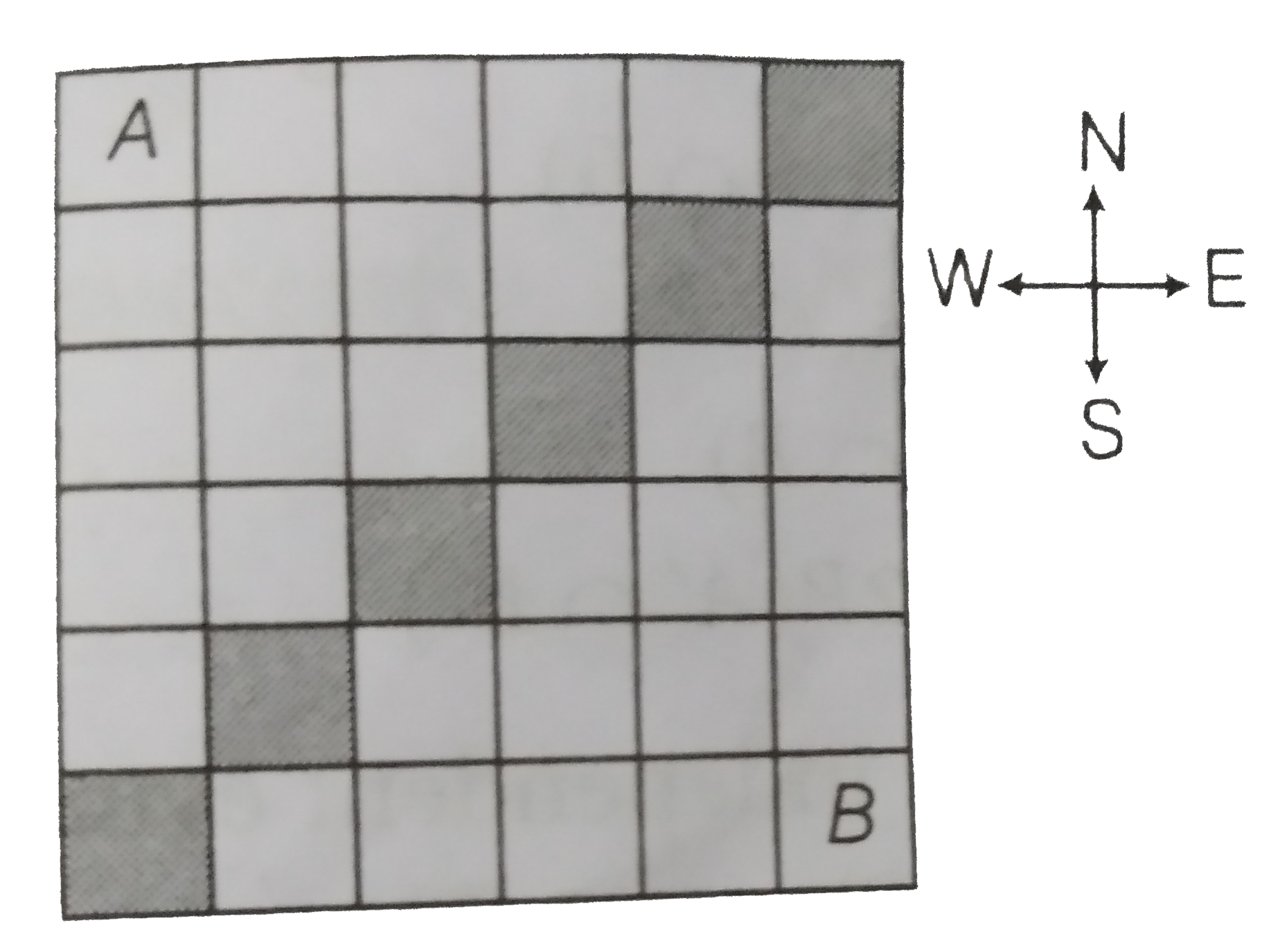
A and B are persons standing in corner square as shown in the figure. They start to move on same time with equal speed, of A can move in East or South diretion and B can move in North or West diretion. If in each step they reach in next square and their choice of direction are equlity. If it is given that A and B meet in shaded region, then the probability that they have met in the top most shaded square, is
View SolutionFor two given event A and B, P(A∩B) is
View SolutionThe probability of getting exactly two heads when tossing a coin three times is
View SolutionThere is a rectangular sheet of dimension (2m−1)×(2n−1), (where m>0,n>0) It has been divided into square of unit area by drawing line perpendicular to the sides. Find the number of rectangles having sides of odd unit length.
View SolutionLet O be the origin and let PQR be an arbitrary triangle. The point S is such that ¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OQ+¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OP+¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OR+¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OS Then the triangle PQR has S as its
View SolutionFor every positive integer n, prove that 7n−3nis divisible by 4.
View SolutionIf n is a positive integer, show that: 4n−3n−1 is divisible by 9
View SolutionIf n is positive integer show that 9n+7 is divisible 8
View SolutionIf the four positive integers are selected randomly from the set of positive integers, then the probability that the number 1,3, 7 and 9 are in the unit place in the product of 4 - digit, so selected is
View SolutionIf n is a positive integer, then (n+4)!(n+7)!=
View Solutionयदि पूर्णांक m और n को यादृच्छिक रूप से (At random) 1 और 100 के बीच से चुना जाता है, तो संख्या 7m+7n के 5 से विभाजित होने की प्रायिकता है
View SolutionFor every positive integer n, prove that 7n–3n is divisible by 4.
View SolutionFor every positive integer n, prove that 7n–3n is divisible by 4.
View SolutionFor every positive integer n, prove that 7n–3n is divisible by 4.
View SolutionFor every positive integer n, prove that 7n−3nis divisible by 4.
View SolutionFor every positive integer n, prove that 7n−3n is divisible by 4.
View Solution
