A toy manufacturer produces two types of dolls; a basic version dill A and a deluxe version doll B. Each doll of type B takes twice as long to produce as one doll of type A. The company have time to make a maximum of 2000 dolls of type A per day, the supply of plastic is sufficient to produce 1500 dolls per day and each type requires equal amount of it. The deluxe version i.e. type B requires a fancy dress of which there are only 600 per day available. If the company makes a profit of RLs. 3 and Rs. 5 per doll, respectively, on doll A and B; how many of each should be produced per day in order to maximize profit? Solve it by graphical method.
het Amit solves correctly
from first st=x.
Frow second scta fork third set =2.
Frow third set=2.
x+y+2≤10>.
Total credit points =5x+4y+6z.
Z=5x+4y+6z
Total time required by Amit =3x+2y+4z.
Total time Amit has =312 hours =210{mins}.
⇒3x+2y+4z≤210
Aho, 3x+2y≤150+
Number of poblens solved cannot be negative ⇒x,y,z≥0.
Therefore, the LPP is :-
Maximisk z=5x+4y+6z subject to x+y+z
Subject to x+y+z≤10203x+2y+4z≤210
3x+2y+4z≤210
3x+2y≤150
x,y,z≥0
Similar Questions
A toy company manufactures two types of doll; a basic version doll; a basic version doll A and a deluxe version doll B. Each doll of type B takes twice as long to produce as one of type. A and the company would have time to ,make a maximum of 2, 000 per day if it produces only the basic version. The supply of plastic is sufficient to produce 1500 dolls per day (both A and B combined). The deluxe version requires a fancy dress of which there are only 600 per day available. If the company makes profit Rs. 3 and Rs. 5 per doll respectively o doll A and doll B; how many of each should be produced per day in order to maximize profit?
View SolutionA toy company manufactures two types of doll; a basic version doll; a basic version doll A and a deluxe version doll B. Each doll of type B takes twice as long to produce as one of type. A and the company would have time to ,make a maximum of 2, 000 per day if it produces only the basic version. The supply of plastic is sufficient to produce 1500 dolls per day (both A and B combined). The deluxe version requires a fancy dress of which there are only 600 per day available. If the company makes profit Rs. 3 and Rs. 5 per doll respectively o doll A and doll B; how many of each should be produced per day in order to maximize profit?
View SolutionA toy company manufacutes two types of dolls, A and B. Each doll of type B takes twice as long to produce as one of type. A and the company would have time to make a maxium of 2000 prr day, if it produces only type.A the supply of plastic is sufficient to produce 1500 dolls per day (both A and B combined). Type B requires a fancy dress of which there are only 600 per day available. If the company makes profits of Rs.3 and Rs5 per doll respectively on dolls A and B, how many of each should be produced per day in order to mazimize the profit? Also, find the maximum profit.
View SolutionA toy company manufactures two types of dolls A and B. Market research and available resources have indicated that the combined production level should not exceed 1200 dolls per week and the demand for dolls of type B is at most half of that for dolls of type A. Further the production level of dolls of type A can exceed three times the production of dolls of other type by at most 600 units. If the company makes profit of Rs. 12 and Rs. 16 per doll respectively on dolls A and B how many of each should be produced weekly in order to maximise the profit?
View SolutionA toy company manufactures two types of dolls, A and B. Market tests and available resources have indicated that the combined production level should not exceed 1200 dolls per week and the demand for dolls of type B is at most half of that for dolls of type A. Further, the production level of dolls of type A can exceed three times the production of dolls of other type by at most 600 units. If the company makes profit of Rs 12 and Rs 16 per doll respectively on dolls A and B. how many of each should be produced weekly in order to maximise the profit?
View SolutionA company produces two types of leather belts A and B on which the profits are Rs. 40 and Rs. 30 per belt respectively. Each belt of type A requires twice as much time as required by a belt to type B.If all belts were of type B the company could produce 1000 belts per day. But the supply of leather is sufficient only for 800 belts per day. Belt A requires a fancy buckle and only 400 fancy buckles are available per day. For belt of type B only 700 buckles are available per day. How should the company manufacture the two types of belts in order to have maximum overall profit?
View SolutionA company produces two types of belts A and B. Profits on these belts are Rs. 2 and Rs. 1.50 per belt respectively. A belt of type A requires twice as much time as belt of type B. The company can produce at most 1000 belts of type B per day. Material for 800 belts per day is available. At most 400 buckles for belts of type A and 700 for type B are available per day. How much belts of each type should the company produce so as to maximise the profit?
View SolutionA company makes two kinds of leather belts, A and B. Belt A is high quality belt, and B is of lower quality. The respective profits are Rs. 40 and Rs. 30 per belt. Each belt of type A requires twice as much time as a belt of type B, and if all belts were of type B, the company could make 1000 belts per day. The supply of leather is sufficient for only 800 belts per day (both A and B combined). Belt A requires a fancy buckle, and only 400 buckles per day are available. There are only 700 buckles available for belt B. What should be the daily production of each type f belt? Formulate the problem as a LPP.
View SolutionA company makes two kinds of leather belts, A and B. Belt A is high quality belt, and B is of lower quality. The respective profits are Rs. 40 and Rs. 30 per belt. Each belt of type A requires twice as much time as a belt of type B, and if all belts were of type B, the company could make 1000 belts per day. The supply of leather is sufficient for only 800 belts per day (both A and B combined). Belt A requires a fancy buckle, and only 400 buckles per day are available. There are only 700 buckles available for belt B. What should be the daily production of each type f belt? Formulate the problem as a LPP.
View SolutionA manufacturer makes two types of toys A and B. Three machines are needed for this purpose and the tune (in minutes) required for each toy on the machines is given below:
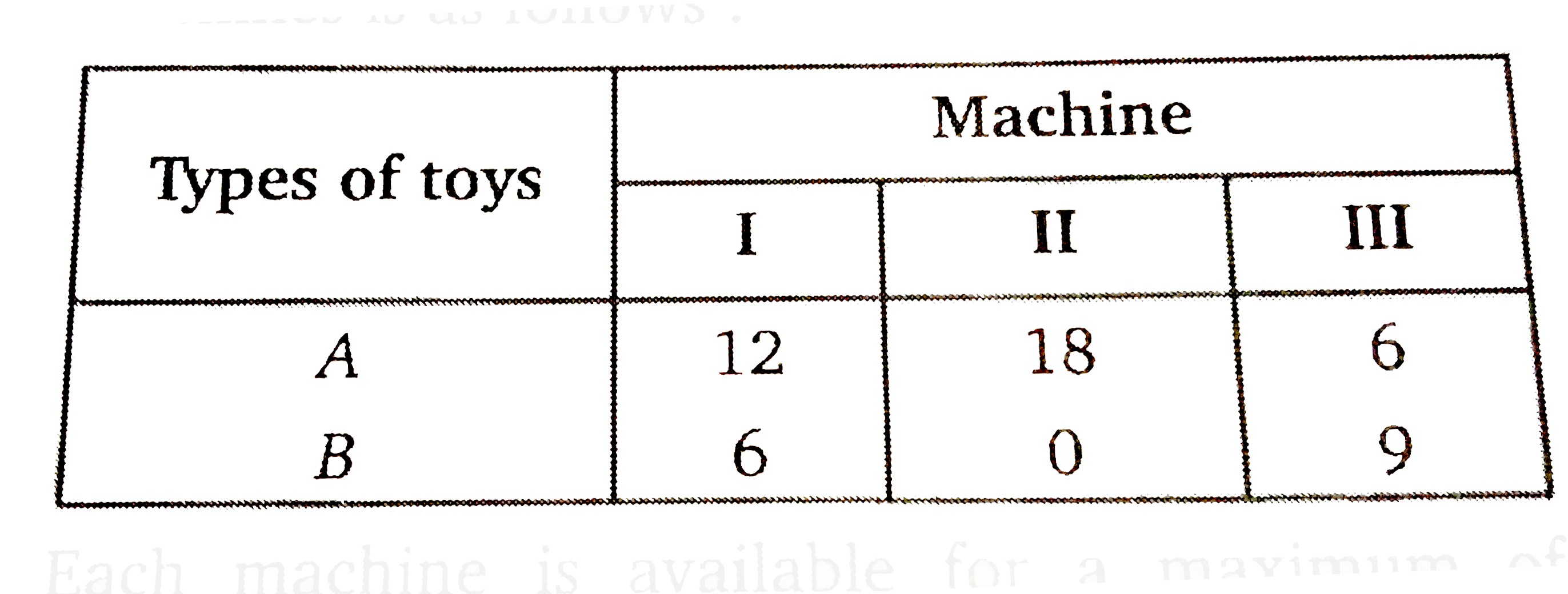 Each machine is available for a
maximum of 6 hours per day. If the profit View Solution
Each machine is available for a
maximum of 6 hours per day. If the profit View Solution
