AB and CD are two parallel chords of a circle which are on opposite sides of the centre such that AB =10cm , CD =24 cm and the distance between AB and CD is 17cm. Find the radius of the circle. Solution in Tamil
The correct Answer is:x2+144
Step by step video, text & image solution for AB and CD are two parallel chords of a circle which are on opposite sides of the centre such that AB =10cm , CD =24 cm and the distance between AB and CD is 17cm. Find the radius of the circle. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - Assignment (I. Choose the correct answer)
5 Videos
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - Assignment (II Match the following)
1 Videos
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - Additional Questions I. Multiple Choice Question.
20 Videos
- COORDINATE GEOMETRYBOOK - FULL MARKSCHAPTER - COORDINATE GEOMETRYEXERCISE - TEXTBOOK ACTIVITY
2 Videos
- MENSURATION BOOK - FULL MARKSCHAPTER - MENSURATION EXERCISE - Assignment (II. Answer the following questions)
8 Videos
Similar Questions
ABCD is a cyclic quadrilateral. Given that ∠ADB+∠DAB=120∘ and ∠ABC+∠BDA=145∘. Find the value of ∠CDB.
View SolutionMatch the following

View SolutionThe angle sum of a convex polygon with number of sides 7 is . . . . . . . . .
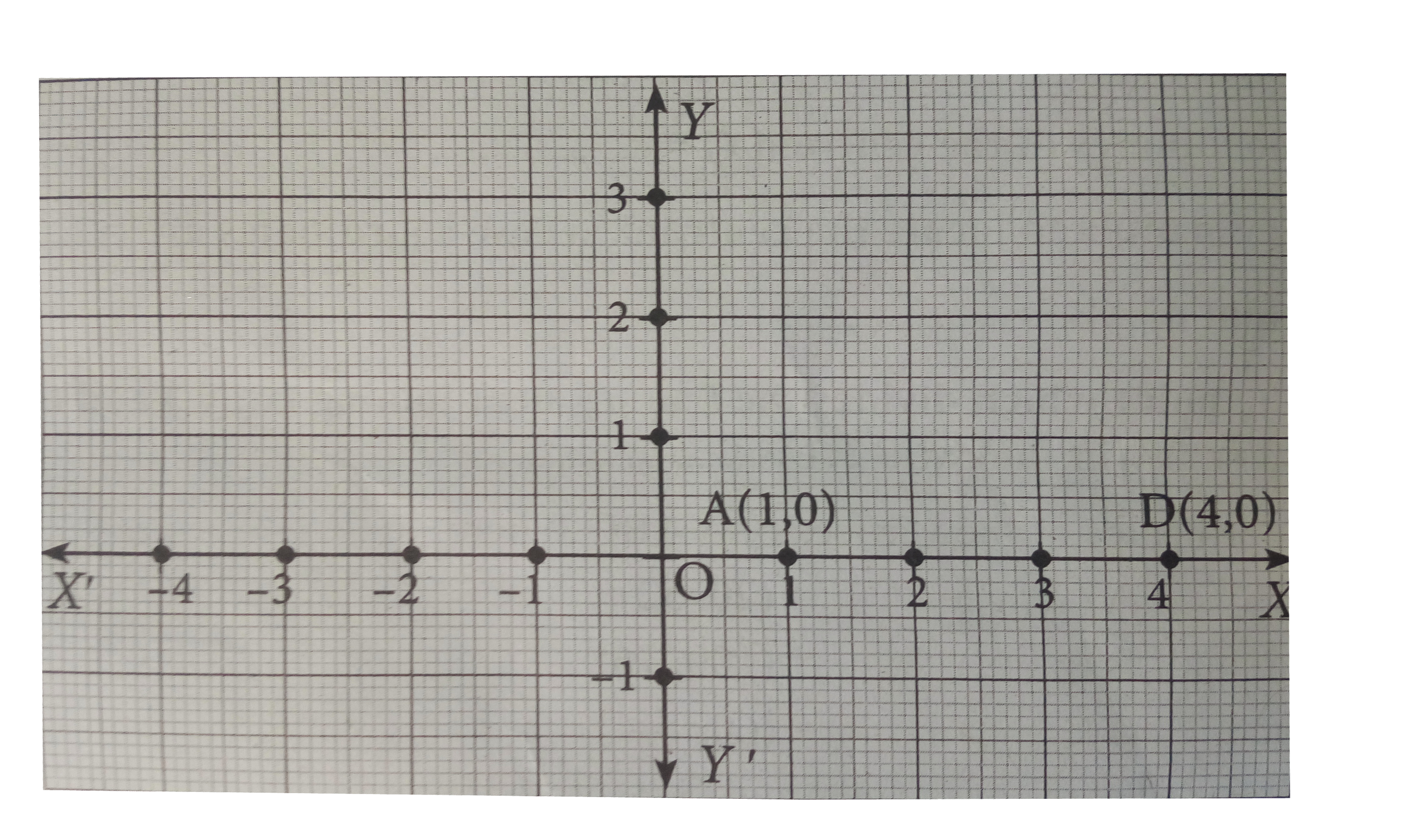
View SolutionPlot the following points on a graph sheet by taking the scale as 1cm=1 unit.
Find how far the points are from each other?
A(1,0) and D(4,0). Find AD and also DA.
Is AD=DA?
You plot another set of points and verify your Result.
View SolutionFind the area of a quadrilateral ABCD where AB = 7 cm, DA = 15 cm, AC = 9 cm, BC = 6 cm and CD = 12 cm.
View SolutionAB and CD are two parallel chords of a circle which are on opposite sides of the centre such that AB=10cm,CD=24cm and the distance between AB and CD is 17cm. Find the radius of the circle.
View SolutionAB and CD are two parallel chords of a circle such that AB=10cm and CD=24cm. If the chords are on the opposite sides of the centre and the distance between them is 17cm, find the radius of the circle.
View SolutionAB and CD are two parallel chords of a circle such that AB=10 cm and CD=24 cm. If the chords are on the opposite sides of the centre and the distance between them is 17 cm, find the radius of the circle.
View SolutionAB and CD are two parallel chords of a circle which are on opposite sides of the centre such that AB=10cm, CD=24cm and the distance between AB and CD is 17cm. Find the radius of the circle.
View SolutionAB and CD are two parallel chords of a circle which are on opposite sides of the centre such that AB =10cm , CD =24 cm and the distance between AB and CD is 17cm. Find the radius of the circle.
View SolutionAB और CD एक वृत्त की दो जीवाएं है जहँ AB = 10 सेमी, CD = 24 सेमी और AB∣∣CD है। AB और CD के बीच की दूरी 17 सेमी है। वृत्त की त्रिज्या ज्ञात कीजिए।
View SolutionAB व CD एक वृत्त कि दो समांतर जिवाएँ इस प्रकार है कि AB=10 सेमी तथा CD=24 सेमी। यदि ये जिवाएँ केंद्र के विपरीत दिशाओं में है तथा उनकी बीच कि दूरी 17 सेमी है तो वृत्त कि त्रिज्या ज्ञात कीजिए।
View SolutionIn a circle with centre O. AB and CD are parallel chords lying on opposite sides of a diameter parallel to them. If AB = 30 cm, CD - 48 cm and the distance between AB and CD is 27 cm, find the radius of the circle.
View SolutionIn a circle with centre O. AB and CD are parallel chords lying on opposite sides of a diameter parallel to them. If AB = 30 cm, CD - 48 cm and the distance between AB and CD is 27 cm, find the radius of the circle.
View SolutionAB and CD are two parallel chords of a circle such that AB=10cm and CD=24cm. If the chords are on the opposite sides of the centre and the distance between them is 17cm, find the radius of the circle.
View SolutionChords AB and CD of a circle are parallel to each other and lie on opposite sides of the centre of the circle. If AB = 36 cm, CD = 48 cm and the distance between the chords is 42 cm, find the radius of the circle.
View Solution
