Question

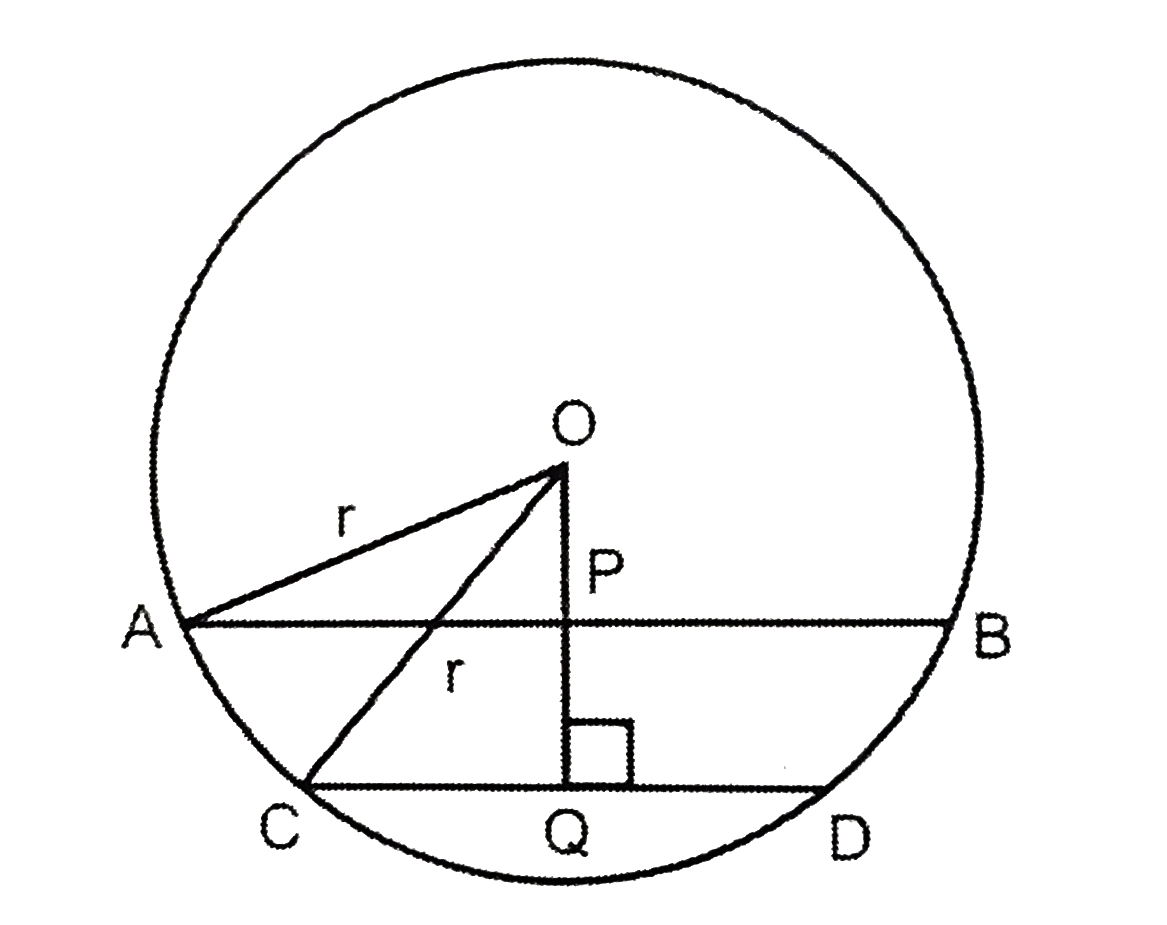
AB and CD are two parallel chords of a circle which are on opposite sides of the centre such that $AB=10cm$, $CD=24cm$ and the distance between AB and CD is $17cm$. Find the radius of the circle.

Answer

`r=13 cm`

Explanation

To find the radius of the circle given two parallel chords AB and CD, we can follow these steps:

1. Draw the Circle and Chords:
- Draw a circle with center O.
- Draw two parallel chords AB and CD such that AB = 10 cm and CD = 24 cm. Place AB above CD, with a distance of 17 cm between them.

2. Label Distances:
- Let the distance from the center O to chord AB be \( OP = x \).
- The distance from the center O to chord CD will then be \( OQ = 17 - x \) (since the total distance between the two chords is 17 cm).

3. Bisect the Chords:
- Since the perpendicular from the center of the circle to a chord bisects the chord:
- For chord AB, let \( AP = PB = \frac{AB}{2} = \frac{10}{2} = 5 \) cm.
- For chord CD, let \( CQ = QD = \frac{CD}{2} = \frac{24}{2} = 12 \) cm.

4. Apply the Pythagorean Theorem:
- For triangle OPB (right triangle):
\( OB^2 = OP^2 + PB^2 \implies r^2 = x^2 + 5^2 \implies r^2 = x^2 + 25 \quad \text{(Equation 1)} \)
- For triangle OQD (right triangle):
\( OD^2 = OQ^2 + QD^2 \implies r^2 = (17 - x)^2 + 12^2 \implies r^2 = (17 - x)^2 + 144 \quad \text{(Equation 2)} \)

5. Set Equations Equal:
- Since both expressions equal \( r^2 \):
\( x^2 + 25 = (17 - x)^2 + 144 \)

6. Expand and Simplify:
- Expand \( (17 - x)^2 \):
\( (17 - x)^2 = 289 - 34x + x^2 \)
- Substitute back into the equation:
\( x^2 + 25 = 289 - 34x + x^2 + 144 \)
- Cancel \( x^2 \) from both sides:
\( 25 = 433 - 34x \)
- Rearranging gives:
\( 34x = 433 - 25 \implies 34x = 408 \implies x = \frac{408}{34} = 12 \)

7. Find the Radius:
- Substitute \( x = 12 \) back into Equation 1:
\( r^2 = 12^2 + 25 = 144 + 25 = 169 \implies r = \sqrt{169} = 13 \text{ cm} \)

Final Answer:
The radius of the circle is 13 cm.

---