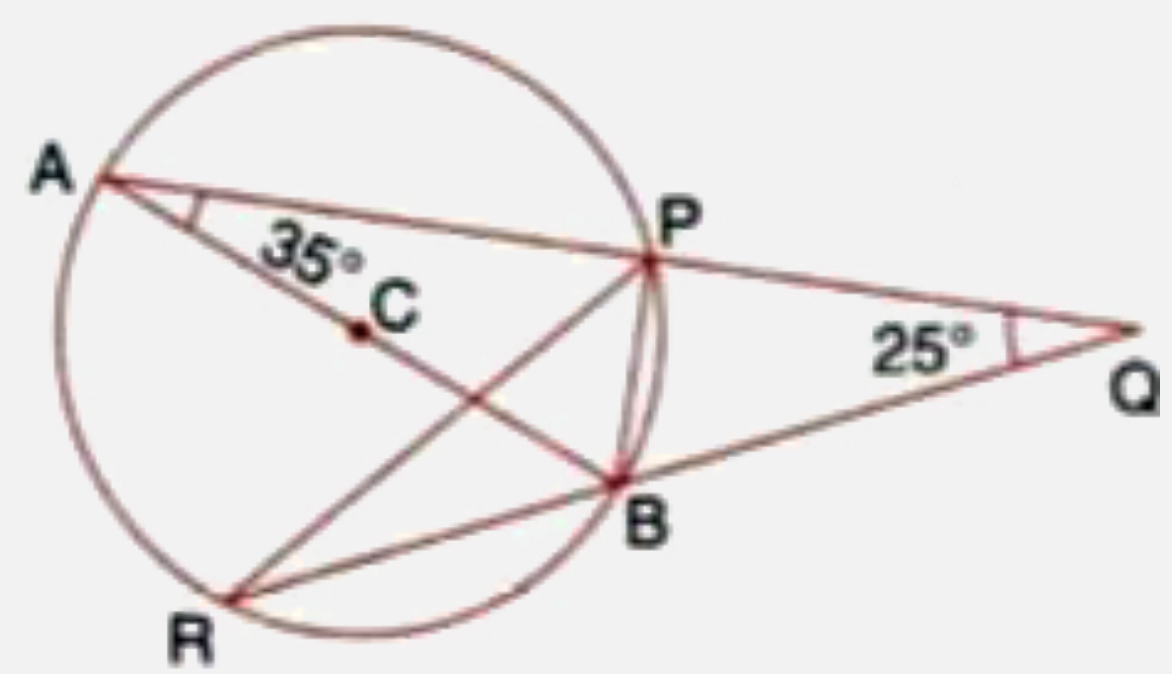
AB is a diameter of the circle APBR at shown in the figure. APQ and RBQ are straight lines
Find :
(i)
(ii)
(iii)
The correct Answer is:
Step by step video & image solution for AB is a diameter of the circle APBR at shown in the figure. APQ and RBQ are straight lines Find : (i) angle PRB (ii) angle PBR (iii) angle BPR. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - CIRCLESEXERCISE - EXERCISE 17(B)10videos
CIRCLES
- BOOK - ICSECHAPTER - CIRCLESEXERCISE - EXERCISE 17( C )28videos
CIRCLES
- BOOK - ICSECHAPTER - CIRCLESEXERCISE - EXERCISE 17( C )28videos
CIRCLES
- BOOK - ICSECHAPTER - CHAPTERWISE REVISION EXERCISEEXERCISE - CHAPTERWISE REVISION EXERCISE (PROBABILITY)16videos
CHAPTERWISE REVISION EXERCISE
- BOOK - ICSECHAPTER - CONSTRUCTIONS (CIRCLES)EXERCISE - EXERCISE39videos
CONSTRUCTIONS (CIRCLES)
Similar Questions
In the figure given , O is the centre of the circle . ∠DAE=70∘ , Find the giving suitable reasons , the measure of
(i) ∠BCD
(ii) ∠BOD
(iii) ∠OBD
View SolutionIn the given figure , AD is a diameter O is the centre of the circle AD is parallel to BC and ∠CBD=32∘
(i) ∠OBD
(ii )∠AOB
(iii) ∠BED
 View Solution
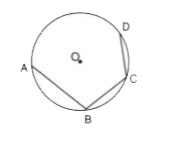
View SolutionIn the given figures , O is the centre of the given circle. AB is a side of a square ,BC
is a side of regular pentagon and CD is a side of regular hexagon. Find:
(i) ∠AOB
(ii) ∠AOC
(iii) ∠AOD
(iv)∠BCD
 View Solution
View SolutionIn the followoing figure , AD is the diameter of the circle with centre O. Chords AB,BC and CD are equal .If ∠DEF=110∘, Calculate :
(i) ∠AEF.
(ii) ∠FAB
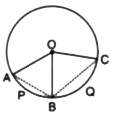
(##SEL_RKB_ICSE_MAT_X_C17_E03_002_Q01.png" width="80%">View SolutionIn the given figure , arc APB : arc BQC = 2: 3 and ∠AOC=150∘, Find :
(i) ∠AOB
(ii) ∠BOC
(iii) ∠OBA
(iv) ∠OCB
(v) ∠ABC
 View Solution
View SolutionIn the given figure. AB is a side of a regular six- sided polygon and AC is a side of a regular eight - sided polygon inscribed in the circle with centre O. Calculate the sizes of :
(i) ∠AOB
(ii) ∠ACB.
(iii) ∠ABC.
 View Solution
View SolutionIn the given figure , the lengths of arc AB and are BC arc in the ratio 3: 2 if ∠AOB=96∘, find
(i)∠CAB(ii)∠ADB
 View Solution
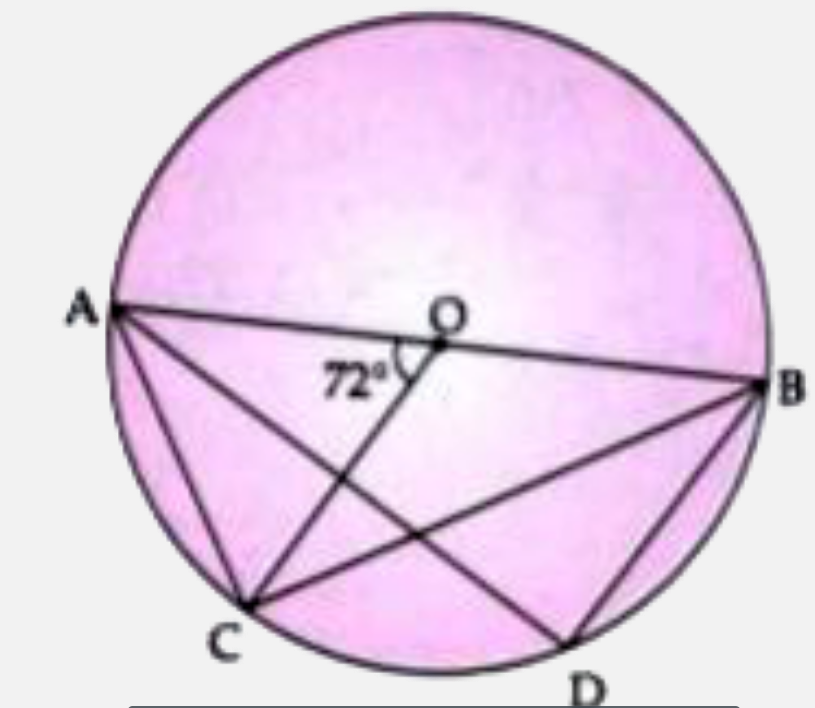
View SolutionIn the figure given below, O is the centre of the circle and Ab is a diameter.
If AC = BC and ∠AOC=72∘. Find:
(i) ∠ABC
(ii) ∠BAD
(iii) ∠ABD
 View Solution
View SolutionIn the figure given alongside ,AB and CD are straight lines thorugh the centre O of a circle. If ∠AOC=80∘and∠CDE=40∘ , find ∠DCE
 View Solution
View SolutionIn the given figure,AB is parallel to DC. ∠BCE=80∘and∠BAC=25∘.F∈d:(i) angle CAD <br>(ii) angle CBD <br>(iii) angle ADC
View Solution

