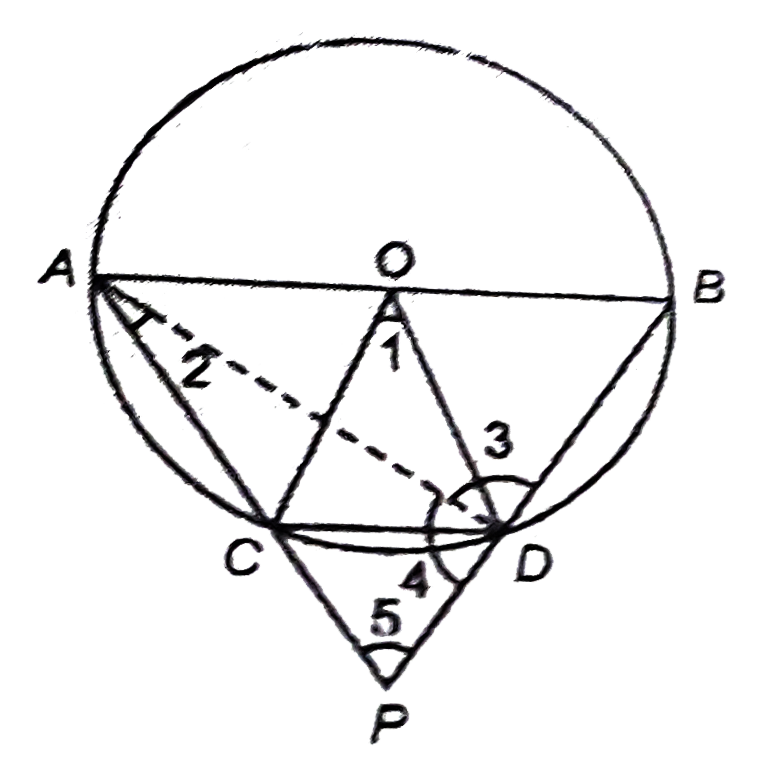
AB is a diameter of the circle with centre $O$ and chord $C D$ is equal to radius $O C$ (fig). $A C$ and $B D$ proudced meet at P. Prove that $∠ C P D = 60^{\circ}$ .

A

$60^{\circ}$

B

$30^{\circ}$

C

$90^{\circ}$

D

$160^{\circ}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Since, chord CD is equal to radius
$∴ D C = O C = O D$
$\Rightarrow ∠ 1 = 60^{\circ}$ ( $∵$ each angle of an equilateral triangle is of $60^{\circ}$ )
$∴ ∠ 2 \frac{1}{2} ∠ 1$ (degree measure theorem)
$= \frac{1}{2} \times 60^{\circ} = 30^{\circ}$ ........(1)
Now, since $∠ 3 = 90^{\circ}$ (angle in a semicircle is $90^{\circ}$ )
$\Rightarrow ∠ 4 = 90^{\circ}$ (linear pair axiom).....(2)
In $Δ A D P$ ,
$∠ 2 + ∠ 4 + a n \leq 5 = 180^{\circ}$ (angle sum property)
$\Rightarrow 30^{\circ} + 90^{\circ} + ∠ 5 = 180^{\circ}$
$\Rightarrow ∠ 5 = 180^{\circ} - 120^{\circ}$
$60^{\circ}$

|

Updated on:21/07/2023

Class 9 MATHS CIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
AB is a diameter of a circle with centre O. CD is a chord equal to the radius of the circle. AC and BD are produced to meet at P. Then the measure of $∠ A P B$ is:
A $120^{\circ}$
B $30^{\circ}$
C $60^{\circ}$
D $90^{\circ}$
Question 2 - Select One
AB is a diameter of the circle, CD is a chord equal to the radius of the circle. AC and BD when extended intersect at a point E. Find the $∠ A E B$ .
A $30^{\circ}$
B $60^{\circ}$
C $90^{\circ}$
D $45^{\circ}$
Question 3 - Select One
AB is a diameter of the circle with centre O, CD is chord of the circle. If $∠ B O C = 120^{\circ}$ , then the value of $∠ A D C$ is
A $42^{\circ}$
B $30^{\circ}$
C $60^{\circ}$
D $35^{\circ}$

NAGEEN PRAKASHAN-CIRCLE -Revision Exercise (long Answer Questions )

Home
Ask Doubt
Profile