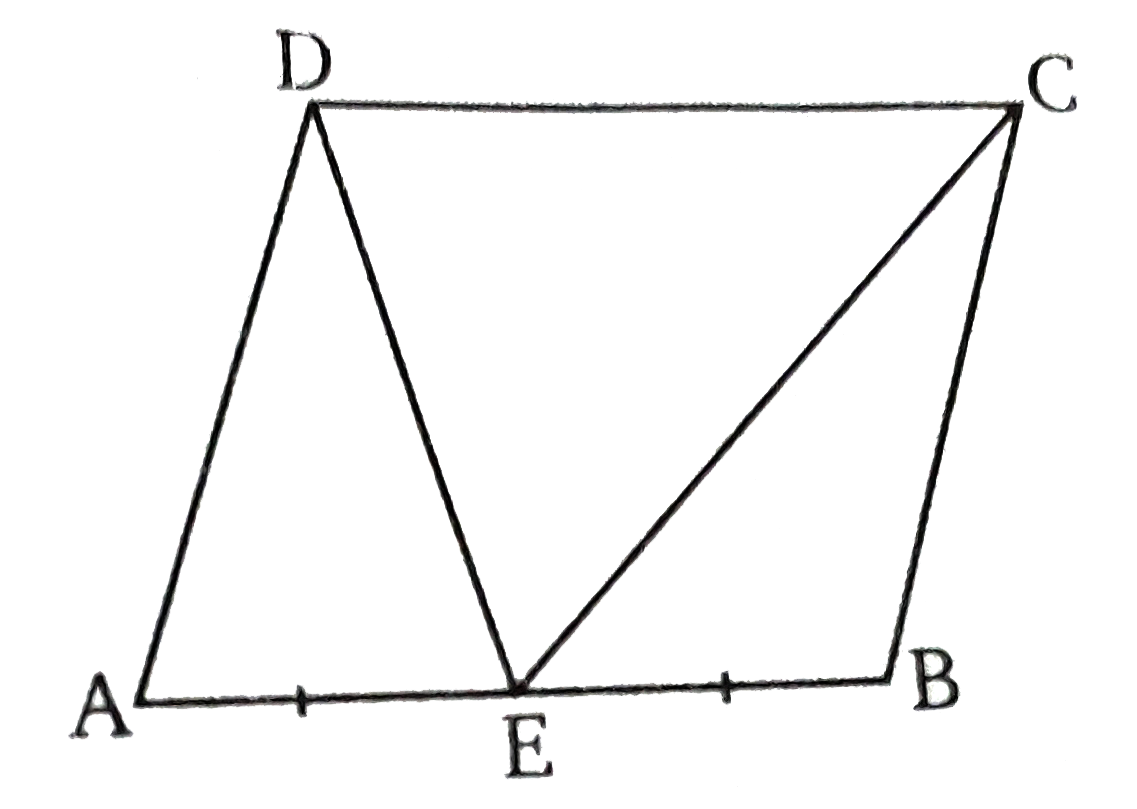
ABCD is a parallelogram, E is the mid-point of AB and CE bisects ∠BCD. Then ∠DEC is Solution in Tamil
60∘
90∘
100∘
120∘
The correct Answer is:B
Step by step video, text & image solution for ABCD is a parallelogram, E is the mid-point of AB and CE bisects angleBCD. Then angleDEC is by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRY BOOK - SURA PUBLICATIONCHAPTER - GEOMETRY EXERCISE - UNIT TEST (SECTION A)
5 Videos
- GEOMETRY BOOK - SURA PUBLICATIONCHAPTER - GEOMETRY EXERCISE - UNIT TEST (SECTION B)
5 Videos
- GEOMETRY BOOK - SURA PUBLICATIONCHAPTER - GEOMETRY EXERCISE - ADDITIONAL QUESTIONS AND ANSWERS (EXERCISE 4.6)
4 Videos
- COORDINATE GEOMETRYBOOK - SURA PUBLICATIONCHAPTER - COORDINATE GEOMETRYEXERCISE - UNIT TEST (SECTION - C)
4 Videos
- I TERM SUMMATIVE ASSESSMENT 2018-19BOOK - SURA PUBLICATIONCHAPTER - I TERM SUMMATIVE ASSESSMENT 2018-19EXERCISE - SECTION - I
40 Videos
Similar Questions
The exterior angle of a triangle is equal to the sum of two
View SolutionThe angles of a triangle are in the ratio 1:2:3, find the measure of each angles of the triangle.
View SolutionDraw the circumcircle for an equilateral triangle of side 6 cm.
View SolutionLocate the point (i) (3,5) and (5,3) (ii) (-2,-5) and (-5,-2) in the rectangular coordinate system.
View SolutionIf B-A is B, then is A∩B is
View SolutionIf ABCD is a parallelogram,E is the mid-point of AB and CE bisects /_BCD, then /_DEC is
View SolutionIn parallelogram ABCD,E is the mid-point of side AB and CE bisects angle BCD.Provethat: (i) AE=AD(i) DE bisects /_ADC and (ili) Angle DEC is a right angle.
View SolutionIn parallelogram ABCD,E is the mid-point of AD and F is the mid-point of BC.Prove that BFDE is a parallelogram.
View SolutionE and F are the mid-points of the sides AB and CD of a parallelogram ABCD. Prove that the line segment AF and CE trisects BD in three equal parts.
View Solutionसंलग्न चित्र में,ABCD एक समान्तर चतुर्भुर्ज है, E,AB का मध्य बिंदु है और CE,∠BCD को समद्विभाजित करता है। सिद्ध कीजिए की
(i) AE=AD
(ii) DE∠ADC को समद्विभाजित करता है।
(iii)∠DEC=90∘
View SolutionIn parallelogram ABCD, if P is the mid-point of side AB, then
View SolutionABCD is a parallelogram, E and F are the mid-points of BC and CD. Find the ratio of area of parallelogram ABCD and ΔAEF
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
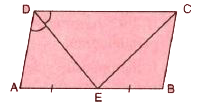
BC= BE
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
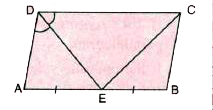
CE bisects angle C
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
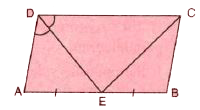
∠DEC=90∘
View Solution
