ABCD is a rhombus with AC=2BD. Diagonals AC and BD intersect at P.E1,E2,E3andE4 are four ellipes passing through P and their foci are A and B, B and C, C and D and D and A, respectively . If for i=1,2,3,4,ei are the eccentricities fo Ei, then Solution in Bengali
ei=e3
e2=e4
e1=2e2
e1=e2
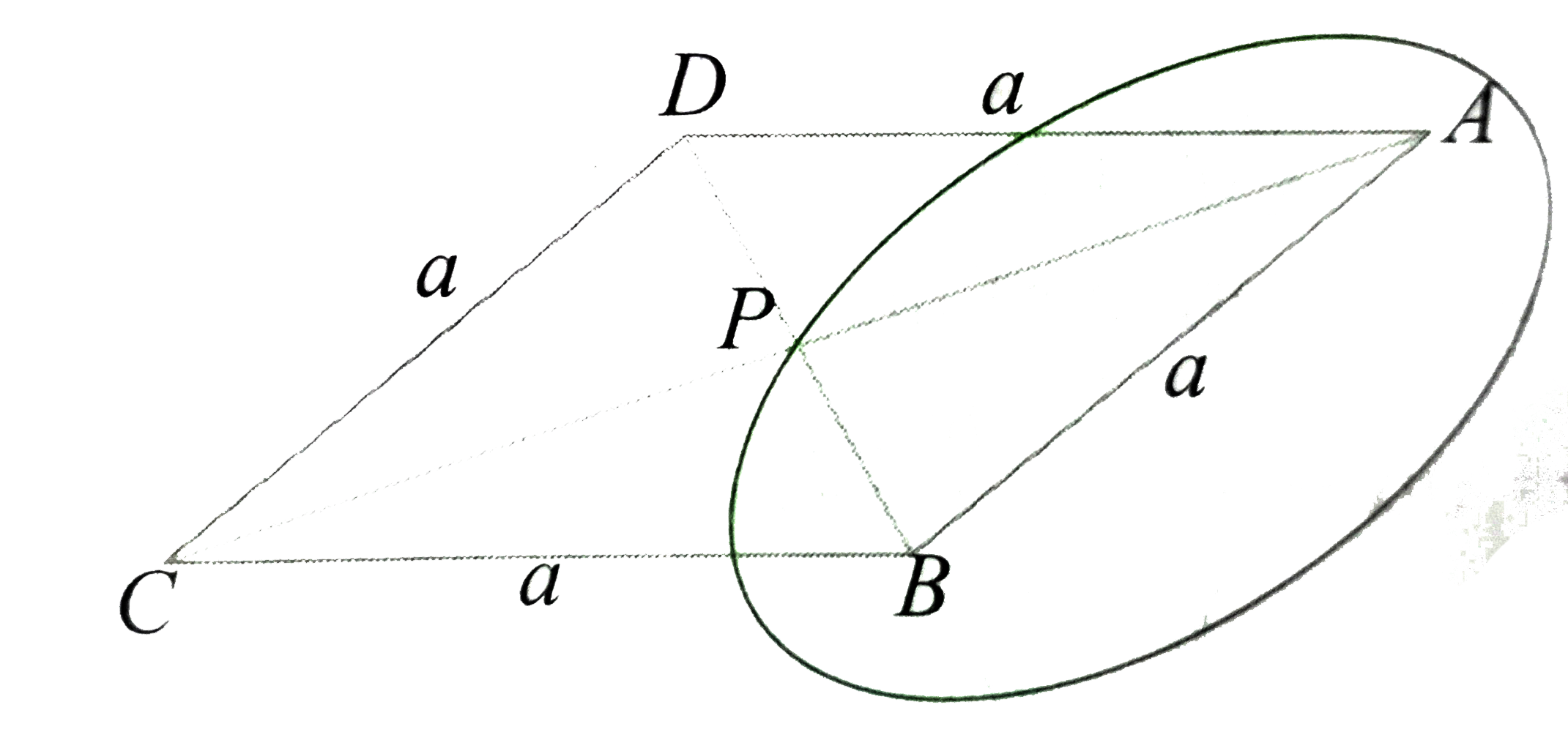
AB=BC=CD=DA=
Since ΔPAB,ΔPBC,ΔPCD,ΔPDA are congruent,
e1=e2=e3=34=aPA+PB
Similar Questions
एक सम पाँसे को उछाला गया है | घटनाओ E1={1,3,5},E2={2,3},E3={2,3,4,5} के लिए निम्न के मान ज्ञात कीजिए -
(i) P(E1/E2) व P(E2/E1)
(ii) P(E1/E3) व P(E3/E1)
(iii) P(E1∪E2/E3) व P(E1∩E2/E3)05:41View SolutionLet A, B,C be any three events in a sample space of a random experiment. Let the events E1= exactly one of A,B occurs, E2= exactly one of B ,c occurs, E3= exactly one of c ,A occurs, E4= all of A,B ,c occurs,E5= atleast one of A,B, c occurs P(E1)=P(E2)=P(E3)=13,P(E4)=19,P(E5)=
05:39View SolutionIf (3,4) and (5,12) are the foci of two conics both passing through origin, and the eccentricities of the conics are e1 and ,e2 where e1>e2 ,then 4e1e2 is equal to
05:20View SolutionAn urn contains four balls bearing numbers 1,2,3 and 123 respectively . A ball is drawn at random from the urn. Let Epi=1,2,3 donote the event that digit i appears on the ball drawn
statement 1 : P(E1∩E2)=P(E1∩E3)=P(E2∩E3)=14
Statement 2 : PE1=P(E2)=P(E3)=1202:02View SolutionIf e1 is the eccentricity of the ellipse x216+y225=1 and e2 is the eccentricity of a hyperbola passing through the foci of the given ellipse and e1e2=1, then the equation of such a hyperbola among the following is
06:16View SolutionIf e1,e2,e3 and e4 are the four elementary outcomes in a sample space and P(e1)=0.1,P(e2)=0.5,and P(e3)=0.1, then the probability of e4 is ……
01:08View SolutionABCD is a rhombus with AC=2BD. Diagonals AC and BD intersect at P.E1,E2,E3andE4 are four ellipes passing through P and their foci are A and B, B and C, C and D and D and A, respectively . If for i=1,2,3,4,ei are the eccentricities fo Ei, then
03:24View SolutionIf E1,E2,E3 and E4 are the four elementary outcomes in a sample space and P(E1)=0.1,P(E2)=0.5,P(E3)=0.1, then the probability of E4 is ……….
02:01View Solution

