An even periodic function f:R→R with period 4 is such that
f(x)={max.(|x|,x2),0≤x<1x,1≤x≤2. Then draw the graph of y=f(x) for x∈R
Video Solution
Text Solution
We have f(x)={max.(|x|,x2),0≤x<1x,1≤x≤2
Graph of y=f(x) for x∈[0,2] is as follows.
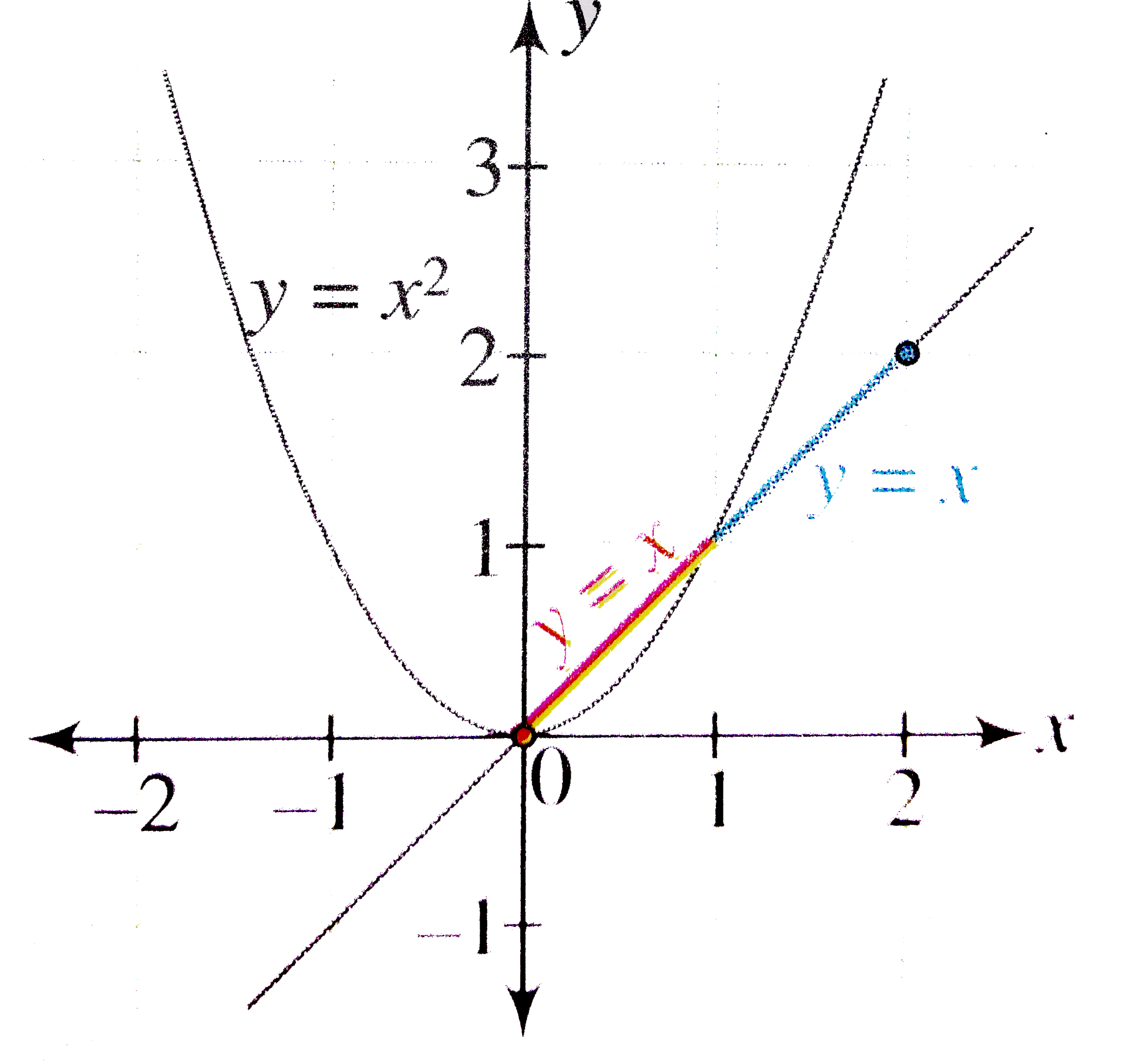
Now the function is even, so the graph of y=f(x) for [−2,2] is as follows.
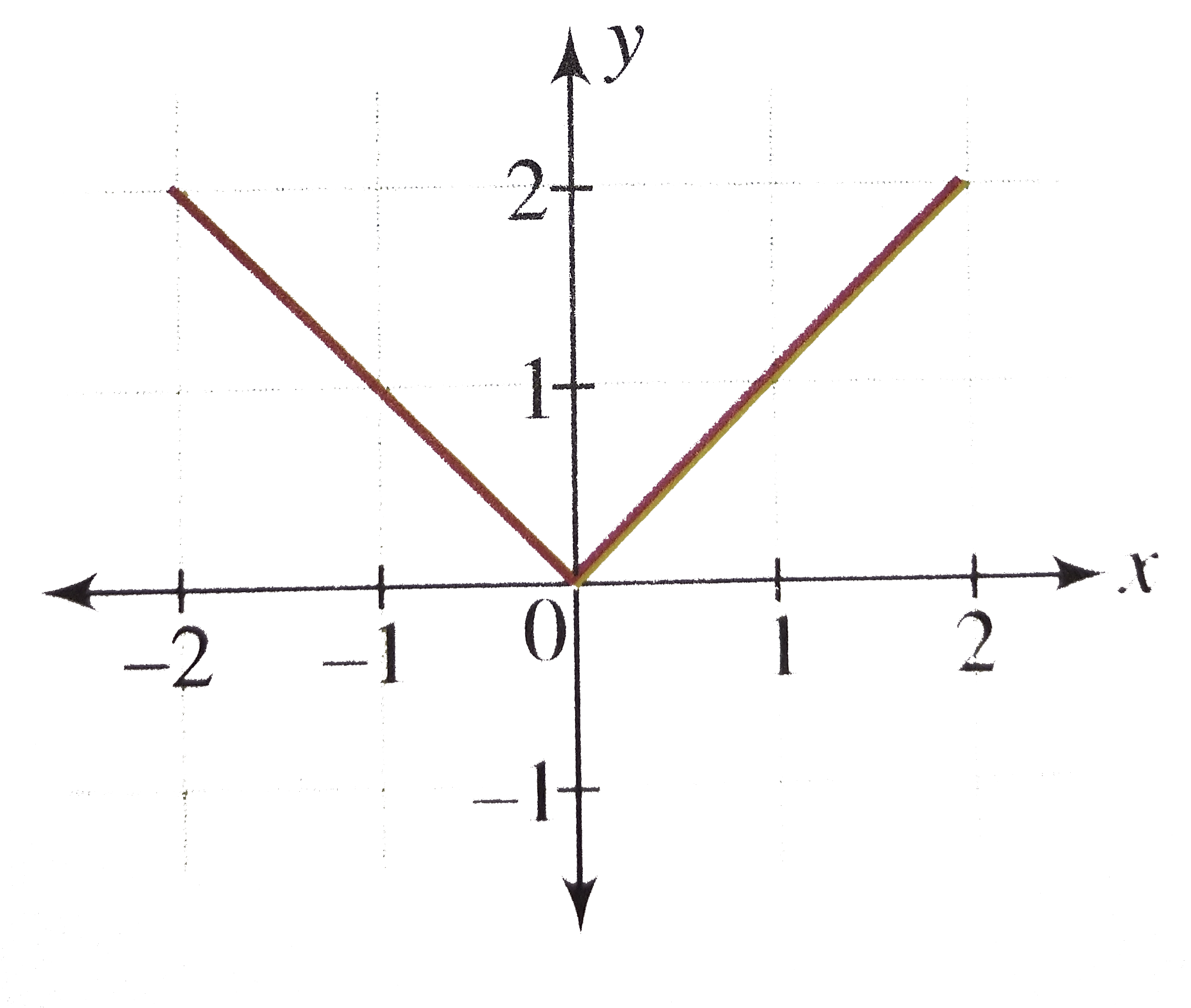
Further, the function is periodic with period '4', so the graph of y=f(x), for x∈R is as follows.
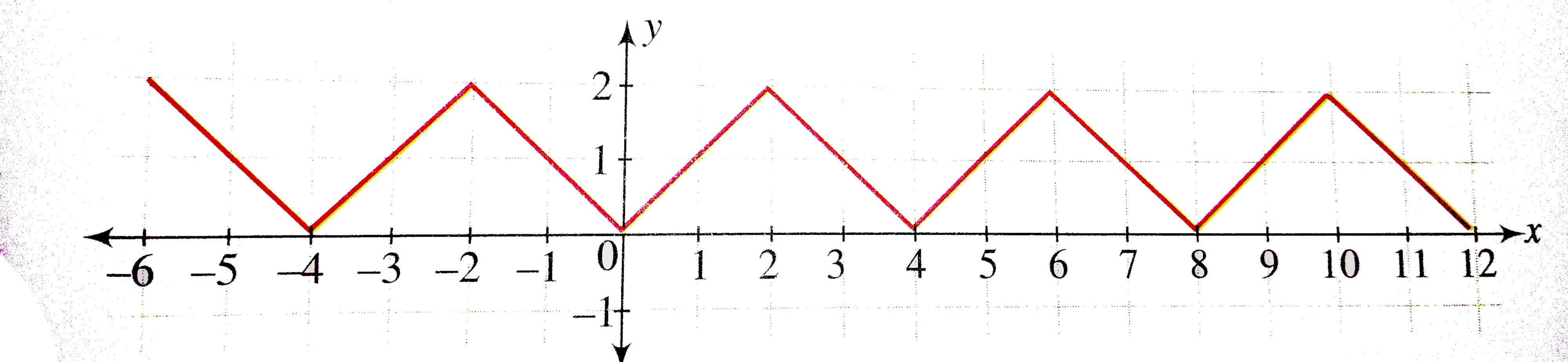
|
Topper's Solved these Questions
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercise
13 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
Similar Questions
Knowledge Check
An even periodic functin f:R→R with period 4 is such that
f(x)=[max.(|x|,x2)0≤x<1x1≤x≤2
The number of solution of f(x)|3sinx| for x∈(−6,6) are :A5B3C7D9An even periodic functin f:R→R with period 4 is such that
f(x)=[max.(|x|,x2)0≤x<1x1≤x≤2
The value of {f(5.12)} (where {.} denotes fractional part function), is :A{f(3.26)}B{f(7.88)}C{f(2.12)}D{f(5.88)}Let f(x)={xfor0≤x<13−xfor1≤x≤2
Then f(x) isAcontinuous at x =1BRight continuous at x =1CLeft continuous at x =1DLimit exists at x =1
Let f be an even function defined on R. Suppose f is defined on [0,4] as follows:
f(x)={3xif0≤x<14−xif1≤x≤4
and f satisfies the condition.
f(x−2)=f{x+[6x2+272x2+2])∀x∈R
Where [x] = greatest integer ≤x. Then f(3271)+f(−2052)+f(806)=..............
View SolutionSuppose that f is even, periodic function with period 2, and that f(x) = x for all x in the interval [0, 1]. Then draw the graph of y = f(x).
View SolutionFind the inverse of the function
f(x)=⎧⎪⎨⎪⎩x,x<1x2,1≤x≤48√x,x>4
View Solutionf (x) is an even periodic function with period 10 In [0,5],f(x)=⎧⎪⎨⎪⎩2x0≤x<23x2−82≤x<410x4≤x≤5. Then:
View SolutionLet f(x)={−1,−2≤x<0x2−1,0≤x≤2 and g(x)=|f(x)|+f(|x|). Then in the interval (−2,2), g is
View Solution
