Area of quadrilateral ABCD =Area of area of
Step by step video & image solution for Area of quadrilateral ABCD =Area of Delta ABC+ area of "_______" by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CBSE BOARDS 2020BOOK - X BOARDSCHAPTER - CBSE BOARDS 2020EXERCISE - SECTION B
22 Videos
- CBSE BOARDS 2020BOOK - X BOARDSCHAPTER - CBSE BOARDS 2020EXERCISE - SECTION C
34 Videos
- QUESTION PAPER 2022 TERM 1 SET 1 BASICBOOK - X BOARDSCHAPTER - QUESTION PAPER 2022 TERM 1 SET 1 BASICEXERCISE - SECTION-C (CASE STUDY)
10 Videos
Similar Questions
O' is an interior point of a parallelogram ABCD. Prove that :
area of ΔAOB+ area of ΔCOD= area of ΔAOD+ area of ΔBOC
View SolutionIn Quadrilateral ABCD, diagonal AC & BD intersect at "O". By Diagonals area of quadrilateral ABCD is divided into 4 parts. Find area of the fourth part (x), area of other 3 parts is mentioned in the figure.
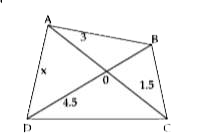
View Solution
Knowledge Check
In a ΔABC, G is centroid and AD, BE, CF are medians. Find the area of quadrilateral BDGF, if the area of ΔABC=72cm2
A12B16C24D8ABC is a right angled triangle, B being the right angle. Mid-points of BC and AC are respectively B' and A'. The ratio of the area of the quadrilateral AA' B'B to the area of the triangles ABC is
A1:2B2:3C3:4DNone of the above
In a triangle ABC with ∠BCA=90∘, the perpendicular bisector of AB intersects segments AB and AC at X and Y, respectively. If the ratio of the area of quadrilateral BXYC be the area of triangle ABC is 13:18 and BC=12 then which is the length of AC?
View SolutionIn parallelogram ABCD, P is a point on side AB and Q is a point on side BC, prove that
(i) ΔCPDandΔAQD are equal in area.
(ii) area (ΔAQD)= area of ΔAPD+ area of ΔCPB
View SolutionIf G is the centroid of ΔABC, then the area of ΔBGC is _______________ times the area of quadrilateral ABCG.
View SolutionThe diagonals of a cyclic quadrilateral ABCD intersect at P and the area of the triangle APB is 24 square cm. If AB = 8 cm and CD = 5 cm, then what is the area of the triangle CPD ?
View SolutionThe diagonals of quadrilateral ABCD, AC and BD intersects at O. Prove that if BO = OD then the triangles ABC and ADC are equal in area.
View SolutionO' is any point on diagonal AC of a parallelogram ABCD. Prove that :
area of ΔAOD= area of ΔAOB
View Solution
