At the foot of the mountain the elevation of its summit is 45∘, after ascending 100 m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘. The height of the mountain is
√3+12m
√3−13 m
√3+12√3 m
none of these
The correct Answer is:A
To solve the problem, we will break it down step by step.
Step 1: Understand the Problem
We need to find the height of the mountain given the angles of elevation and the distance traveled along a slope.
Step 2: Draw a Diagram
Draw a diagram to visualize the situation:
- Let A be the point at the foot of the mountain.
- Let B be the point after ascending 100 m along the slope.
- Let C be the summit of the mountain.
- The angle of elevation from A to C is 45°.
- The angle of elevation from B to C is 60°.
- The slope AB makes an angle of 30° with the horizontal.
Step 3: Identify the Angles
From the diagram:
- The angle ∠CAB = 45° (angle of elevation from A).
- The angle ∠ABC = 30° (slope inclination).
- The angle ∠BCM = 60° (angle of elevation from B).
Step 4: Calculate the Remaining Angles
Using the triangle properties:
- In triangle ABC, the angle ∠CBA = 180° - (∠CAB + ∠ABC) = 180° - (45° + 30°) = 105°.
- In triangle ABE, where E is the point vertically below C on the horizontal line through A, we can find the height.
Step 5: Use Trigonometric Ratios
Using the sine rule in triangle ABE:
- For triangle ABE:
BE=AB⋅sin(30°)=100⋅12=50 m
Using the cosine rule in triangle ABE:
- For triangle ABE:
AE=AB⋅cos(30°)=100⋅√32=50√3 m
Step 6: Calculate Height from B to C
Using the angle of elevation from B to C:
- In triangle BEC:
CE=BE⋅tan(60°)=50⋅√3 m
Step 7: Calculate Total Height of the Mountain
The total height of the mountain (H) is the sum of the height from A to E and from B to C:
- Total height H=AE+CE
- H=50√3+50√3=100√3 m
Final Answer
The height of the mountain is 100√3 m.
---
We need to find the height of the mountain given the angles of elevation and the distance traveled along a slope.
Draw a diagram to visualize the situation:
- Let A be the point at the foot of the mountain.
- Let B be the point after ascending 100 m along the slope.
- Let C be the summit of the mountain.
- The angle of elevation from A to C is 45°.
- The angle of elevation from B to C is 60°.
- The slope AB makes an angle of 30° with the horizontal.
From the diagram:
- The angle ∠CAB = 45° (angle of elevation from A).
- The angle ∠ABC = 30° (slope inclination).
- The angle ∠BCM = 60° (angle of elevation from B).
Using the triangle properties:
- In triangle ABC, the angle ∠CBA = 180° - (∠CAB + ∠ABC) = 180° - (45° + 30°) = 105°.
- In triangle ABE, where E is the point vertically below C on the horizontal line through A, we can find the height.
Using the sine rule in triangle ABE:
- For triangle ABE:
BE=AB⋅sin(30°)=100⋅12=50 m
- For triangle ABE:
AE=AB⋅cos(30°)=100⋅√32=50√3 m
Using the angle of elevation from B to C:
- In triangle BEC:
CE=BE⋅tan(60°)=50⋅√3 m
The total height of the mountain (H) is the sum of the height from A to E and from B to C:
- Total height H=AE+CE
- H=50√3+50√3=100√3 m
The height of the mountain is 100√3 m.
Topper's Solved these Questions
- HEIGHTS AND DISTANCESBOOK - OBJECTIVE RD SHARMACHAPTER - HEIGHTS AND DISTANCESEXERCISE - Exercise
45 Videos
- EXPONENTIAL AND LOGARITHMIC SERIESBOOK - OBJECTIVE RD SHARMACHAPTER - EXPONENTIAL AND LOGARITHMIC SERIESEXERCISE - Chapter Test
20 Videos
- INCREASING AND DECREASING FUNCTIONSBOOK - OBJECTIVE RD SHARMACHAPTER - INCREASING AND DECREASING FUNCTIONSEXERCISE - Chapter Test
20 Videos
Similar Questions
At the foot of a mountain the elevation of its summit is 45∘, after ascending 1000m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘ Find the height of the mountain.
View SolutionAt the foot of a mountain the elevation of its summit is 450; after ascending 1000 m towards the mountain up a slope of 300 inclination, the elevation is fund to be 600 . Find the height of the mountain.
View Solution
Knowledge Check
At the foot of the mountain, the elevation of its summit is 45∘ , after ascending 1000 m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘ . The height of the mountain is
A√3+12mB√3−12mC√3+12√3Dnone of theseAt the foot of a mountain, the elevation of its summit is 45∘. After ascending 2 km towards the mountain upon an incline of 30∘, the elevation changes to 60∘. The height of the mountain is
A(√3−1)kmB(√3+1)kmC(√3−2)kmD(√3−2)kmAt the foot of a mountain the elevation of its summit is 45∘. After ascending 2000 meter towards the mountain upon an incline of 30∘, the elevation changes to 60∘. What is the height of the mountain.
A2000(√3−1) meterB1000(√3−1) meterC2000(√3+1) meterD1000(√3+1) meter
At the foot of a mountain, the elevation of its summit is 45∘. After ascending 1000 m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘. find the height of the mountain.
View SolutionAt the foot of a mountain the elevation of its summit is 45∘. After ascending 600 meter towards the mountain, upon an incline of 30∘, the elevation changes to 60∘. Find the height of the mountain.
View SolutionAt the foot of a mountain the elevation of it's peak is found to be(π4) ,after ascending 'h' meters toward the mountain up a slope of π6 inclination,the elevation is found to be π3 .Height of the mountain is
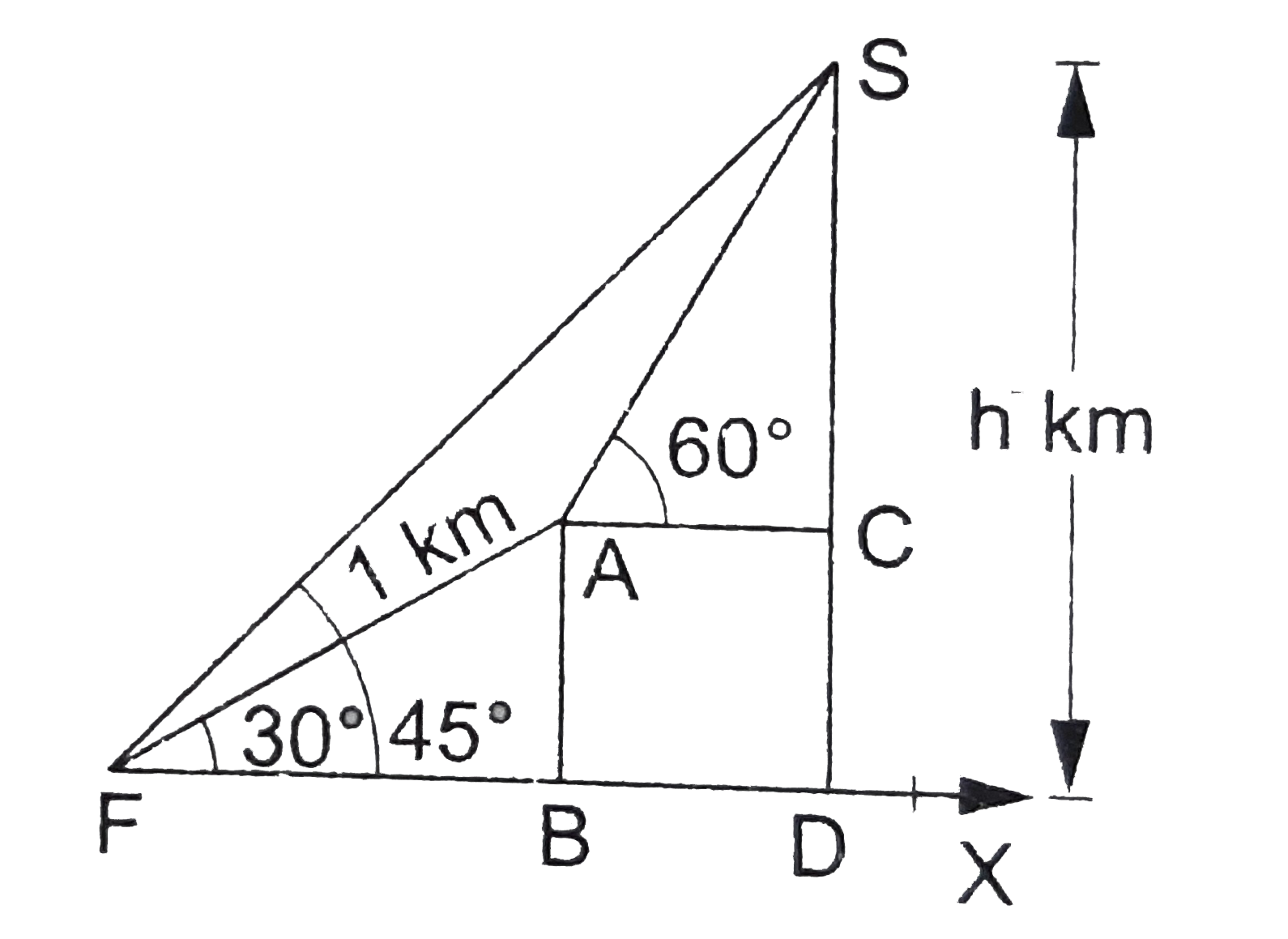
View SolutionAt the foot of a mountain, the angle of elevation of its summit is 45∘. After ascending 1 km towards the mountain up an incline of 30∘, the elevatioon changes to 60∘ (as shown in the given figure). Find the height of the mountain. [Given: √3=1.73.]
View SolutionFrom a point on the ground, the angle of elevation of a summit is found to be 45∘. After walking 150mt towards the mountain, the angle of elevation of the summit is found to be 60∘. The height of the mountain is:
View Solution
