At the foot of the mountain the elevation of its summit is , after ascending 1000 m towards the mountain up a slope of 30 degree inclination, the elevation found to be . Find the height of the mountain.Solution in Kannada
The correct Answer is:1.366 km
Step by step video, text & image solution for At the foot of the mountain the elevation of its summit is 45^(@), after ascending 1000 m towards the mountain up a slope of 30 degree inclination, the elevation found to be 60^(@). Find the height of the mountain. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - ZEN PUBLICATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - ADDITIONAL QUESTIONS (HOTS (HIGHER ORDER THINKING SKILLS ) QUESTIONS)11videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - ZEN PUBLICATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - ADDITIONAL QUESTIONS (VALUE BASED QUESTIONS )2videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - ZEN PUBLICATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - ADDITIONAL QUESTIONS ( SHORT ANSWER TYPE-2 QUESTIONS )6videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - ZEN PUBLICATIONCHAPTER - REAL NUMBERS EXERCISE - ADDITIONAL QUESTIONS(LONG ANSWER TYPE QUESTIONS )5videos
REAL NUMBERS
- BOOK - ZEN PUBLICATIONCHAPTER - STATISTICS EXERCISE - ZEN ADDITIONAL QUESTIONS IIT FOUNDATION5videos
STATISTICS
Similar Questions
The angle of elevation of the top of a hill from the foot of a tower is 60∘ and the angle of elevation of the top of the tower from the foot of the hill is 30∘. If the height of the tower is 50 m, what is the height of the hill?
06:30View SolutionThe angle of elevation of the top of a chimney from the foot of a tower is 60∘ and the angle of depression of the foot of the chimney from the top of the tower is 30∘. If the height of the tower is 40m, find the height of the chimney. According to pollution control norms, the minimum height of a smoke-emitting chimney should be 100m. State if the height of the above mentioned chimney meets the pollution control norms. What value is discussed in this question?
05:37View SolutionFrom the top of a tower 100 m high, a man observes two cars on the opposite sides of the tower with angle of depression 30∘ and 45∘ respectively. Find the distance between the cars
07:15View SolutionShow that the square of any odd integer is of the form 4q +1 for any integer q.
06:06View SolutionThe mean of five numbers is 18. If one number is excluded then their mean is 16. Find excluded number.
06:00View SolutionAt the foot of a mountain,the elevation of its summit is 45^(@). After ascending 1000m towards the mountain up a slope of 30^(@) inclination,the elevation is found to be 60^(@). find the height of the mountain.
05:26View SolutionAt the foot of a mountain the elevation of its summit is 45^(@), after ascending 1000m towards the mountain up a slope of 30^(@) inclination,the elevation is found to be 60^(@) Find the height of the mountain.
07:19View SolutionAt the foot of a mountain the elevation of its summit is 450; after ascending 1000 m towards the mountain up a slope of 300 inclination, the elevation is fund to be 600 . Find the height of the mountain.
05:40View SolutionAt the foot of a mountain, the angle of elevation of its summit is 45∘. After ascending 1 km towards the mountain up an incline of 30∘, the elevatioon changes to 60∘ (as shown in the given figure). Find the height of the mountain. [Given: √3=1.73.]
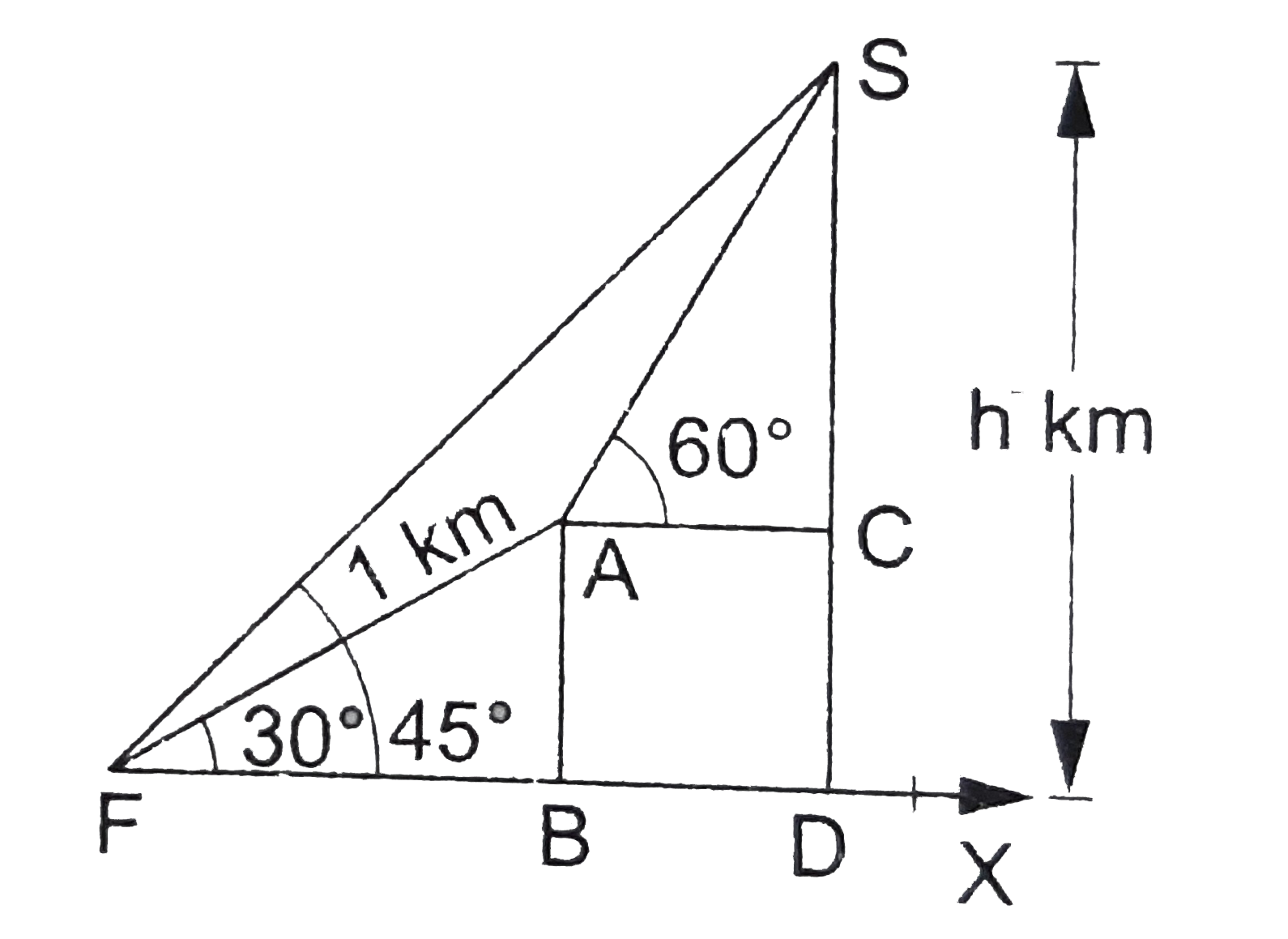 05:22View Solution
05:22View SolutionAt the foot of the mountain the elevation of its summit is 45∘, after ascending 100 m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘. The height of the mountain is
05:01View SolutionAt the foot of a mountain the elevation of it's peak is found to be(π4) ,after ascending 'h' meters toward the mountain up a slope of π6 inclination,the elevation is found to be π3 .Height of the mountain is
03:36View SolutionAt the foot of a mountain, the elevation of its summit is 45∘. After ascending 1000 m tounds the mountain up a slope of 30∘ inclination, the elevation is found 1o be 60∘. The height of the mountain is:
05:47View SolutionAt the foot of a mountain, the elevation of its summit is 45∘. After ascending 2 km towards the mountain upon an incline of 30∘, the elevation changes to 60∘. The height of the mountain is
06:15View SolutionAt the foot of a mountain the elevation of its summit is 45∘, after ascending 1000m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘ Find the height of the mountain.
04:24View SolutionAt the foot of a mountain, the angle of its summit is 45∘. After ascending 1000m towards the mountain up a slope of 30∘ inclination, the angle of elevation is found to be 60∘. Find the height of the mountain (√3=1.732)
09:28View Solution
