At the foot of a mountain, the elevation of its summit is 45∘. After ascending 2 km towards the mountain upon an incline of 30∘, the elevation changes to 60∘. The height of the mountain is
(√3−1)km
(√3+1)km
(√3−2)km
(√3−2)km
The correct Answer is:B
To solve the problem step by step, we will use trigonometric concepts related to heights and distances.
Step 1: Understand the Problem
We have a mountain with an elevation angle of 45 degrees from the foot of the mountain. After ascending 2 km at an incline of 30 degrees, the elevation angle changes to 60 degrees. We need to find the height of the mountain.
Step 2: Set Up the Diagram
Let:
- Point A be the foot of the mountain.
- Point B be the summit of the mountain.
- Point C be the point after ascending 2 km at a 30-degree incline.
- Point D be the point directly below B on the horizontal line from A.
Step 3: Determine the Height from Point C
From point C, the elevation angle to the summit (point B) is 60 degrees. The height of the mountain (AB) can be expressed in terms of the distance from point C to point D (CD) and the angle of elevation (60 degrees).
Using the tangent function:
tan(60∘)=ABCD
Since tan(60∘)=√3, we have:
√3=ABCD(1)
Step 4: Calculate CD
From point A to point C, we ascend 2 km at a 30-degree incline. We can find the vertical height (CD) using the sine function:
CD=2⋅sin(30∘)
Since sin(30∘)=12:
CD=2⋅12=1 km(2)
Step 5: Substitute CD into Equation (1)
Substituting CD=1 km into equation (1):
√3=AB1
Thus, we find:
AB=√3 km(3)
Step 6: Calculate the Height of the Mountain
Now we need to find the total height of the mountain, which is the height from point A to point B. Since we already calculated the height from point C to point B (AB) and the height from point A to point C (CD):
Total height=AB+CD
Substituting the values from (2) and (3):
Total height=√3+1 km
Final Answer
The height of the mountain is:
√3+1 km
We have a mountain with an elevation angle of 45 degrees from the foot of the mountain. After ascending 2 km at an incline of 30 degrees, the elevation angle changes to 60 degrees. We need to find the height of the mountain.
Let:
- Point A be the foot of the mountain.
- Point B be the summit of the mountain.
- Point C be the point after ascending 2 km at a 30-degree incline.
- Point D be the point directly below B on the horizontal line from A.
From point C, the elevation angle to the summit (point B) is 60 degrees. The height of the mountain (AB) can be expressed in terms of the distance from point C to point D (CD) and the angle of elevation (60 degrees).
tan(60∘)=ABCD
Since tan(60∘)=√3, we have:
√3=ABCD(1)
From point A to point C, we ascend 2 km at a 30-degree incline. We can find the vertical height (CD) using the sine function:
CD=2⋅sin(30∘)
Since sin(30∘)=12:
CD=2⋅12=1 km(2)
Substituting CD=1 km into equation (1):
√3=AB1
Thus, we find:
AB=√3 km(3)
Now we need to find the total height of the mountain, which is the height from point A to point B. Since we already calculated the height from point C to point B (AB) and the height from point A to point C (CD):
Total height=AB+CD
Substituting the values from (2) and (3):
Total height=√3+1 km
The height of the mountain is:
√3+1 km
Topper's Solved these Questions
- BOOK - ARIHANT SSCCHAPTER - HEIGHT AND DISTANCEEXERCISE - Multi Concept QUESTIONS2videos
HEIGHT AND DISTANCE
- BOOK - ARIHANT SSCCHAPTER - HCF AND LCMEXERCISE - Exercise Higher Skill Level Questions18videos
HCF AND LCM
- BOOK - ARIHANT SSCCHAPTER - INDICES AND SURDSEXERCISE - HIGHER SKILL LEVEL QUESTIONS17videos
INDICES AND SURDS
Similar Questions
At the foot of a mountain the elevation of its summit is 45∘. After ascending 600 meter towards the mountain, upon an incline of 30∘, the elevation changes to 60∘. Find the height of the mountain.
View SolutionAt the foot of a mountain, the angle of elevation of its summit is 45∘. After ascending 1 km towards the mountain up an incline of 30∘, the elevatioon changes to 60∘ (as shown in the given figure). Find the height of the mountain. [Given: √3=1.73.]
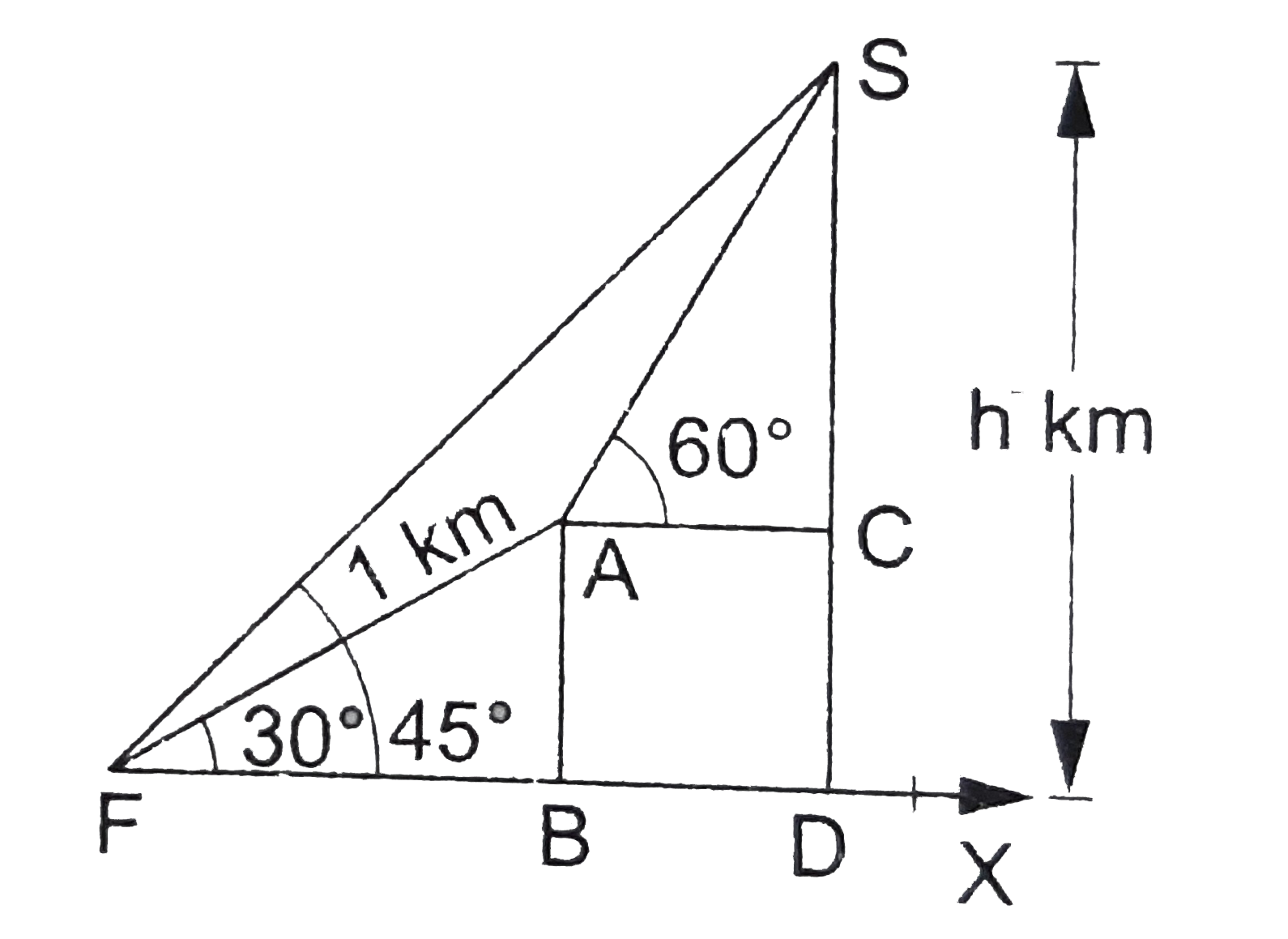 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
At the foot of a mountain the elevation of its summit is 45∘. After ascending 2000 meter towards the mountain upon an incline of 30∘, the elevation changes to 60∘. What is the height of the mountain.
A2000(√3−1) meterB1000(√3−1) meterC2000(√3+1) meterD1000(√3+1) meter - Question 2 - Select One
At the foot of the mountain the elevation of its summit is 45∘, after ascending 100 m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘. The height of the mountain is
A√3+12mB√3−13 mC√3+12√3 mDnone of these - Question 3 - Select One
At the foot of the mountain, the elevation of its summit is 45∘ , after ascending 1000 m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘ . The height of the mountain is
A√3+12mB√3−12mC√3+12√3Dnone of these
At the foot of a mountain, the elevation of its summit is 45∘. After ascending 1000 m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘. find the height of the mountain.
View SolutionAt the foot of a mountain the elevation of its summit is 450; after ascending 1000 m towards the mountain up a slope of 300 inclination, the elevation is fund to be 600 . Find the height of the mountain.
View SolutionAt the foot of a mountain the elevation of its summit is 45∘, after ascending 1000m towards the mountain up a slope of 30∘ inclination, the elevation is found to be 60∘ Find the height of the mountain.
View SolutionAt the foot of a mountain the elevation of it's peak is found to be(π4) ,after ascending 'h' meters toward the mountain up a slope of π6 inclination,the elevation is found to be π3 .Height of the mountain is
View SolutionFrom a point on the ground, the angle of elevation of a summit is found to be 45∘. After walking 150mt towards the mountain, the angle of elevation of the summit is found to be 60∘. The height of the mountain is:
View Solution

