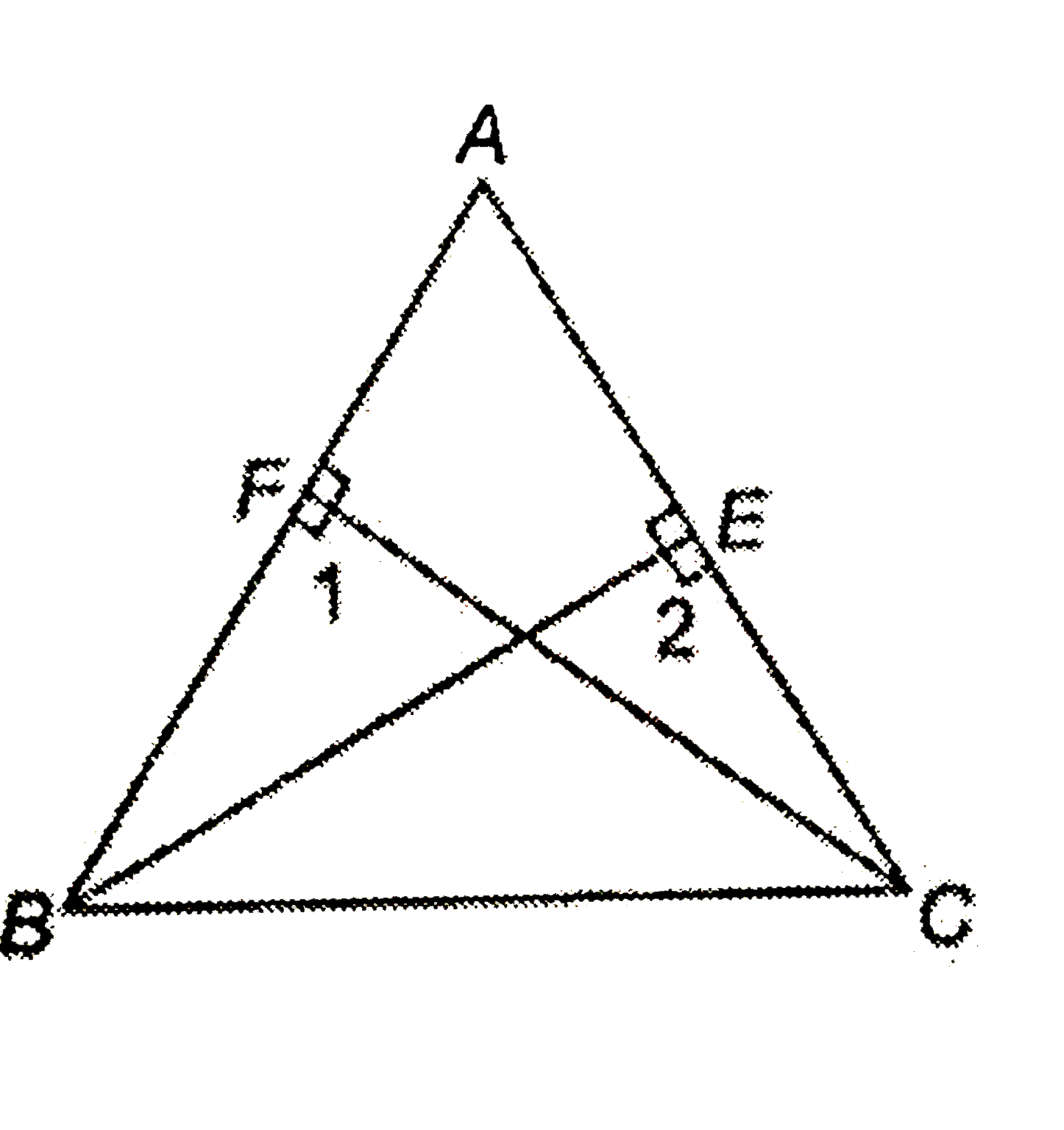
BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles
Given : ΔABC in which BE and CF are the altitudes such that BE=CF. To prove : ΔABC is an isosceles triangle i.e., AB=AC.
Proof : Since BE and CF are the altitudes
∴∠1=∠2 (each 90∘)
Now, in ΔBFC and ΔBEC,
∵⎧⎪⎨⎪⎩∠1=∠2(given)BC=BC(common)CF=BE(given)
∴ΔBFC≅ΔBEC (RIIS)
∴∠B=∠C (c.p.c.t)
⇒AC=AB (sides opposite to equal angles are equal)
∴ΔABC is an isosceles triangle. Hence Proved.
We can also prove this by taking AAS congruence rule in Δs ABE and AFC, if no particular method is asked)
Topper's Solved these Questions
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar
5 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 7a
35 Videos
- SURFACE AREA AND VOLUMEBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)
10 Videos
Similar Questions
In a triangle ABC
View SolutionIf the altitudes from two vertices of a triangle to the opposite sides are equal, prove that the triangle is isosceles.
View SolutionIf the altitudes from two vertices of a triangle to the opposite sides are equal, prove that the triangle is isosceles.
View SolutionIf A, B, C are the angles of a triangle ABC and if cosA=sinB2sinC, show that the triangle is isosceles.
View SolutionProve that the altitudes of a triangle are concurrent.
View SolutionAD, BE and CF , the altitudes of triangle ABC are equal. Prove that ABC is an equilateral triangle.
View SolutionIn △ABC, if cosA+sinA−2cosB+sinB=0 then prove that triangle is isosceles right angled.
View SolutionIf atanA+btanB=(a+b)tan(A+B2) prove that triangle ABC is isosceles.
View SolutionThe sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and the median PM of triangle PQR respectively. Prove that the triangles ABC and PQR are congruent.
View SolutionIf in a triangle ABC,bc2cosA=b2+c2−2bccosA then prove that the triangle must be isosceles.
View Solution
