Consider a triangle PQR with coordinates of its vertices as P(-8,5), Q(-15, -19), and R (1, -7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0. triangle PQR is
The sum a + c isSolution in Bengali
129
78
89
none of these
The correct Answer is:C
The coordinates of D using section formula are (-5, -23/2) and
mPD=−11/2. The equation of PD is
11x+2y+78=0
or a+c = 89
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)8videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (NUMERICAL VALUE TYPE)13videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MULTIPLE CORRECT ANSWERS TYPE)30videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
Match the following lists:
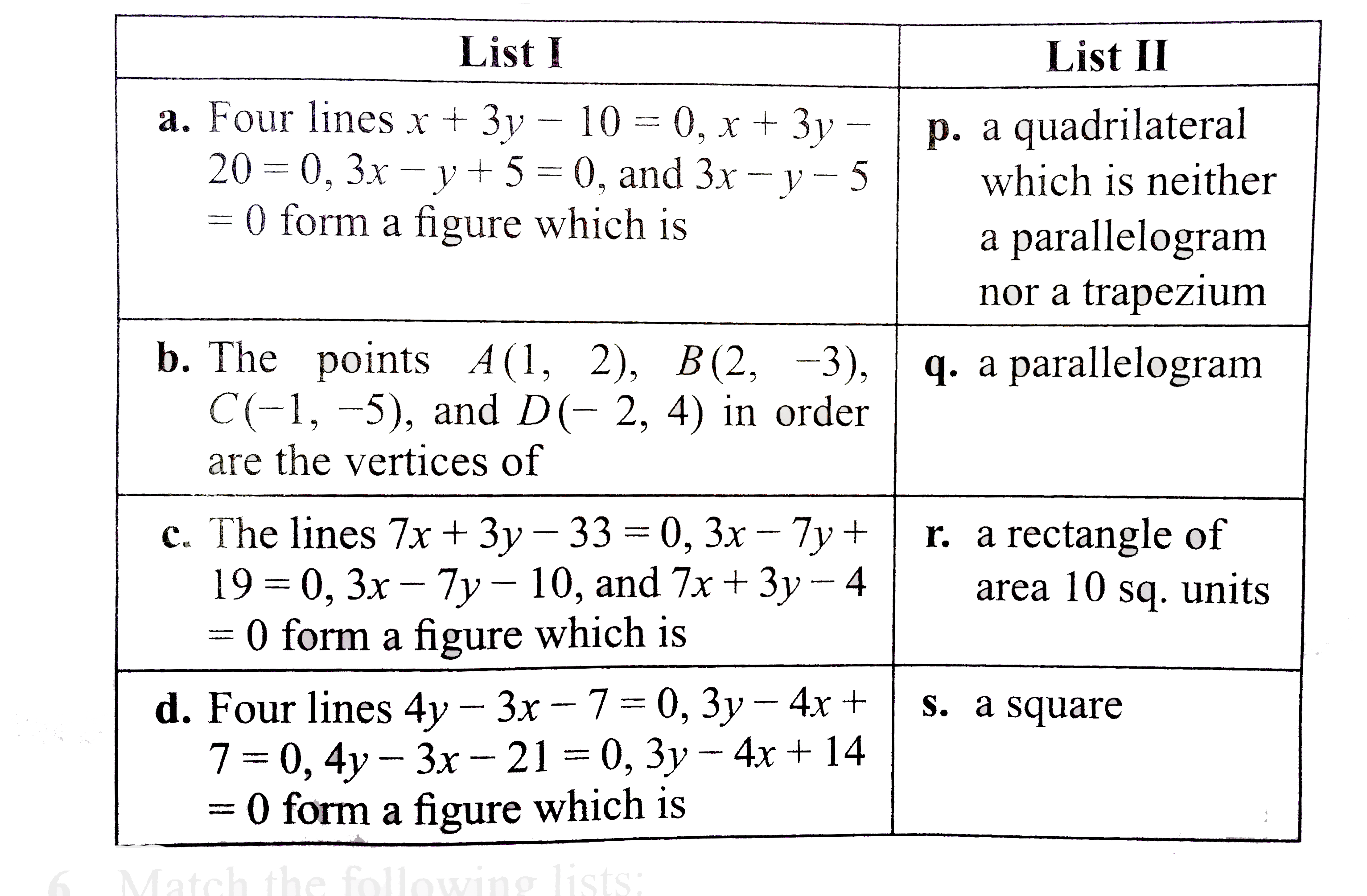 03:39View Solution
03:39View SolutionFind the number of permutations of the set {1,2,3,4} in which no two adjacent positions are filled by consecutive integers ( increasing orders).
06:42View SolutionIf P is a point (x,y) on the line y=−3x such that P and the point (3, 4) are on the opposite sides of the line 3x−4y=8, then x>815 (b) x>85 y<−85 (d) y<−815
08:59View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionThe interior angle bisector of angle P for the trangle PQR whose coordinates of the veetices are P(−8,5);Q(−15,−19)andR(1,−7) has the equation ax+2y+c=0, then (a,c)= a=11 a=10 c=78 c=80
03:27View SolutionThe interior angle bisector of angle A for the triangle ABC whose coordinates of the vertices are A(−8,5),B(−15,−19)andC(1,−7) has the equation ax+2y+c=0 Find 'a' and 'c'
06:42View SolutionConsider a triangle PQR with coordinates of its vertices as P(-8,5), Q(-15, -19), and R (1, -7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0.
The distance between the orthocenter and the circumcenter of triangle PQR is02:17View SolutionConsider a triangle PQR with coordinates of its vertices as P(−8,5),Q(−15,−19), and R(1,−7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0.
The radius of the in circle of triangle PQR is06:52View SolutionConsider a triangle PQR with coordinates of its vertices as P(-8,5), Q(-15, -19), and R (1, -7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0.
The radius of the in circle of triangle PQR is
The sum a + c is09:33View SolutionConsider a triangle PQR with coordinates of its vertices as P(-8,5), Q(-15, -19), and R (1, -7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0.
The distance between the orthocenter and the circumcenter of triangle PQR is07:23View SolutionConsider a triangle PQR with coordinates of its vertices as P(-8,5), Q(-15, -19), and R (1, -7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0.
The radius of the in circle of triangle PQR is09:26View SolutionConsider a triangle PQR with coordinates of its vertices as P(-8,5), Q(-15, -19), and R (1, -7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0.
The radius of the in circle of triangle PQR is
The sum a + c is07:28View SolutionConsider a triangle PQR with coordinates of its vertices as P(-8,5), Q(-15,-19), and R(1,-7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0
The distance between the orthocenter and the circumcenter of triangle PQR is06:40View SolutionConsider a triangle PQR with coordinates of its vertices as P(-8,5), Q(-15,-19), and R(1,-7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0
The radius of the incircle of triangle PQR is08:22View SolutionConsider a triangle PQR with coordinates of its vertices as P(-8,5), Q(-15,-19), and R(1,-7). The bisector of the interior angle of P has the equation which can be written in the form ax+2y+c=0
The sum a+c is02:08View Solution
