Question

Consider the function $f\left(x\right)=\{\begin{array}{cc}x-\left[x\right]-\frac{1}{2}& x\notin I\\ 0& x\in I\end{array}$ where [.] denotes the greatest integer function and I is the set of integers. Then find $g\left(x\right)max.[x^2,f\left(x\right),\left|x\right|\},-2\le x\le 2.$

Explanation

We first draw the graphs of `y=f(x),y=x^(2)andy=|x|``y=f(x),y=x^(2)andy=|x|`.
To deaw the graph of `y=x-[x]-0.5={x}-0.5`, we shift the graph of `y={x},0.5` units downward vertically for non-nitegral values of x and plotting '0' for integral values fo x as shown in the following in the following figure.

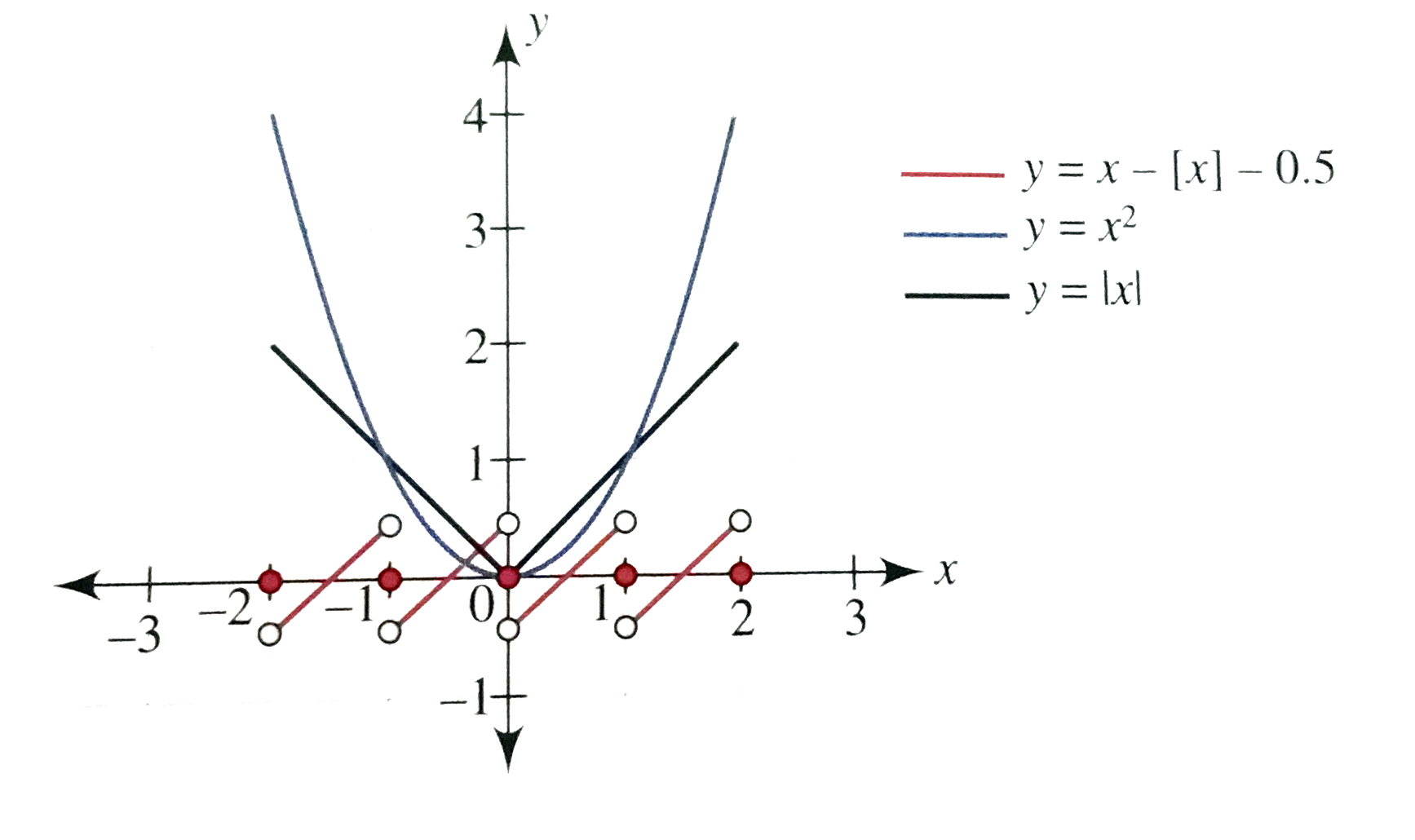
Clearly, from the graph, g(.x) =`{{:(x^(2)",",-2lexle-1),(1-x",",-1lexle-1//4),((1)/(2)+x",",-(1)/(4)ltxlt0),(1+x",",0lexle1),(x^(2)",",1lele2):}`