Draw a right triangle and the perpendicular from the midpoint of the hypotenuse to the base.
i) Prove that this perpendicular is half the perpendicular side of the large triangle.
ii) Prove that this perpendieular bisects the bottom side of the larger triangle.
iiii) Prove that in the large triangle, the distances from the midpoint of the hypoteniuse to all the vertices are equal.
iv) Prove thàt the circumcentre of a right triangle is the midpoint of its hypotenuse.Solution in Malayalam
Step by step video & image solution for Draw a right triangle and the perpendicular from the midpoint of the hypotenuse to the base.i) Prove that this perpendicular is half the perpendicular side of the large triangle.ii) Prove that this perpendieular bisects the bottom side of the larger triangle.iiii) Prove that in the large triangle, the distances from the midpoint of the hypoteniuse to all the vertices are equal. iv) Prove thàt the circumcentre of a right triangle is the midpoint of its hypotenuse. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In the picture, the square on the hypotenuse of the top most right triangle Is drawn. Calculate the area and the length of a side of the square.
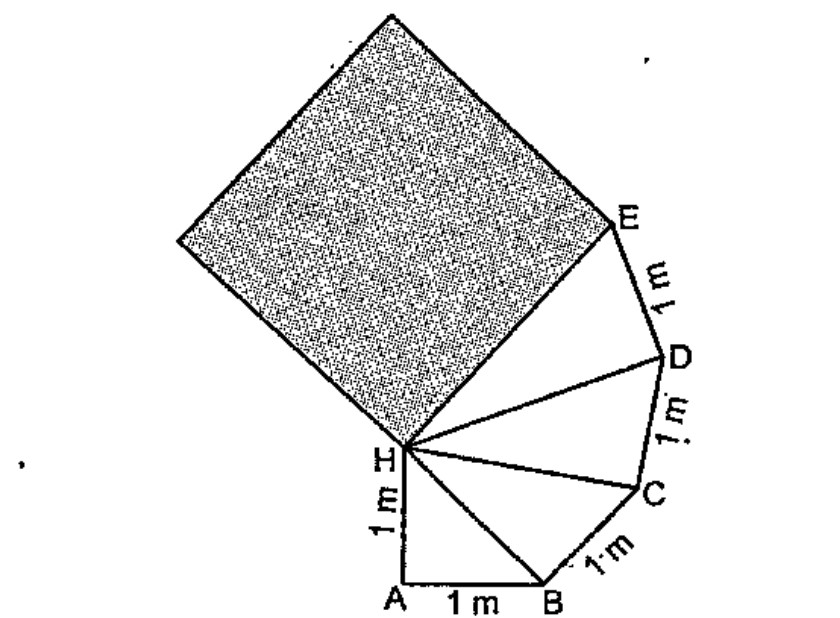 .
.
View SolutionIn rectangle with one side 1cm shorter than the other , take the length of the shorter side as x cm.
i) Taking their perimeters as p(x) cm, write the relation between p(x) and x as an equation.
ii) Taking their areas as a(x) sq.cm, write the relation between a(x) and x as an equation.
iii) Calculate p(1),p(2),p(3),p(4),p(5). Do you see any pattern?
iv) Calculate a(1),a(2),a(3),a(4),a(5).Do you see any pattern?
View SolutionIf a perpendicular is drawn from the vertex containing the right angle of a right triangle to the hypotenuse then prove that the triangle on each side of the perpendicular are similar to each other and to the original triangle. Also, prove that the square of the perpendicular is equal to the product of the lengths of the two parts of the hypotenuse.
View SolutionProve that the perpendicular let fall from the vertices of a triangle to the opposite sides are concurrent.
View Solutionसिद्ध कीजिए कि त्रिभुज की शीर्षों से सम्मुख भुजाओं पर डाले गये लम्ब संगामी होते हैं |
View Solutionसिद्ध कीजिए कि त्रिभुज की शीर्षों से सम्मुख भुजाओं पर डाले गये लम्ब संगामी होते हैं |
View Solutionसिद्ध करें कि समद्विबाहु त्रिभुज के शीर्षकोण के अर्द्धक रेखा आधार पर लम्ब होती है और उसे समद्विभाजित करती है |
View Solution(i) साबित करें कि समकोण त्रिभुज मैं कर्ण का वर्ग अन्य दो भुजाओं के वर्गों के
योगफल के बराबर होता है |
(ii) साबित करें कि समकोण त्रिभुज मैं कर्ण का मध्यबिन्दु तीनों शीर्षों से सामान दुरी
पर होता है |
View SolutionProve by vector method that the perpendiculars (altitudes) from the vertices to the sides of a triangle are concurrent.
View Solutionযুক্তি দিয়ে প্রমাণ করো, যে-কোনো সমকোণী ত্রিভুজের অতিভুজের মধ্যবিন্দু ত্রিভুজের তিনটি শীর্ষবিন্দু থেকে সমদূরবর্তী ।
View SolutionIf a perpendicular is drawn from the vertex containing the right angle of a right triangle to the hypotenuse then prove that the triangle on each side of the perpendicular are similar to each other and to the original triangle. Also, prove that the square of the perpendicular is equal to the product of the lengths of the two parts of the hypotenuse.
View SolutionIf a perpendicular is drawn from the vertex containing the right angle of a right triangle to the hypotenuse then prove that the triangle on each side of the perpendicular are similar to each other and to the original triangle. Also, prove that the square of the perpendicular is equal to the product of the lengths of the two parts of the hypotenuse.
View Solutionसिद्ध कीजिए कि किसी त्रिभुज की भुजाओं के लम्ब अर्द्धक संगामी होते हैं |
View SolutionDraw a right triangle and the perpendicular from the midpoint of the of the hypotenuse to the base
Prove that this perpendicular is half the perpendicular side of the large triangle.
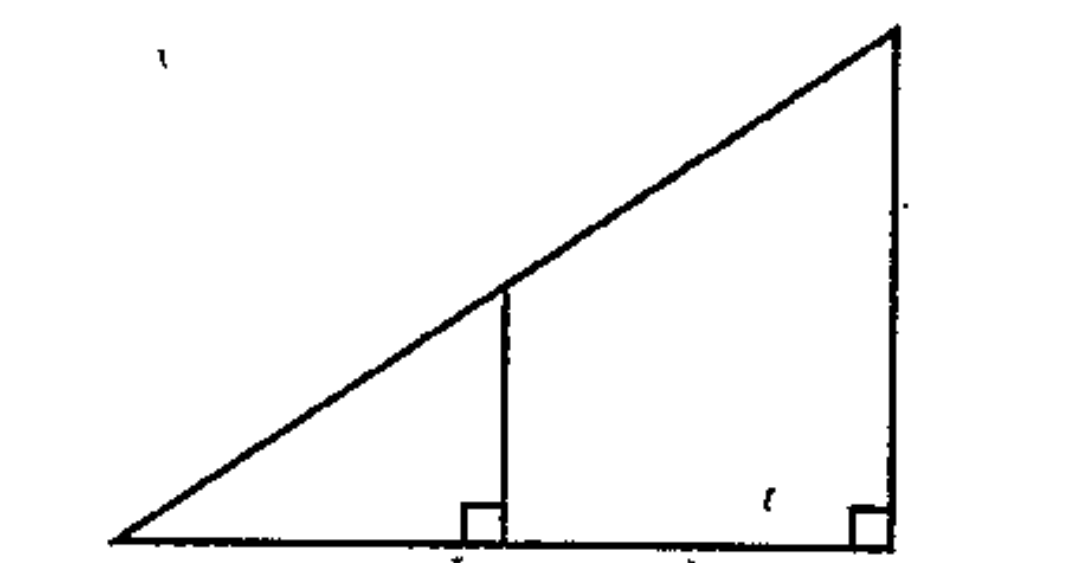 .
.
View SolutionDraw a right triangle and the perpendicular from the midpoint of the of the hypotenuse to the base
Prove that perpendicular bisects the bottom side of the larger triangle.
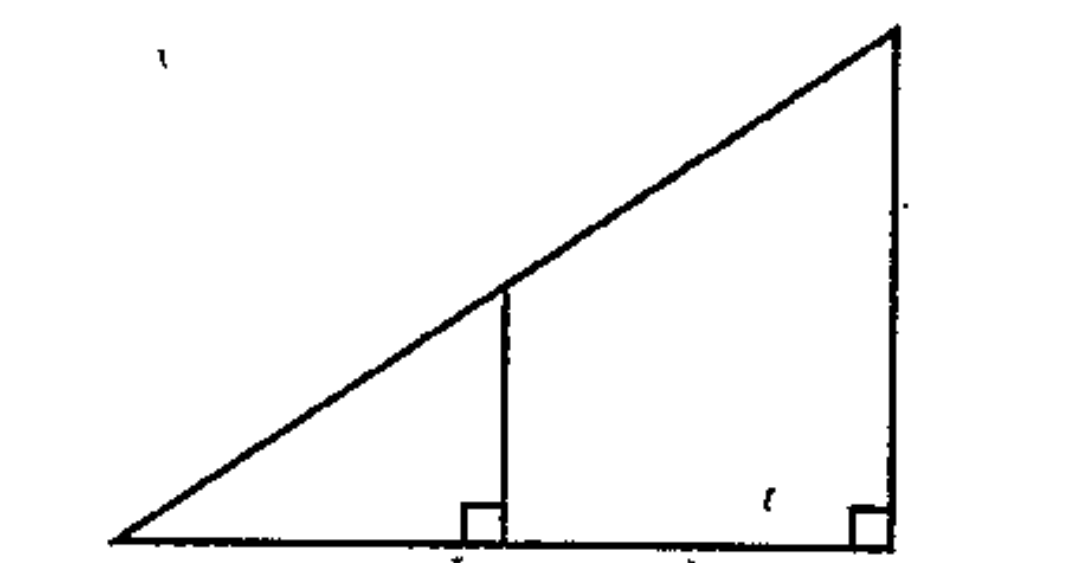 .
.
View SolutionDraw a right triangle and the perpendicular from the midpoint of the of the hypotenuse to the base
Prove that in the large triangle the distances from the midpoint of the hypotenuse to all thevertices are equal.
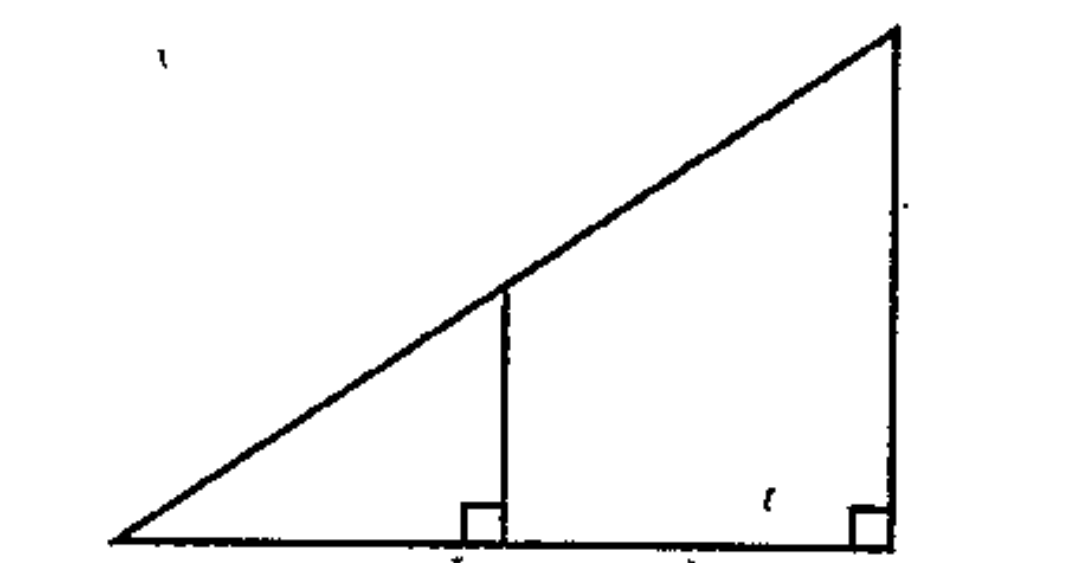 .
.
View Solution
