Find the area bounded by the curve $y = x (x - 1) (x - 2)$ and the x-axis.

Video Solution

Text Solution

Verified by Experts

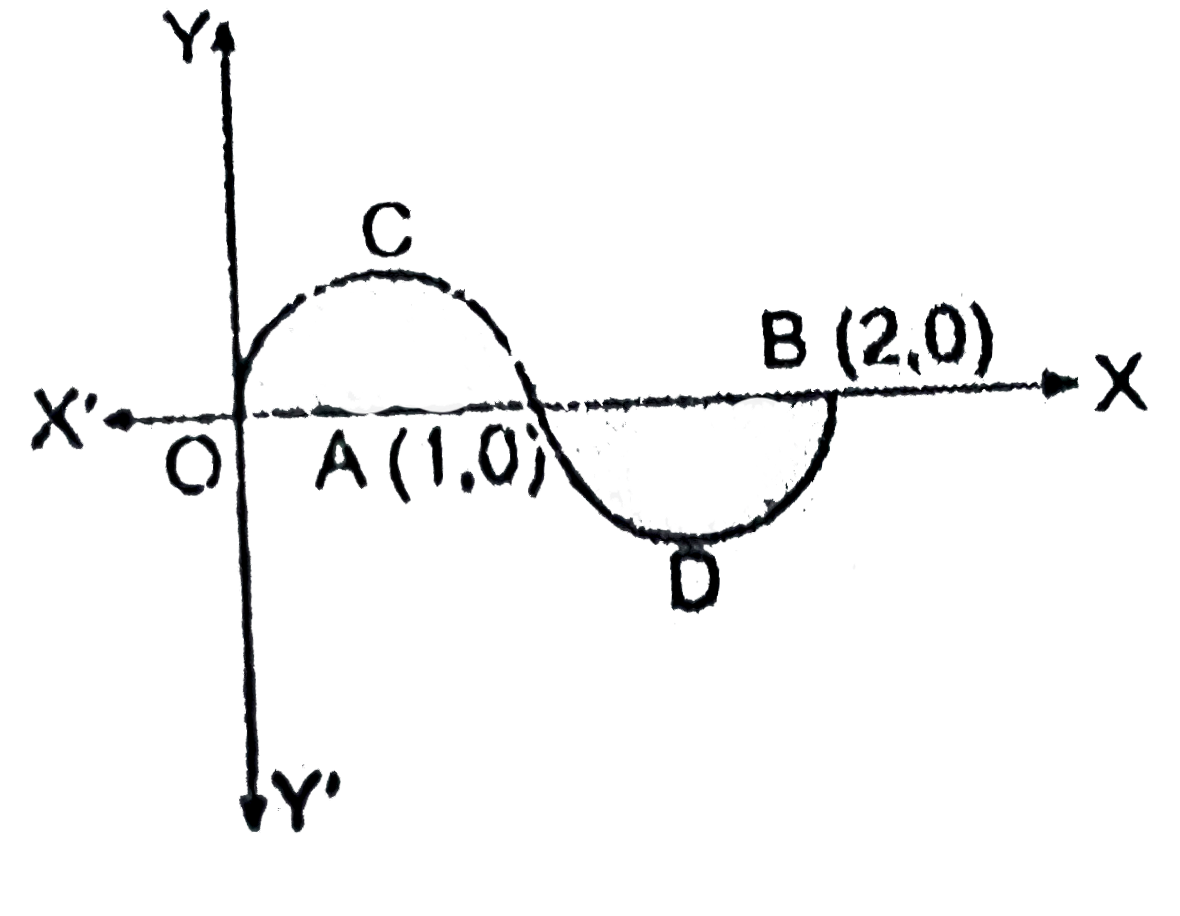
The given curve crosses the X-axis at points (0, 0), (1, 0) and (2, 0).
Its graph is shown in the figure.
Area of the region bounded by curve and X-axis
$=$ Area of OACO + Area of ADBA
$= \int_{0}^{1} y d x + \int_{1}^{2} (-y) d x$
$= \int_{0}^{1} 4 x (x - 1) (x - 2) d x - \int_{1}^{2} 4 x (x - 1) (x - 2) d x$
$= 4 \int_{0}^{1} (x^{3} - 3 x^{2} + 2 x) d x - 4 \int_{1}^{2} (x^{3} - 3 x^{2} + 2 x) d x$
$= [(\frac{1}{4} - 1 + 1) - (0 - 0 + 0)] - 4 [(4 - 8 + 4) - (\frac{1}{4} - 1 + 1)]$
$= 1 + 1 = 2 s q .$ units.

Area bounded by the curve $y = (x - 1) (x - 5)$ and the X-axis is
A $\frac{32}{3}$
B $\frac{31}{3}$
C $\frac{29}{3}$
D $\frac{28}{3}$
The area bounded by the curve $a^{2} y = x^{2} (x + a)$ and the x-axis is
A $a^{2} / 3$ sq. units
B $a^{2} / 4$ sq. units
C $3 a^{2} / 4$ sq. units
D $a^{2} / 12$ sq. units
Area bounded by the curve $y = x^{2} + x - 6$ and the X-axis is
A $\frac{127}{6}$
B $\frac{225}{6}$
C $\frac{125}{6}$
DNone of these