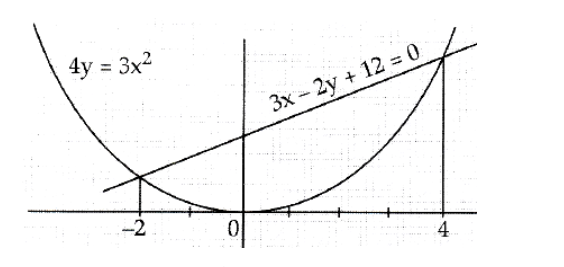
Find the area enclosed by the parabola $4 y = 3 x^{2}$ and the line $2 y = 3 x + 12 .$

Video Solution

Text Solution

Verified by Experts

$4 y = 3 x^{2}$ and $3 x - 2 y + 12 = 0$
or $4 (\frac{3 x + 12}{2}) = 3 x^{2}$
$= 3 x^{2} - 6 x - 24 = 0$
$= x^{2} - 2 x - 8 = 0$
$= (x - 4) (x + 2) = 0$
x-coordinates of points of intersection are $x = 2, x = 4$
Area(A)= $\int_{- 2}^{4} [\frac{1}{2} (3 x + 12) - \frac{3}{4} x^{2}] d x$
$= {[\frac{1}{2} \frac{{(3 x + 12)}^{2}}{6} - \frac{3}{4} . \frac{x^{3}}{3}]}_{- 2}^{4}$
$= 45 - 18 = 27$ sq. units

Updated on:21/07/2023