Find the area of the region bounded by the parabola y2=2pxandx2=2py.
The correct Answer is:N/a
We have, y2=2px and x2=2py
∴Y=√2px
⇒x2=2p.√2px
⇒x4=4p2.(2px)
⇒x4=8p3x
⇒x4−8p3x=0
⇒x3(x−8p3)=0
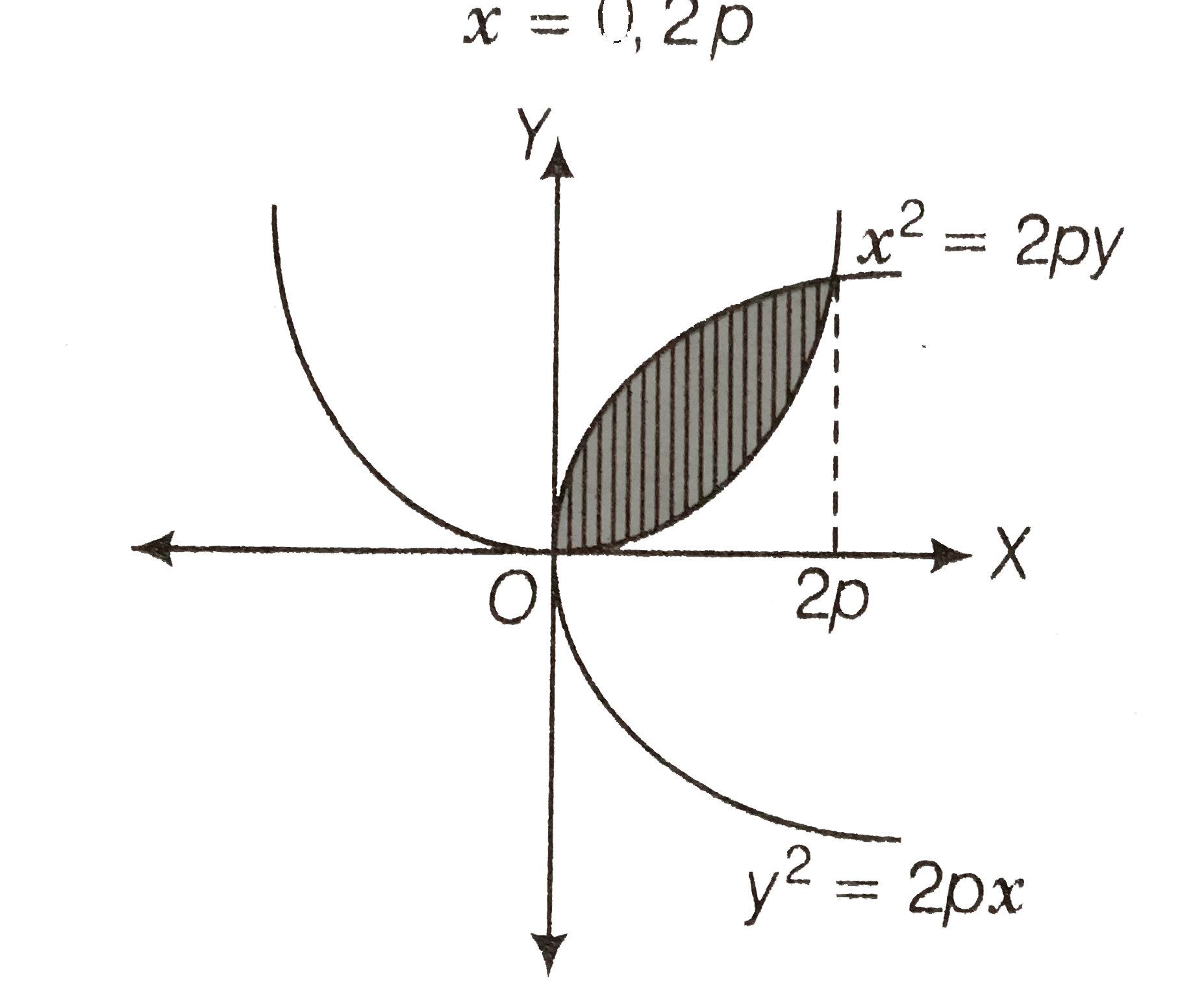
∴ Required area =∫2p0√2pxdx−∫2p0x22pdx
=√2p∫2p0x1/2dx−12pdx
=√2p[2(x)3/23]2p0−12p[x33]2p0
=√2p[23.(2p)3/2−0]−12p[13(2p)3−0]
=√sp(23.2p3/2)−12p(138p3)
=√2p(4√23p3/2)−12p(83p3)
=4√23.√2p2−86p2
(16−8)p26=8p26
=4p23 sq units
Topper's Solved these Questions
- APPLICATION OF INTEGRALSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Long Answer Type Questions
16 Videos
- APPLICATION OF INTEGRALSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Objective Type Questions
22 Videos
- APPLICATION OF DERIVATIVESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Fillers
5 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - True/False
10 Videos
Similar Questions
Find the area of the region bounded by the parabola y=x2 and y=|x| .
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x|.
View SolutionFind the area of the region bounded by the two parabolas y=x2and y2=x.
View SolutionUsing integration, find the area of the region bounded by the parabola y2=4x and the line x=4.
View SolutionDraw a rough sketch and find the area of the region bounded by the parabolas y2=4xandx2=4y, using the method of integration.
View SolutionFind the area of the region bounded by the curve y2=4x and x2=4y.
View SolutionFind the area of the region bounded by the curve y2=4x and x2=4y.
View SolutionFind the area of the region bounded by the parabola y2=2x and straight line x−y=4.
View SolutionThe area of the region bounded by the parabolas x=−2y2 and x=1−3y2 is
View SolutionUsing integration, find the area of the region bounded by the parabola y2=16x and the line x=4
View Solution
