Find the area of the region {(x,y):y2≤4x,4x2+4y2≤9}
Video Solution
Text Solution
Corrosponding equations of curves
y2=4x
and 4x2+4y2=9
On solving, the points of intersections are
A(12,√2) and B(12,−√2)
The centre is (0, 0) and radius is 32 of the given circle.
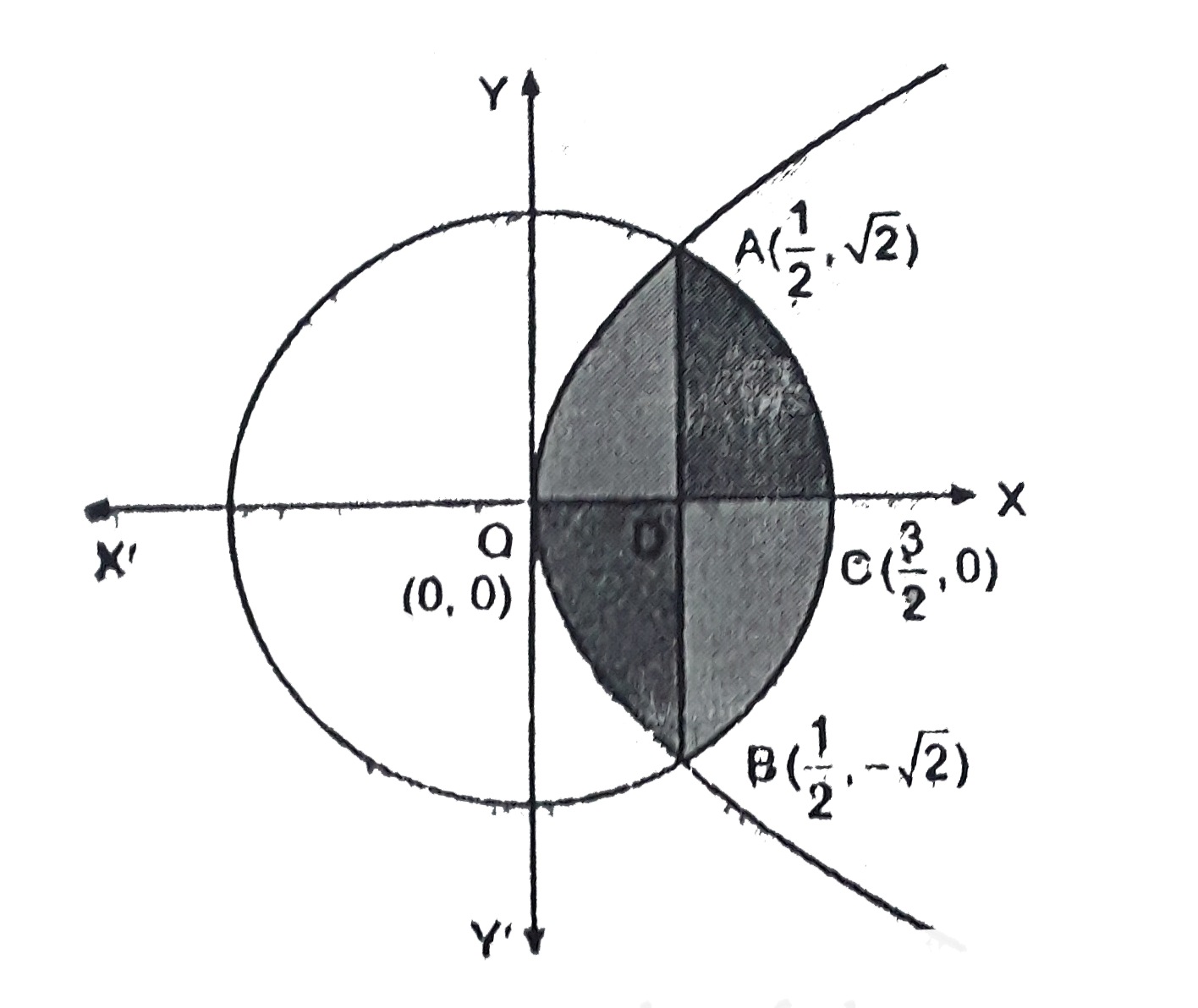
Now we draw the graphs of the curves. Using inequations, we find the required region.
Required region is symmetric about X-axis.
Required area
=2area of (ODAO)+2 area of (DCAD)
=∫1/202√xdx+2∫3/21/2√94−x2dx
=4⋅23[x3/2]1/20+2⎡⎢⎣x2√94−x2+98sin−1(x3/2]3/21/2
=83⋅12√2+2[(0+98sin−1(1))−(14√2+98sin−113)]
=43√2+9π8−√22−94sin−113 sq. units.
=4√26−√22+94(π2−sin−113)
=(√26+94cos−113) sq. units.
|
Topper's Solved these Questions
- APPLICATIONS OF INTEGRALSBOOK - NAGEEN PRAKASHANCHAPTER - APPLICATIONS OF INTEGRALSEXERCISE - Exercise 8.2
7 Videos
- APPLICATIONS OF DERIVATIVESBOOK - NAGEEN PRAKASHANCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Miscellaneous Exercise
24 Videos
- Continuity and DifferentiabilityBOOK - NAGEEN PRAKASHANCHAPTER - Continuity and DifferentiabilityEXERCISE - Miscellaneous Exercise
23 Videos
Similar Questions
Find the area of the region {(x,y):y2≤4x,4x2+4y2≤9}
View SolutionFind the area of the region {(x,y):y2≤4x,4x2+4y2≤9}
View SolutionFind the area of the region {(x,y):y2≤4x,4x2+4y2≤9}
View SolutionFind the areas of the region {x,y}:y2≤4x,4x2+4y2≤9}, using integration.
View SolutionFind the area of the region {(x; y):y2≤4xand 4x2+4y2≤9}.
View SolutionDraw the rough sketch and find the area of the region:
{(x,y):4x2+y2≤4,2x+y≥2}
View SolutionFind the area of the region bounded by y2=4x,x=1,x=4 and the x-axis in the first quadrant.
View SolutionFind the area of the region bouonded by y2=4x,x=1,x=4 and x-axis in the first quadrant.
View SolutionFind the area of the region bounded by the parabola y2=4x, the x-axis, and the lines x = 1 and x = 4.
View SolutionFind the area of the region bounded by the curves x2+y2=4 and (x−2)2+y2=4.
View Solution
