Find the Cartesian coordinates of the center of gravity of the are of the cardioid ρ=a(1+cosφ) between φ=0andφ=π.
The correct Answer is:(4a)/(5)
Topper's Solved these Questions
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Computing Pressure, Work and Other Physical Quantities by the Definite Integrals
7 Videos
- APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONSBOOK - IA MARONCHAPTER - APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONSEXERCISE - ADDITIONAL PROBLEMS
9 Videos
- BASIC CLASSES OF INTEGRABLE FUNCTIONS BOOK - IA MARONCHAPTER - BASIC CLASSES OF INTEGRABLE FUNCTIONS EXERCISE - 5.8 INTEGRATION OF OTHER TRANSCENDENTAL FUNCTIONS
5 Videos
Similar Questions
Find the Cartesian coordinates of the centre of gravity of the figure enclosed by the curve =acos3φ(a>0).
View SolutionFind the are length of the cardioid ρ=a(1+cosφ)(a>0,0≤φ≤2π)
View SolutionCompute the length of the segment of the straight line ρ=asec(φ−π3) between φ=0andφ=π2
View SolutionFind the area of the figure out only by the circle ρ=√3sinφ from the cardioid ρ=1+cosφ
View SolutionCompute the volume of the solid obtained by revolving about the polar axis the cardioid ρ=a(1+cosφ) shown in
View SolutionFind the length of the first turn of the spiral of the Archimedes ρ=aφ
View SolutionCompute the area of the figure bounded by the circle ρ=3√2acosφandρ=3asinφ
View SolutionFind the length of the curve φ=12(ρ+1/ρ) between ρ=2andρ=4
View SolutionComputes the area of the surface formed by revolving one branch of the lemniscate ρ=a√cos2φ about the straight line φ=π4
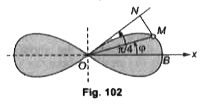
View Solutionφ2=0 represents
View Solution
