Find the equations the sides of an isosceles right angled triangle the equation of whose hypotenuse is $3 x + 4 y = 4$ and the opposite vertex is the point (2, 2).

Video Solution

Text Solution

Verified by Experts

The correct Answer is:N/a

Let slope of line AC be m and slope of line BC is $\frac{- 3}{4}$ and let angle between line AC and BC be $θ$
$∴ tan θ = ∣ ∣ ∣ ∣ \frac{m + \frac{3}{4}}{1 - \frac{3 m}{4}} ∣ ∣ ∣ ∣ \Rightarrow {tan 45}^{\circ} = \pm ⎡ ⎣ \frac{m + \frac{3}{4}}{1 - \frac{3 m}{4}} ⎤ ⎦$
Taking positive sign, $1 = \frac{m + \frac{3}{4}}{1 - \frac{3 m}{4}}$
$\Rightarrow m + \frac{3}{4} = 1 - \frac{3 m}{4}$
$\Rightarrow m + \frac{3 m}{4} = 1 - \frac{3}{4}$
$\Rightarrow \frac{7 m}{4} = \frac{1}{4} \Rightarrow m = \frac{1}{7}$
Taking negative sign, $1 = \frac{m + \frac{3}{4}}{1 - \frac{3 m}{4}}$
$\Rightarrow m + \frac{3}{4} = 1 - \frac{3 m}{4}$
$\Rightarrow m + \frac{3 m}{4} = 1 - \frac{3}{4}$
$\Rightarrow \frac{7 m}{4} = \frac{1}{4} \Rightarrow m = \frac{1}{7}$
Taking negative sign,
$1 = ⎛ ⎝ \frac{m + \frac{3}{4}}{1 - \frac{3 m}{4}} ⎞ ⎠ \Rightarrow 1 - \frac{3 m}{4} = - m - \frac{3}{4}$
$\Rightarrow m - \frac{3 m}{4} = - 1 - \frac{3}{4}$
$\Rightarrow \frac{3 m}{4} = \frac{- 7}{4} \Rightarrow m = - 7$
$∴$ Equation of side AC having slope $(\frac{1}{7})$ is
$y - 2 = \frac{1}{7} (x - 2)$
$\Rightarrow 7 y - 14 = x - 2$
$\Rightarrow x - 7 y + 12 = 0$
and equation of side AB having slope $(- 7)$ is
$y - 2 = - 7 (x - 2)$
$\Rightarrow y - 2 = - 7 x + 14$
$\Rightarrow 7 x + y - 16 = 0$

Updated on:21/07/2023

|

Class 11 MATHS STRAIGHT LINES

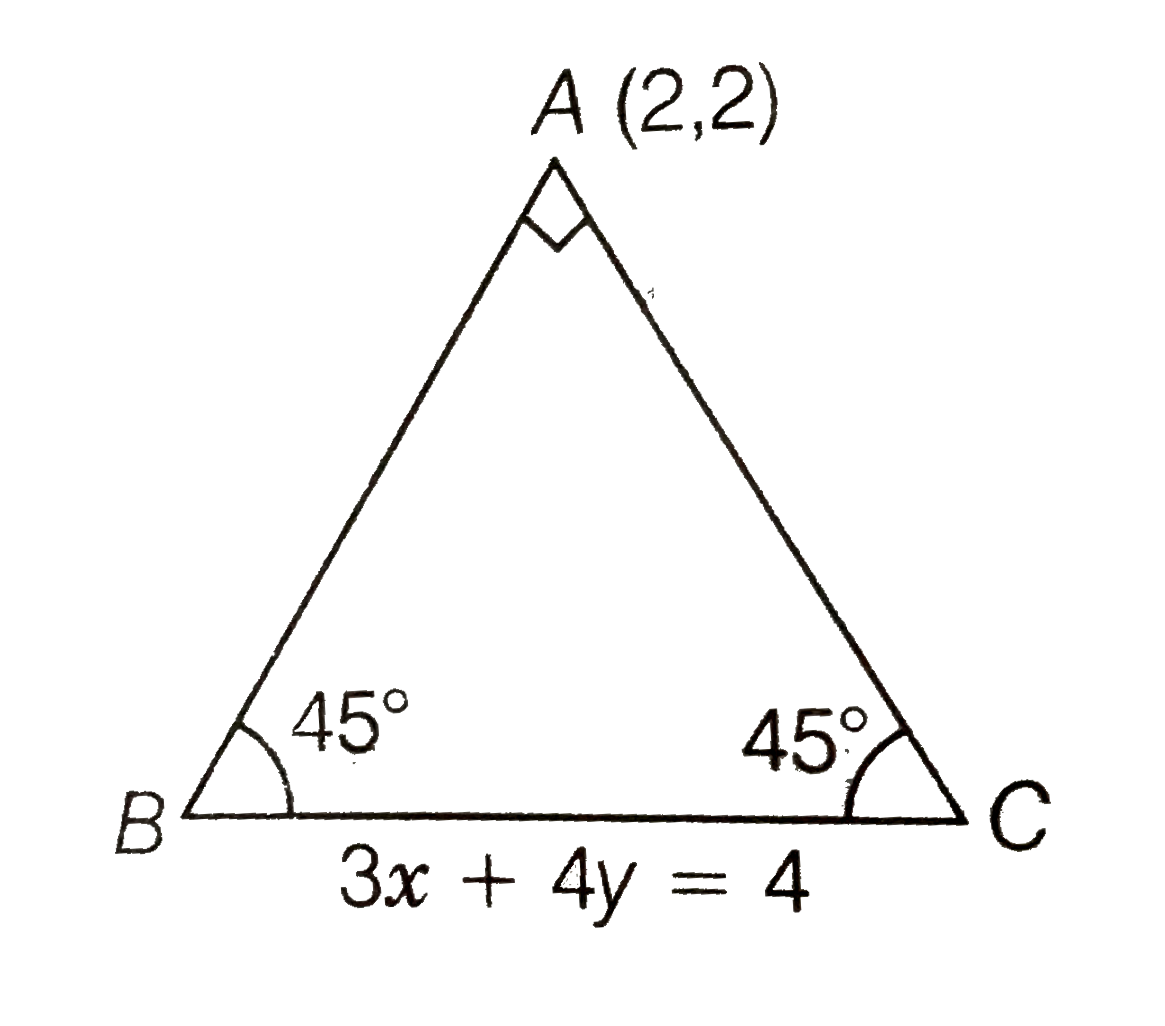
Topper's Solved these Questions

Similar Questions

NCERT EXEMPLAR ENGLISH-STRAIGHT LINES-MATCHING THE COLUMN

Home
Ask Doubt
Profile