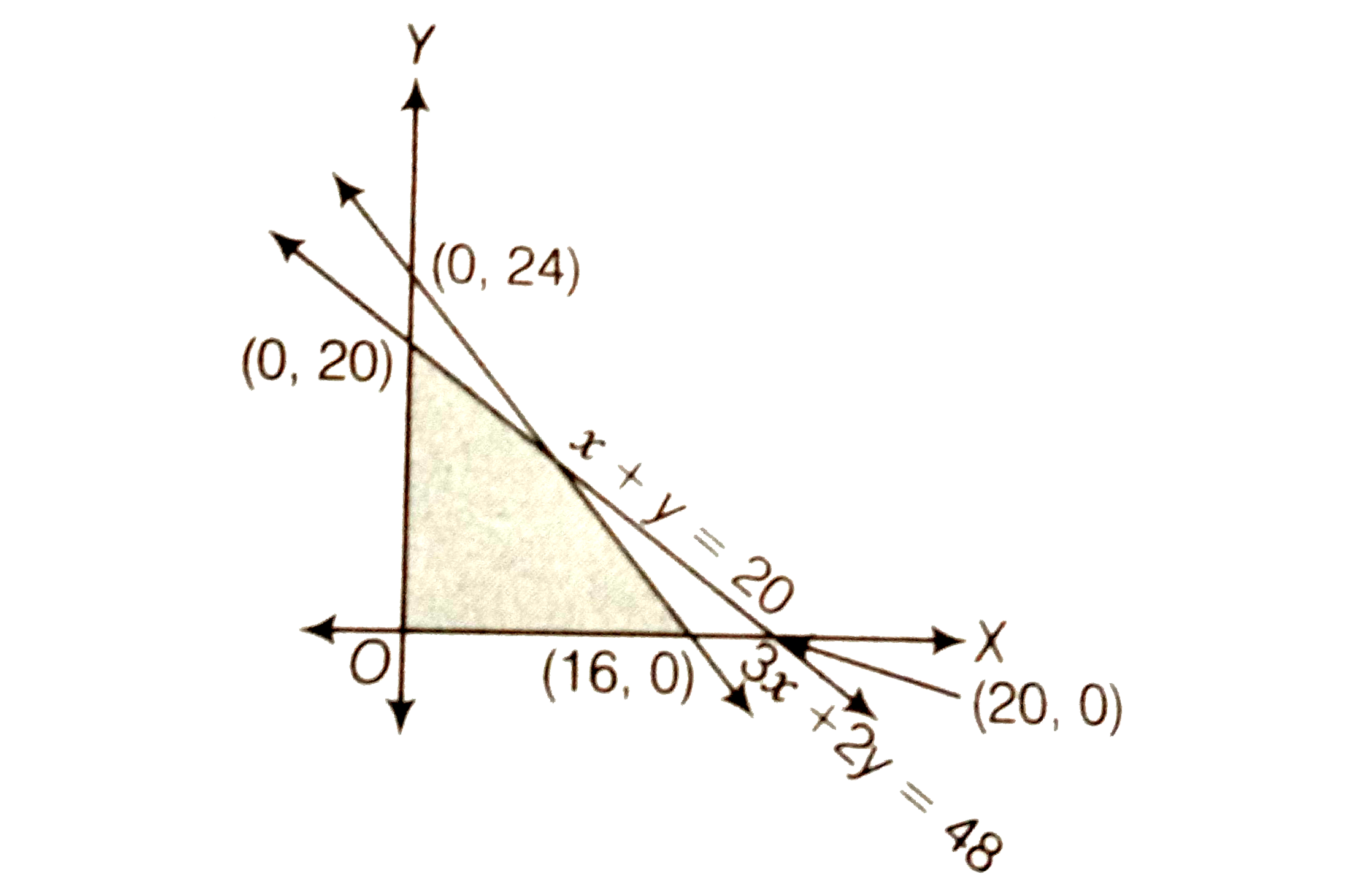
Find the linear inequations for which the shaded area in the figure given below is the solution set.
To find the linear inequalities corresponding to the shaded area in the figure, we will follow these steps:
Step 1: Identify the boundary lines
From the video transcript, we have two boundary lines:
1. x+y=21
2. 3x+2y=48
Step 2: Determine the inequalities
To find the inequalities, we need to analyze the regions defined by these lines.
1. For the line x+y=21:
- The shaded area is below this line, which means we have:
x+y≤21
2. For the line 3x+2y=48:
- The shaded area is below this line as well, which gives us:
3x+2y≤48
Step 3: Consider the constraints from the axes
Since the shaded area is in the first quadrant (where both x and y are non-negative), we have the following constraints:
1. x≥0
2. y≥0
Final Set of Inequalities
Combining all the inequalities, we have the following system:
1. x+y≤21
2. 3x+2y≤48
3. x≥0
4. y≥0
Summary
The linear inequalities that represent the shaded area in the figure are:
1.x+y≤212.3x+2y≤483.x≥04.y≥0
From the video transcript, we have two boundary lines:
1. x+y=21
2. 3x+2y=48
To find the inequalities, we need to analyze the regions defined by these lines.
- The shaded area is below this line, which means we have:
x+y≤21
- The shaded area is below this line as well, which gives us:
3x+2y≤48
Since the shaded area is in the first quadrant (where both x and y are non-negative), we have the following constraints:
1. x≥0
2. y≥0
Combining all the inequalities, we have the following system:
1. x+y≤21
2. 3x+2y≤48
3. x≥0
4. y≥0
The linear inequalities that represent the shaded area in the figure are:
1.x+y≤212.3x+2y≤483.x≥04.y≥0
Similar Questions
Find the linear inequalities for which the shaded region in the given figure is the solution set.
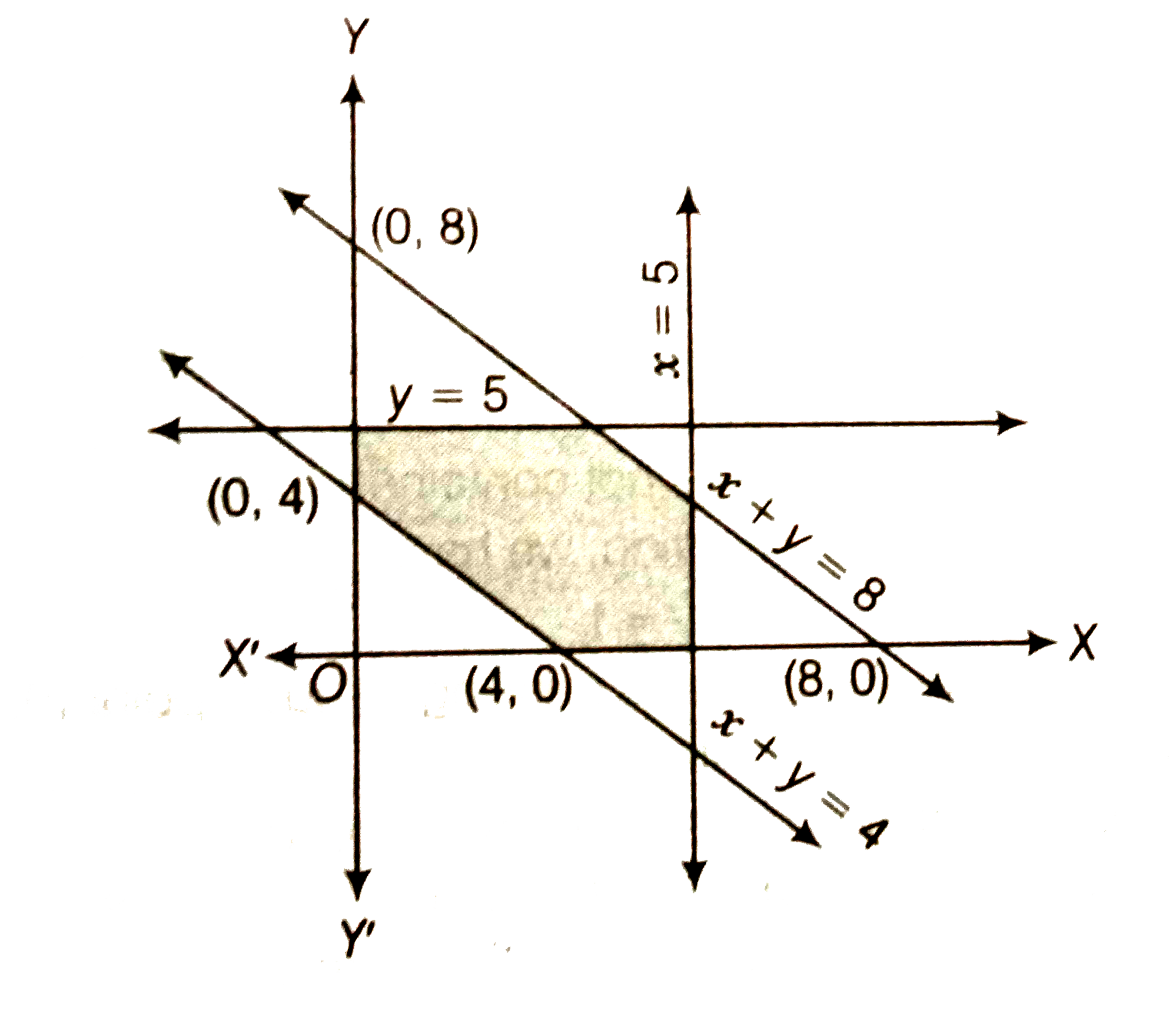
View SolutionFind the linear inequalities for which the shaded region in the given figure is the solution set.
View SolutionFind the linear inequations for which the shaded area in fig. 15.41 is the solution set. Draw the diagram of the solution set of the linear inequations.
View SolutionFind the area of the figure given below.

View SolutionFind the linear inequations for which the shaded region in fig. 15.40 is the solution set.
View SolutionFind the linear equations for which the shaded area in Fig: 15.39 is the solution set.
View SolutionThe shaded region in the given figure is
View SolutionThe shaded region in the given figure is
View SolutionFind the linear inequations for which the solution set is the shaded region given in fig. 15.42.
View SolutionCalculate the area of the figure given below : which is not drwn to scale.
View Solution
