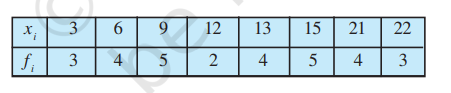
Find the mean deviation about the median for the data : xi: 5 7 9 10 12 15. fi: 8 6 2 2 2 6
To find the mean deviation about the median for the given data, we will follow these steps:
Step 1: Organize the Data
We have the data points xi and their corresponding frequencies fi:
- xi:5,7,9,10,12,15
- fi:8,6,2,2,2,6
Step 2: Calculate the Total Frequency
Calculate the total frequency Σfi:
Σfi=8+6+2+2+2+6=26
Step 3: Find the Cumulative Frequency
Next, we find the cumulative frequency to determine the median position:
- For x1=5: Cumulative Frequency = 8
- For x2=7: Cumulative Frequency = 8 + 6 = 14
- For x3=9: Cumulative Frequency = 14 + 2 = 16
- For x4=10: Cumulative Frequency = 16 + 2 = 18
- For x5=12: Cumulative Frequency = 18 + 2 = 20
- For x6=15: Cumulative Frequency = 20 + 6 = 26
Step 4: Determine the Median
The median is located at the 262=13th and 14th positions. From the cumulative frequency:
- The 13th term falls in the range of x2=7 (cumulative frequency = 14).
- The 14th term also falls in the range of x2=7.
Thus, the median m is:
m=7
Step 5: Calculate |xi−m|
Now, we calculate |xi−m| for each xi:
- For x1=5: |5−7|=2
- For x2=7: |7−7|=0
- For x3=9: |9−7|=2
- For x4=10: |10−7|=3
- For x5=12: |12−7|=5
- For x6=15: |15−7|=8
Step 6: Calculate fi⋅|xi−m|
Now, we multiply each |xi−m| by its corresponding frequency fi:
- For x1=5: 8⋅2=16
- For x2=7: 6⋅0=0
- For x3=9: 2⋅2=4
- For x4=10: 2⋅3=6
- For x5=12: 2⋅5=10
- For x6=15: 6⋅8=48
Step 7: Calculate the Total of fi⋅|xi−m|
Now, sum these products:
16+0+4+6+10+48=84
Step 8: Calculate the Mean Deviation
Finally, the mean deviation about the median is given by:
Mean Deviation=Σfi⋅|xi−m|Σfi=8426≈3.23
Final Answer
The mean deviation about the median for the given data is approximately 3.23.
---
We have the data points xi and their corresponding frequencies fi:
- xi:5,7,9,10,12,15
- fi:8,6,2,2,2,6
Calculate the total frequency Σfi:
Σfi=8+6+2+2+2+6=26
Next, we find the cumulative frequency to determine the median position:
- For x1=5: Cumulative Frequency = 8
- For x2=7: Cumulative Frequency = 8 + 6 = 14
- For x3=9: Cumulative Frequency = 14 + 2 = 16
- For x4=10: Cumulative Frequency = 16 + 2 = 18
- For x5=12: Cumulative Frequency = 18 + 2 = 20
- For x6=15: Cumulative Frequency = 20 + 6 = 26
The median is located at the 262=13th and 14th positions. From the cumulative frequency:
- The 13th term falls in the range of x2=7 (cumulative frequency = 14).
- The 14th term also falls in the range of x2=7.
m=7
Now, we calculate |xi−m| for each xi:
- For x1=5: |5−7|=2
- For x2=7: |7−7|=0
- For x3=9: |9−7|=2
- For x4=10: |10−7|=3
- For x5=12: |12−7|=5
- For x6=15: |15−7|=8
Now, we multiply each |xi−m| by its corresponding frequency fi:
- For x1=5: 8⋅2=16
- For x2=7: 6⋅0=0
- For x3=9: 2⋅2=4
- For x4=10: 2⋅3=6
- For x5=12: 2⋅5=10
- For x6=15: 6⋅8=48
Now, sum these products:
16+0+4+6+10+48=84
Finally, the mean deviation about the median is given by:
Mean Deviation=Σfi⋅|xi−m|Σfi=8426≈3.23
The mean deviation about the median for the given data is approximately 3.23.
Topper's Solved these Questions
- BOOK - NCERTCHAPTER - STATISTICSEXERCISE - EXERCISE 15.35videos
STATISTICS
- BOOK - NCERTCHAPTER - STATISTICSEXERCISE - EXERCISE 15.210videos
STATISTICS
- BOOK - NCERTCHAPTER - STATISTICSEXERCISE - SOLVED EXAMPLES18videos
STATISTICS
- BOOK - NCERTCHAPTER - SETSEXERCISE - EXERCISE 1.57videos
SETS
- BOOK - NCERTCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE 10.52videos
STRAIGHT LINES
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the mean deviation about median for the following data.
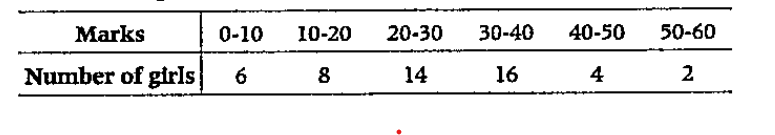 A10.34B10.50C10.40D10.37
A10.34B10.50C10.40D10.37 - Question 2 - Select One
What is the mean deviation about the mean for the data 4, 7, 8, 9, 10, 12, 13, 17?
A2.5B3C3.5D4
Find the mean deviation about the median for the data in Question
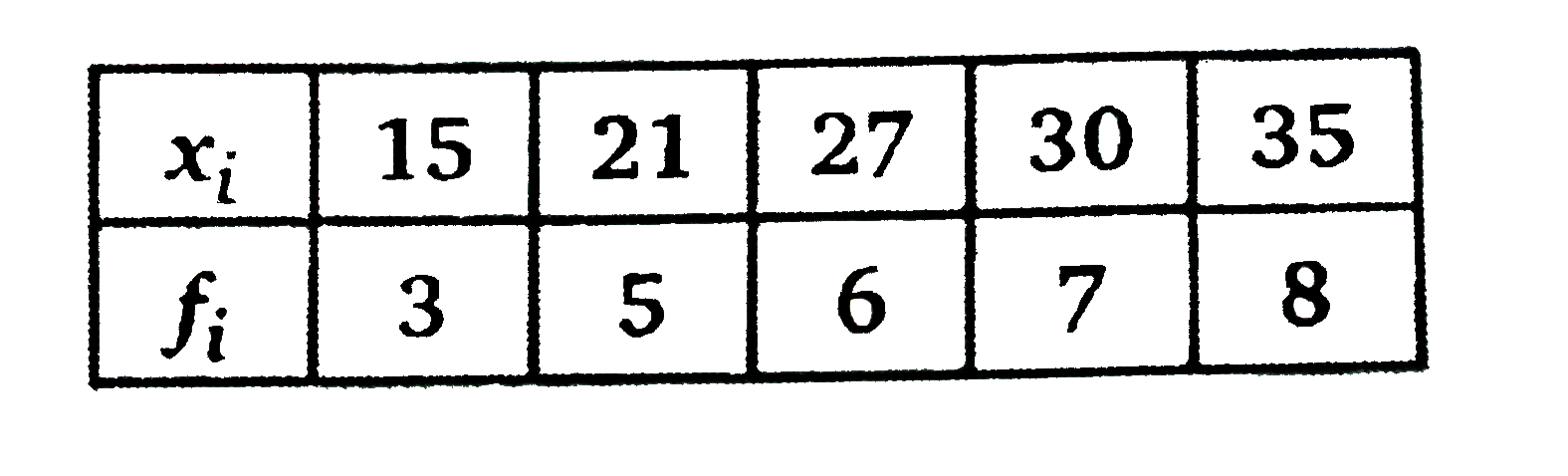 View Solution
View SolutionFind the mean deviation about median for the following data:
 View Solution
View SolutionFind the mean deviation about the median for the data : xi 15 21 27 30 35 fi 3 5 6 7 8
View SolutionFind the mean deviation about the mean for the data :
 View Solution
View SolutionFind the mean deviation about the mean for the data :xi 10 30 50 70 90fi 4 24 28 16 8
View SolutionFind the mean deviation about the mean for the data : 4,7,8,9,10,12,13,17
View Solution