Find the mode of the following distribution of marks obtained by 80 students: Marks obtained 0-10 10-20 20-30 30-40 40-50 No. of students 6 10 12 32 20
To find the mode of the given distribution of marks obtained by 80 students, we will follow these steps:
Step 1: Identify the data
We have the following data:
| Marks obtained | No. of students |
|----------------|-----------------|
| 0 - 10 | 6 |
| 10 - 20 | 10 |
| 20 - 30 | 12 |
| 30 - 40 | 32 |
| 40 - 50 | 20 |
Step 2: Determine the modal class
The modal class is the class with the highest frequency. From the table, we can see that the highest frequency is 32, which corresponds to the class interval 30 - 40.
Step 3: Assign values for the mode formula
The formula for calculating the mode in a grouped frequency distribution is:
Mode=L+(H⋅(F−F1)(2F−F1−F2))
Where:
- L = lower boundary of the modal class
- H = width of the modal class
- F = frequency of the modal class
- F1 = frequency of the class preceding the modal class
- F2 = frequency of the class succeeding the modal class
From our data:
- L=30 (lower boundary of the modal class 30 - 40)
- H=10 (width of the class interval, which is 40 - 30)
- F=32 (frequency of the modal class)
- F1=12 (frequency of the class 20 - 30)
- F2=20 (frequency of the class 40 - 50)
Step 4: Substitute the values into the formula
Now, substituting the values into the mode formula:
Mode=30+(10⋅(32−12)(2⋅32−12−20))
Step 5: Calculate the numerator and denominator
1. Calculate F−F1:
32−12=20
2. Calculate the numerator:
10⋅20=200
3. Calculate 2F−F1−F2:
2⋅32−12−20=64−12−20=32
Step 6: Complete the calculation
Now substitute back into the formula:
Mode=30+(20032)
Calculating 20032:
20032=6.25
Thus, we have:
Mode=30+6.25=36.25
Final Answer
The mode of the distribution is 36.25.
---
We have the following data:
|----------------|-----------------|
| 0 - 10 | 6 |
| 10 - 20 | 10 |
| 20 - 30 | 12 |
| 30 - 40 | 32 |
| 40 - 50 | 20 |
The modal class is the class with the highest frequency. From the table, we can see that the highest frequency is 32, which corresponds to the class interval 30 - 40.
The formula for calculating the mode in a grouped frequency distribution is:
- L = lower boundary of the modal class
- H = width of the modal class
- F = frequency of the modal class
- F1 = frequency of the class preceding the modal class
- F2 = frequency of the class succeeding the modal class
- L=30 (lower boundary of the modal class 30 - 40)
- H=10 (width of the class interval, which is 40 - 30)
- F=32 (frequency of the modal class)
- F1=12 (frequency of the class 20 - 30)
- F2=20 (frequency of the class 40 - 50)
Now, substituting the values into the mode formula:
1. Calculate F−F1:
32−12=20
10⋅20=200
2⋅32−12−20=64−12−20=32
Now substitute back into the formula:
20032=6.25
Mode=30+6.25=36.25
The mode of the distribution is 36.25.
Topper's Solved these Questions
Similar Questions
Find the mode of the following frequency distribution of marks obtained by 50 students. Marks obtained 0−10 10−20 20−30 30−40 40−50 No. of students 5 12 20 10 3
View SolutionFind the mode of the following marks (out of 10) obtained by 20 students:4, 6, 5, 9, 3, 2, 7, 7, 6, 5, 4, 9, 10, 10, 3, 4, 7, 6, 9, 9
View SolutionFind the mode of the following data: Class Frequency 0-20 15 20-40 6 40-60 18 60-80 10
View SolutionIf for somex∈R, the frequency distribution of the marks obtained by 20 students in a test is:
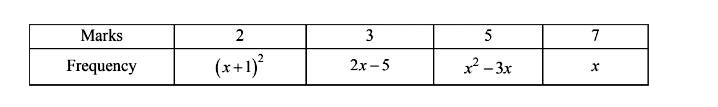
Then the mean of the marks is:
View SolutionFind the mean of the following frequency distributions: Class interval: 0-10 10-20 20-30 30-40 40-50 Frequency: 9 12 15 10 14
View SolutionFind the mean of the following frequency distribution, using step-deviation method: Class 0-10 10-20 20-30 30-40 40-50 Frequency 7 12 13 10 8
View SolutionFind the mean of the following frequency distribution using step-deviation method. Class 0-10 10-20 20-30 30-40 40-50 Frequency 7 10 15 8 10
View SolutionFind the mean of the following frequency distribution: Class-interval: 0-10 10-20 20-30 30-40 40-50 No. of workers f: 7 10 15 8 10
View SolutionFind the mode of the following distribution: (i) Class-interval: 0-10 10-20 20-30 30-40 40-50 50-60 60-70 70-80 Frequency: 5 8 7 12 28 20 10 10
View SolutionIf the mean of the following distribution is 27, find the value of p . Class: 0-10 10-20 20-30 30-40 40-50 Frequency: 8 p 12 13 10
View Solution
