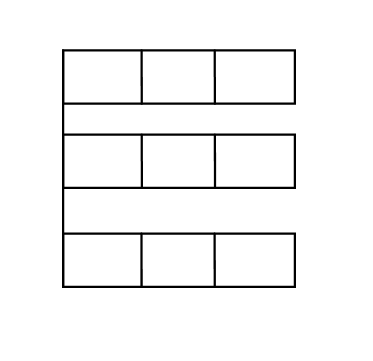
Find the number of ways in which letters A, A, A, B, B, B can be placed in the squares of the figure so that no row remains empty.

row block has to be selected.
One row out of 1st, 3rd and 5th will contain two letters and remaining one letter each.
Selection of one row that contains two letters is done in ways.
Now two blocks can be selected from this row in ways.
From each of reamaining two row, one blocks can be selected in ways.
Hence total number of selections
In each selection of six places, letters A, A, A, B,B, B can be arranged in
So, total number of ways

Similar Questions
Find the number of ways in which the letters A, B, C, D, E, F can be placed in the 8 boxes of the given figure so that no row remains empty. (figure)
View SolutionFind the number of ways in which the letters A, B, C, D, E, F can be placed in the 8 boxes of the given figure so that no row remains empty. (figure)
View SolutionFind the number of ways in which A A A B B B can be places in the square of figure as shown, so that no row remains empty is?
View SolutionThe number of ways in which the letters of the word PERSON can placed in the squares of the given figure so that no row remains empty is a. 24×6! b. 26×6! c. 26×7! d. 27×6!
View SolutionThe number of ways in which the letters of the word PERSON can placed in the squares of the given figure so that no row remains empty is a. 24×6! b. 26×6! c. 26×7! d. 27×6!
View SolutionThe number of ways the letters of the word PERSON cann be placed in the squares of the figure shown so that no row remain empty is

View SolutionThe number of ways the letters of the word PERSON cann be placed in the squares of the figure shown so that no row remain empty is

View SolutionThe number of ways in which 5 X's can be placed in the squares of the figure so that no row remains empty is:
View SolutionThe number of ways in which 5 X's can be placed in the squares of the figure so that no row remains empty is:
View SolutionThe number of ways that the letters of the word ''PERSON'' can be placed in the squares of the adjoining figure so that no row remains empty
View Solution