Find the straight line passing through the point of intersection of lines 2x+3y+5=0 and 5x-2y-16=0 and through the point (-1,3) using the concept of family of lines. Solution in Tamil
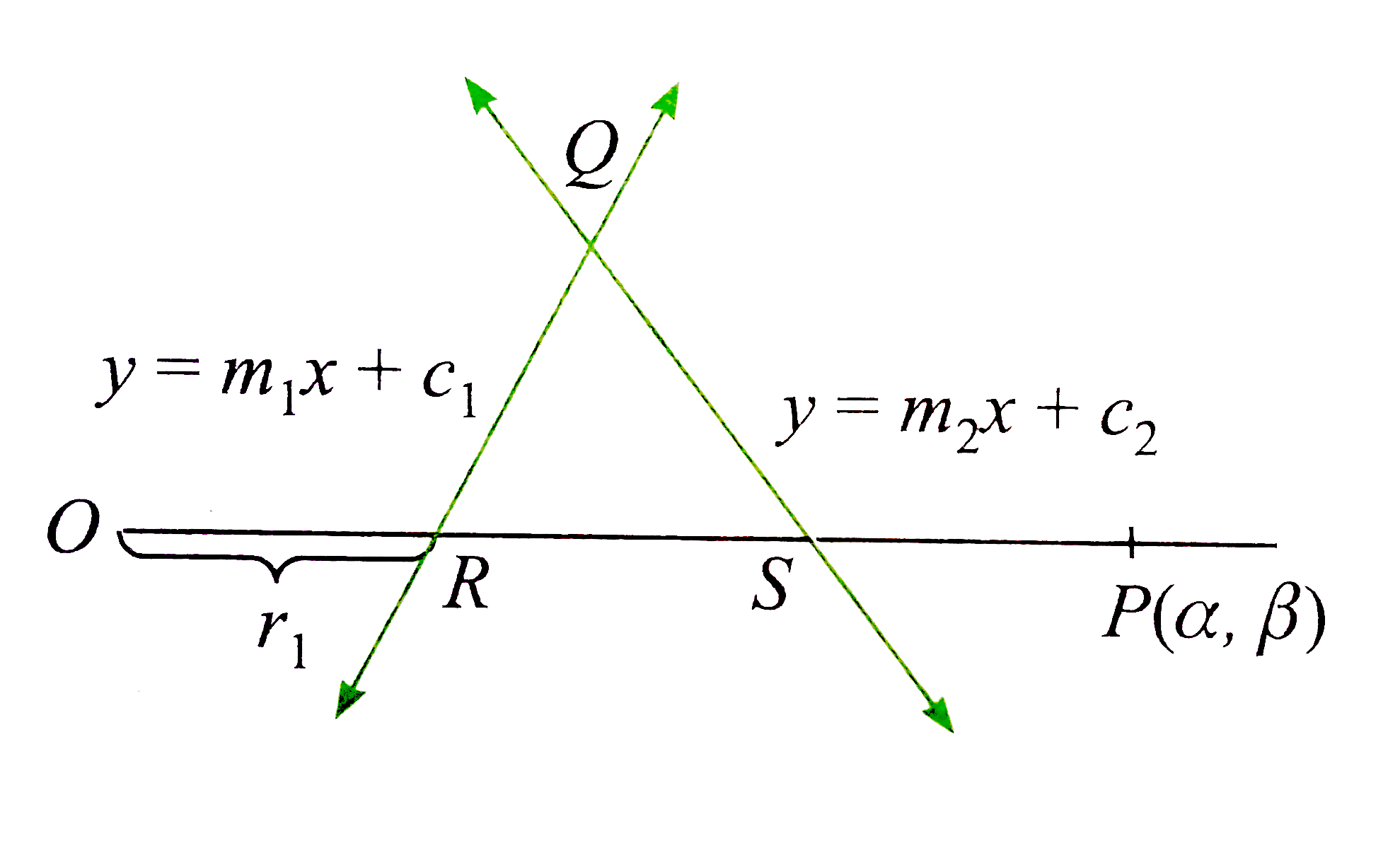
Let the variable line through O makes an angle θ with the positive direction of the x-axis. Any point on this variable line is (O+rcosθ,0+rsinθ)
i.e.,(rcosθ,rsinθ)
Let the fixed lines be
y=m1x+c1 (1)
and y=m2x+c2 (2)
Let OR=r1,OS=r2,OP=r3. So, according to the equation,
m+nr3=mr1+mr2 (3)
Since R and S lie on lines (1) and (2), respectively, we have
r1sinθ=m1(r1cosθ)+c1 (4)
and r2sinθ=m2(r2cosθ)+c2 (5)
LetP≡(α,β). Then,
α=r3cosθ,β=r3sinθ (6)
From (3), (4), and (5), we have
m+nr3=msinθ−m1cosθc1=+nsinθ−m2cosθc2 ltrgt or m+n=mc1(β−m1α)+nc2(β−m2α) [From(6)]
Hence, the locus of P is
m+n=(mc1+nc2)y−(mm1c1+nm2c2)x
or y−m1x−c1+nmc1c2(y−m2x−c2)=0 (7)
Clearly, the locus of P is a straight line passing through the point of intersection of the given lines (1) and (2).
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.1
23 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.2
4 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
Find the equation of the right bisector of the line segment joining the points (3,4) and (−1,2).
View SolutionFind the points on the line x + y = 1 that lie at a distance 3 units from the line 5x+12y = 3.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionQ. Let p and q real number such that p≠0,p2≠q and p2≠−q. if α and β are non-zero complex number satisfying α+β=−p and α3+β3=q, then a quadratic equation having αβ and βα as its roots is
View SolutionFind the straight line passing through the point of intersection of 2x+3y+5=0,5x−2y−16=0 , and through the point (−1,3).
View Solutionरेखाओं 5x−3y=1 और 2x+3y−23=0 के प्रतिच्छेद बिंदु से होकर जाने वाली उस रेखा की समीकरण ज्ञात कीजिए जो रेखा 5x−3y−1=0 पर लंब है ।
View SolutionThe straight line passing through the point of intersection of the straight line x+2y−10=0and2x+y+5=0 is
View SolutionFind the equation of the pair of straight lines passing through the point (1,3) and perpendicular to the lines 2x-3y+1=0 and 5x+y-3=0.
View Solutionसरल रेखा 5x+4y=0, सरल रेखाओं x+2y−10=0 और 2x+y+5=0 के प्रतिच्छेद बिन्दु से होकर जाती है ।
View SolutionThe line 5x + 4y = 0 passes through the point of intersection of straight lines (1) x+2y-10 = 0, 2x + y =-5
View SolutionThe line 5x + 4y = 0 passes through the point of intersection of straight lines x+2y-10 = 0, 2x + y =-5
View SolutionFind the straight line passing through the point of intersection of 2x+3y+5=0,5x−2y−16=0 , and through the point (−1,3).
View SolutionFind the straight line passing through the point of intersection of 2x+3y+5=0,5x−2y−16=0 , and through the point (−1,3).
View SolutionThe straight line passing through the point of intersection of the straight line x+2y−10=0and2x+y+5=0 is
View SolutionThe straight line passing through the point of intersection of the straight line x+2y−10=0and2x+y+5=0 is
View SolutionThe line 5x + 4y = 0 passes through the point of intersection of straight lines (1) x+2y-10 = 0, 2x + y =-5
View Solution
