Following are the car parking charges near a railway station up to Check if the parking charges are in direct proportion to the parking time.
To determine if the parking charges are in direct proportion to the parking time, we will analyze the given data step by step.
Given Data:
- For 4 hours: ₹60
- For 8 hours: ₹100
- For 12 hours: ₹140
- For 24 hours: ₹180
Step 1: Identify the relationship
We need to check if the parking charges increase as the parking time increases. In direct proportion, if one quantity increases, the other should also increase.
Step 2: Calculate the increase in time and charges
- From 4 hours to 8 hours:
- Time increase = 8 - 4 = 4 hours
- Charges increase = 100 - 60 = ₹40
- From 8 hours to 12 hours:
- Time increase = 12 - 8 = 4 hours
- Charges increase = 140 - 100 = ₹40
- From 12 hours to 24 hours:
- Time increase = 24 - 12 = 12 hours
- Charges increase = 180 - 140 = ₹40
Step 3: Analyze the increases
- The increase in parking charges for the first two intervals (4 to 8 hours and 8 to 12 hours) is ₹40 for every 4 hours.
- The increase in parking charges from 12 to 24 hours is ₹40 for an increase of 12 hours.
Step 4: Check for consistency
- The ratio of charges to time for the first two intervals is consistent:
- For 4 to 8 hours: ₹40/4 hours = ₹10 per hour
- For 8 to 12 hours: ₹40/4 hours = ₹10 per hour
- The ratio for the last interval (12 to 24 hours) is:
- For 12 to 24 hours: ₹40/12 hours = ₹3.33 per hour
Conclusion
Since the ratio of charges to time is not consistent (it changes from ₹10 per hour to ₹3.33 per hour), we can conclude that the parking charges are not in direct proportion to the parking time.
- For 4 hours: ₹60
- For 8 hours: ₹100
- For 12 hours: ₹140
- For 24 hours: ₹180
We need to check if the parking charges increase as the parking time increases. In direct proportion, if one quantity increases, the other should also increase.
- From 4 hours to 8 hours:
- Time increase = 8 - 4 = 4 hours
- Charges increase = 100 - 60 = ₹40
- Time increase = 12 - 8 = 4 hours
- Charges increase = 140 - 100 = ₹40
- Time increase = 24 - 12 = 12 hours
- Charges increase = 180 - 140 = ₹40
- The increase in parking charges for the first two intervals (4 to 8 hours and 8 to 12 hours) is ₹40 for every 4 hours.
- The increase in parking charges from 12 to 24 hours is ₹40 for an increase of 12 hours.
- The ratio of charges to time for the first two intervals is consistent:
- For 4 to 8 hours: ₹40/4 hours = ₹10 per hour
- For 8 to 12 hours: ₹40/4 hours = ₹10 per hour
- The ratio for the last interval (12 to 24 hours) is:
- For 12 to 24 hours: ₹40/12 hours = ₹3.33 per hour
Since the ratio of charges to time is not consistent (it changes from ₹10 per hour to ₹3.33 per hour), we can conclude that the parking charges are not in direct proportion to the parking time.
Topper's Solved these Questions
- BOOK - NCERT ENGLISHCHAPTER - DIRECT AND INVERSE PROPORTIONSEXERCISE - SOLVED EXAMPLES8videos
DIRECT AND INVERSE PROPORTIONS
- BOOK - NCERT ENGLISHCHAPTER - DATA HANDLINGEXERCISE - SOLVED EXAMPLES1videos
DATA HANDLING
- BOOK - NCERT ENGLISHCHAPTER - EXPONENTS AND POWERSEXERCISE - SOLVED EXAMPLES9videos
EXPONENTS AND POWERS
Similar Questions
Statement 1: If a proton and an α-particle enter a uniform magnetic field perpendicularly with the same speed, the time period of revolution of α-particle is double than that of proton.
Statement 2: In a magnetic field, the period of revolution of a charged particle is directly proportional to the mass of the particle and inversely proprotional to the charge of particle.View SolutionStatement 1: If a proton and an α-particle enter a uniform magnetic field perpendicularly with the same speed, the time period of revolution of α-particle is double than that of proton.
Statement 2: In a magnetic field, the period of revolution of a charged particle is directly proportional to the mass of the particle and inversely proprotional to the charge of particle.View Solution(A): Electric and gravitational fields are acting along same line. When proton and α-particle are projected up vertically along that line, the time of flight is less for proton.
(R ): In the given electric field acceleration of a charged particle is directly proportional to specific chargeView SolutionIn the given circuit, the capacitor of capacitance C is charged by closing key K at t = 0. Find the time required to charge the capacitor up to the maximum charge for the given circuit, if it were to be charged with the charged with constant initial charging rate at t=0 in the given circuit.
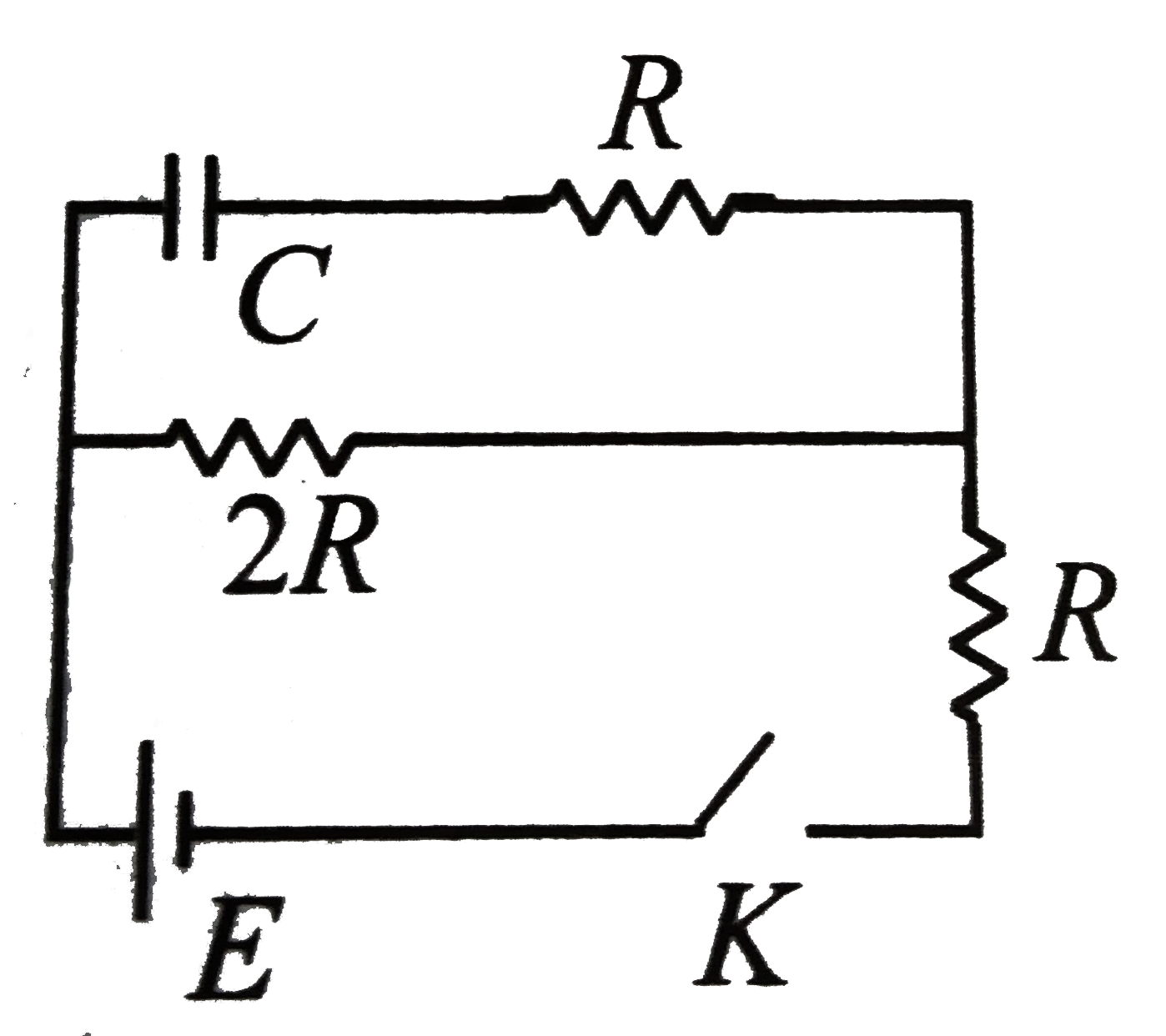 View Solution
View SolutionAn electric dipole consists of charges ±2.0×108C separated by a distance of 2.0×10−3 m. It is placed near a long ilne charge of linear charge density 4.0×10−4Cm−1 as shown in figure (30-W4), Such thet the negative charge is at a distance of 2.0 cm from the line charge. Find the force acting on the dipole.
 View Solution
View SolutionAn electric dipole consists of charges ±2.0×10−8C separated by a distance of 2.0×10−3 m. It is placed near a long line charge of linear charge density 4.0×10−4Cm−1 as shown in figure (30-W4), Such that the negative charge is at a distance of 2.0 cm from the line charge. Find the force acting on the dipole.
 View Solution
View SolutionA uniformly charged sphere, made up of non-conducting material and carrying charge Q, is placed near a uniformly charged non-conducting rod, carrying charge q. The centre of the sphere lies on the perpendicular bisector of the rod as shown in the figure. Calculate the force exerted by the sphere on the rod.
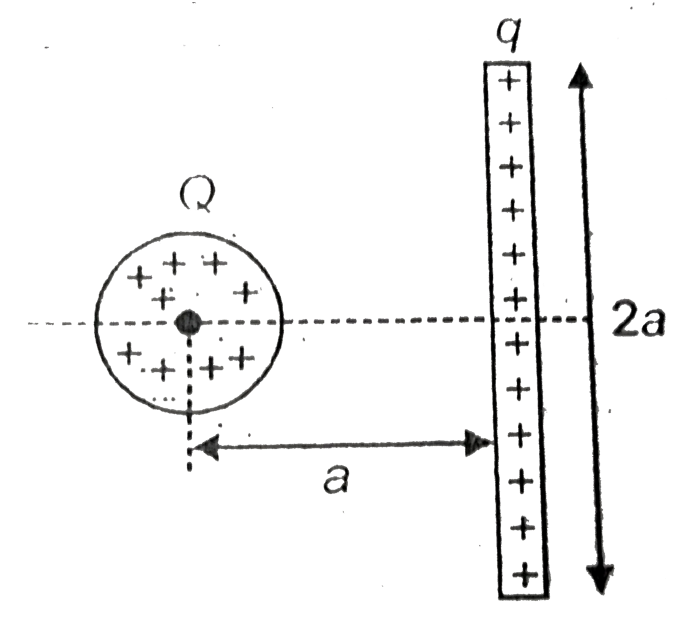 View Solution
View SolutionThe length of a rectangular park is three times its breadth. If the perimeter of the park is 192 meters, find the dimensions of the park.
View SolutionA capacitor of capacitance 10mF is charged up a potential difference of 2V and then the cell is removed. Now it is connected to a cell of emf4V and is charged fully. In both cases the polarities of the two cells are in the same directions. Total heat produced in the second charging process is :
View SolutionAccording to a FIR in police station, car A was at rest waiting for a red light at a crossing when it was hit by similar car B from rear side. Both cars had then hard brekes on, and from their skid marks it is surmiesed that they skidded together about 8m in the original direction of travel before coming to rest. Assuming a stopping force of about 0.4 times the combined weights of the cars. the speed of car B just before the collision is approximately [g=10m/s2]
View Solution

