From a point R(5,8) , two tangents RPandRQ are drawn to a given circle S=0 whose radius is 5. If the circumcenter of triangle PQR is (2, 3), then the equation of the circle S=0 is (a)x2+y2+2x+4y−20=0 (b) x2+y2+x+2y−10=0 (c) x2+y2−x+2y−20=0 (d )x2+y2+4x−6y−12=0Solution in Tamil
x2+y2+2x+4y−20=0
x2+y2+x+2y−10=0
x2+y2−x−2y−20=0
x2+y2−4x−6y−12=0
The correct Answer is:1
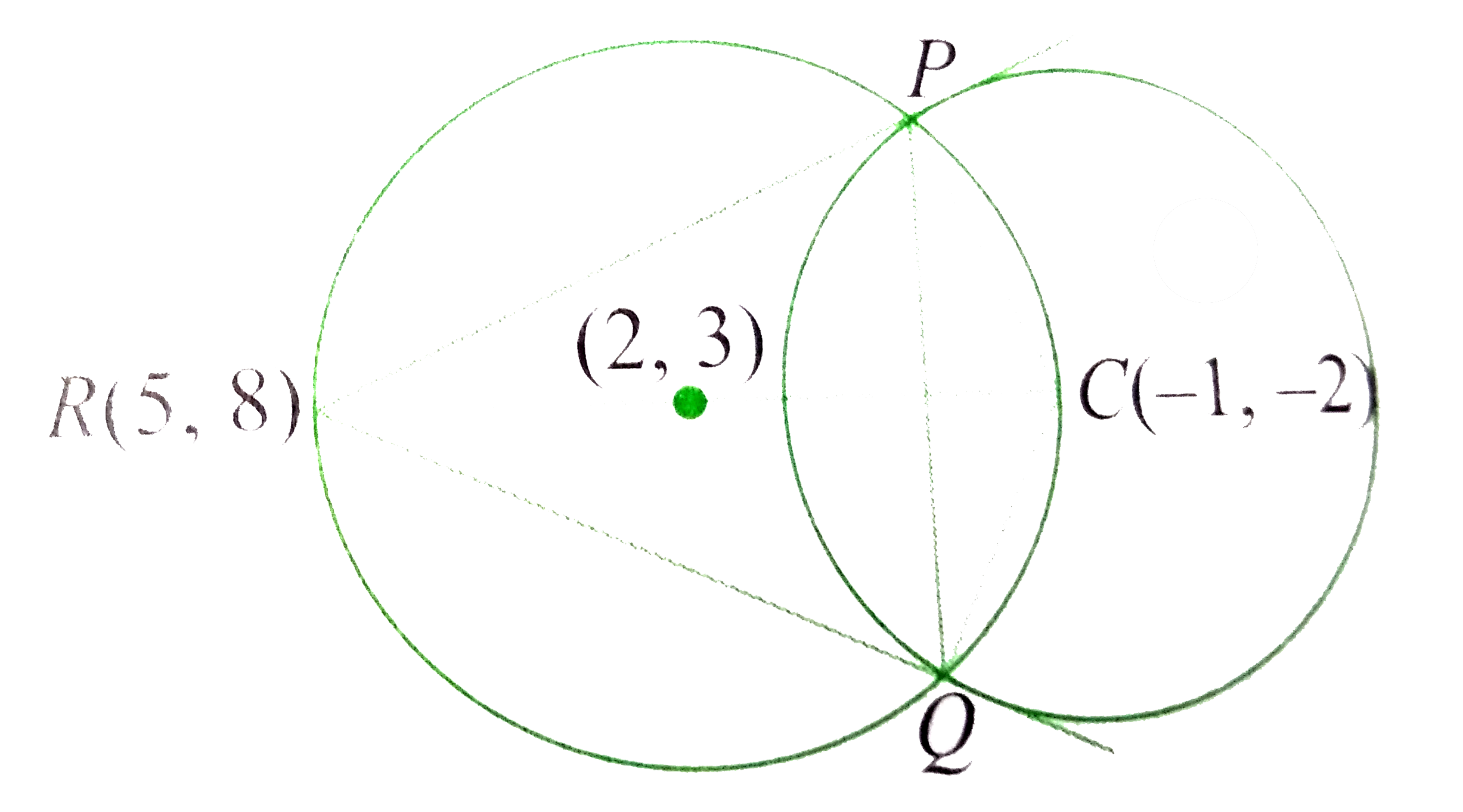
Let C be the center of the given circle.
Then, the circumcircle of ΔRPQ passes through C.
Therefore, (2,3) is the midpoint of RC.
Thus, the coordinates of C are (−1,−2).
Hence, the equation of the circle is x2+y2+2x+4y−20=0
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Multiple)
25 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Comprehension)
46 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.6
5 Videos
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
16 Videos
Similar Questions
about to only mathematics
View SolutionEach side of a square is of length 6 units and the centre of the square Is ( - 1, 2). One of its diagonals is parallel to x+y=0. Find the co-ordinates of the vertices of the square.
View SolutionIf the circle x2+y2+2x+3y+1=0 cuts x2+y2+4x+3y+2=0 at AandB , then find the equation of the circle on AB as diameter.
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionTangents drawn from the point P(1,8) to the circle x^(2)+y^(2)-6x-4y-11=0 touch the circle at the points A and B. The equation of the circumcircle of the triangle PAB is (A) x^(2)+y^(2)+4x-6y+19=0 (B) x^(2)+y^(2)-4x-10y+19=0 (B) x^(2)+y^(2)-2x+6y-29=0 (D) x^(2)+y^(2)-6x-4y+19=0
View SolutionFrom a point R(5,8) , two tangents RPandRQ are drawn to a given circle S=0 whose radius is 5. If the circumcenter of triangle PQR is (2, 3), then the equation of the circle S=0 is x2+y2+2x+4y−20=0 x2+y2+x+2y−10=0 x2+y2−x+2y−20=0 x2+y2+4x−6y−12=0
View SolutionFrom point P on the circle x^(2)-y^(2)-2x+6y-8=0 .tangents PA and PB are drawn to the circle x^(2)+y^(2)-2x+6y+1=0 ,then the locus of orthocentre of Delta PAB is x^(2)+y^(2)+ax+by+c=0, where (a+b-c) is equal to -
View SolutionThe line x+2y+3=0 cuts the circle x^(2)+y^(2)+4x+4y=1 at P and Q, and the line 2x+3y+lambda=0 cuts the circle x^(2)+y^(2)+6x+2y=7 at R and S. If P,Q,R and S are concyclic then lambda is equal to
View SolutionThe radical center of the circles x2+y2−4x−6y+5=0,x2+y2−2x−4y−1=0,x2+y2−6x−2y=0.
View SolutionThe radical centre of the circles x2+y2−4x−6y+5=0,
x2+y2−2x−4y−1=0 and x2+y2−6x−2y=0=0 lies on the line
View SolutionThe circles x2+y2−2x−4y−20=0,x2+y2+4x−2y+4=0 are
View SolutionFind the radical centre of the following circles
x2+y2−4x−6y+5=0
x2+y2−2x−4y−1=0
x2+y2−6x−2y=0
View SolutionThe common tangent at the point of contact of the two circles x2+y2−4x−4y=0,x2+y2+2x+2y=0 is
View SolutionThe circles x2+y2−2x−4y−20=0,x2+y2+4x−2y+4=0 are
View SolutionLet P be any moving point on the circle x2+y2−2x=1.AB be the chord of contact of this point w.r.t. the circle x2+y2−2x=0 . The locus of the circumcenter of triangle CAB(C being the center of the circle) is a.2x2+2y2−4x+1=0 b.x2+y2−4x+2=0 c.x2+y2−4x+1=0 d.2x2+2y2−4x+3=0
View Solution
