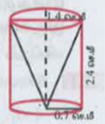
From a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2.Solution in Gujrati
Step by step video & image solution for From a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm^(2). by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - QUESTION PAPER -1EXERCISE - SECTION D ( Answer the following as required with calculations)1videos
QUESTION PAPER -1
- BOOK - KUMAR PRAKASHANCHAPTER - QUESTION PAPER -1EXERCISE - SECTION B( Answer the following briefly with calculations )14videos
QUESTION PAPER -1
- BOOK - KUMAR PRAKASHANCHAPTER - QUADRATIC EQUATIONSEXERCISE - Test Your Skills19videos
QUADRATIC EQUATIONS
- BOOK - KUMAR PRAKASHANCHAPTER - QUESTION PAPER-2EXERCISE - Section C14videos
QUESTION PAPER-2
Similar Questions
Draw a line segment of length 6.5 cm and divide it in the ratio 3:4. Measure the two parts and write the steps of construction.
07:13View SolutionProve that 3+√2 is an irrational number.
06:13View SolutionCheck whether the following are quadratic equations :
(1) (x−3)2=x(2x−5)
(2) (2x−3)(8x+1)=(4x+5)(4x−5)
(3) (5x+3)(x−2)=(4x+3)(2x−1)
(4) (2x+5)3=8(x−1)3
(5) x2+7x−8=x(x+5)
(6) x3+9x2−7x+2=(x+3)3View SolutionOn dividing x3−3x2+x+2 by a polynomial g(x)=x2−x+1, then quotient and remainder.
08:17View SolutionFrom a solid cylinder of height 2.8 cm and diameter 4.2 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid. (Take π=22/7 )
07:22View SolutionFrom a solid cylinder of height 36 cm and radius 14 cm a conical cavity of radus 7 cm and height 24 cm is drilled out . Find the volume and the total surface area of the remaining solid.
06:10View SolutionFrom a circular cylinder of diameter 10 cm and height 12 cm a conical cavity of the same base radius and of the same height in hollowed out. Find the volume and the whole surfce of the remianing solid .Leave the answer in π.
04:43View SolutionFrom a solid cylinder whose height is 15 cm and diameter 16 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid. [Useπ=3.14.]
03:12View Solutionऊँचाई 2⋅4 सेमी और व्यास 1⋅4 सेमी वाले एक ठोस बेलन में से इसी ऊँचाई और इसी व्यास वाला एक शंक्वाकार खोल काट लिया जाता है | शेष बचे ठोस का निकटतम वर्ग सेन्टीमीटर तक पृष्ठीय क्षेत्रफल ज्ञात कीजिए |
05:19View Solutionएक ठोस बेलन, जिसकी ऊँचाई 2.4 सेमी तथा व्यास 1.4 सेमी है, में से उसी ऊँचाई तथा व्यास वाला एक शंकु खोदकर निकाल दिया गया है। शेष बचे ठोस का, निकटतम वर्ग सेमी तक कुल पृष्ठाय क्षेत्रफल ज्ञात कीजिए। [π=227का प्रयोग करें]
05:09View Solutionऊचाई 12 cm और व्यास 10 cm वाले एक ठोस बेलन में से इसी ऊँचाई एवं व्यास बाला एक शंक्वाकार खोल (cavity) काट लिया जाता है । शेष बचे भाग का आयतन ज्ञात करें।
05:30View Solutionएक 24 सेमी. ऊँचाई तथा 1.4 सेमी, व्यास वाले ठोस बेलन में से समान ऊँचाई और समान व्यास वाला एक शंकु के कार का खोल (cavity) काट लिया जाता है। शेष बचे हुए ठोस का पृष्टीय क्षेत्रफल निकटतम वर्ग सेमी, तक ज्ञात करें।
03:35View SolutionFrom a solid cylinder whose height is 2.4 cm and the diameter 1.4 cm, a cone of the same height and same diameter is carved out. Find the volume of the remaining solid to the nearest cm3.
08:15View Solutionஉயரம் 2.4செ.மீ மற்றும் விட்டம் 1.4செ.மீ கொண்ட ஒரு திண்ம உருளையில் இருந்து அதேவிட்டமும் உயரமும் உள்ள ஒரு கூம்பு வெட்டி எடுக்கப்பட்டால் மீதமுள்ள திண்மத்தின் கனஅளவு எவ்வளவு கன செ.மீ ஆகும்? .
 07:17View Solution
07:17View SolutionThe height of a solid cylinder is 2.4 cm and the diameter is 1.4 cm. This caused the conical pits of the same height and diameter to be drilled and hollowed out. The total surface area of the new cube at a very close price cm^(2) Find out at
04:03View SolutionFrom a solid cylinder of height 2.8 cm and diameter 4.2 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid. (Take π=22/7 )
06:46View Solution

